A stream of electrons from a heated filament was passed between two charged plates kept at a potential difference V esu. If c and m are charge and mass of an electron repectively, then the value of h/λ (where λ is wavelength associated with electron wave) is given by :
2meV
√meV
√2meV
meV
The correct Answer is:C
To solve the problem, we need to find the value of hλ, where λ is the wavelength associated with the electron wave. We will use the relationship between kinetic energy, potential difference, and the de Broglie wavelength.
1. Understand the Kinetic Energy of the Electron:
The kinetic energy (KE) of an electron accelerated through a potential difference V is given by:
KE=eV
where e is the charge of the electron.
2. Relate Kinetic Energy to Velocity:
The kinetic energy can also be expressed in terms of the mass m of the electron and its velocity u:
KE=12mu2
Setting the two expressions for kinetic energy equal gives:
eV=12mu2
3. Solve for Velocity:
Rearranging the equation to solve for u2:
mu2=2eV
u2=2eVm
Taking the square root to find u:
u=√2eVm
4. Use the de Broglie Wavelength Formula:
The de Broglie wavelength λ of a particle is given by:
λ=hmv
Substituting u for v:
λ=hmu
5. Substitute for Velocity:
Substitute u=√2eVm into the de Broglie wavelength equation:
λ=hm√2eVm
Simplifying this gives:
λ=h√2emV
6. Find hλ:
Taking the reciprocal to find hλ:
hλ=√2emV
Final Result
Thus, the value of hλ is:
hλ=√2emV
The kinetic energy (KE) of an electron accelerated through a potential difference V is given by:
KE=eV
where e is the charge of the electron.
The kinetic energy can also be expressed in terms of the mass m of the electron and its velocity u:
KE=12mu2
Setting the two expressions for kinetic energy equal gives:
eV=12mu2
Rearranging the equation to solve for u2:
mu2=2eV
u2=2eVm
Taking the square root to find u:
u=√2eVm
The de Broglie wavelength λ of a particle is given by:
λ=hmv
Substituting u for v:
λ=hmu
Substitute u=√2eVm into the de Broglie wavelength equation:
λ=hm√2eVm
Simplifying this gives:
λ=h√2emV
Taking the reciprocal to find hλ:
hλ=√2emV
Thus, the value of hλ is:
hλ=√2emV
Topper's Solved these Questions
Similar Questions
An electron of mass m and charge e is accelerated from rest through a potential difference V in vacuum. The final speed of the electron will be
View SolutionAn electron of mass m and charge e is accelerated from rest through a potential difference V in vacuum. The final speed of the electron will be
View SolutionThe mass of an electron is m, charge is e and it is accelerated form rest through a potential difference of V volts. The velocity acquired by electron will be :
View SolutionA chemist was performing an experiment to study the effect of varying voltage and de-Broglie wavelength of the electron. In first experiment, the electron was accelerated through a potential difference of 1 kV from rest and in second experiment, it was accelerated through a potential difference of 2 kV from rest. The wavelength of de-Broglie waves associated with electron is given by
λ=h√2qVm
Where, V is the voltage through which one electron is accelerated
Putting the values of h, m and q, we get
λ=12.3√VÅ
Answer the following question
In order of get half velocity of electron than in second case , the applied potential difference from rest will be :
View SolutionA chemist was performing an experiment to study the effect of varying voltage and de-Broglie wavelength of the electron. In first experiment, the electron was accelerated through a potential difference of 1 kV from rest and in second experiment, it was accelerated through a potential difference of 2 kV from rest.
The wavelength of de-Broglie waves associated with electron is given by
λ=h√2qVm
Where, V is the voltage through which one electron is accelerated
Putting the values of h, m and q, we get
λ=12.3√VÅ
Answer the following question
The wavelength of electron will be :
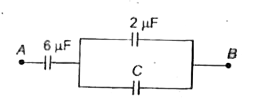
View SolutionIn the given circuit , the potential difference between A and B is 18 V and charge on 2 μF capacitor is 24 μ C . The value of C is
View SolutionIn an electron gun, the electrons are accelerated by the potential V. If the e is the charge and m is the mass of the electron, then the maximum velocity of these electrons will be
View SolutionThe mass of an electron is m, its charge is e and it is accelerated from rest through a potential difference, V. The velocity of electron can be calculated by formula:
View SolutionAn electron of mass m has de Broglie wavelength λ when accelerated through a potential difference V . When a proton of mass M is accelerated through a potential difference 9V, the de Broglie wavelength associated with it will be (Assume that wavelength is determined at low voltage ) .
View SolutionElectron are accelerated through a potential difference V and protons are accelerated through a potential difference of 4 V. The de-Broglie wavelength are λeandλp for electrons and protons, respectively The ratio of λeλp is given by ( given , me is mass of electron and mp is mass of proton )
View Solution
