Question

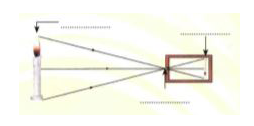
The box of a pin hole camera, of length L, has a hole of radius a . It is assumed that when the hole is illuminated by a parallel beam of light of wavelength $\lambda$ the spread of the spot (obtained on the opposite wall of the camera) is the sum of its geometrical spread and the spread due to diffraction. The spot would then have its minimum size (say b_(min)) when:

Options

A. `a = sqrt(lambda L) and b_(min)=((2lambda^(2))/(L))`
B. `a = sqrt(lambda L) and b_(min)=sqrt(4lambdaL)`
C. `a=(lambda^(2))/(L)and b_(min)=sqrt(4 lambda L)`
D. `a = (lambda^(2))/( L) and b_(min)=((2lambda^(2))/(L))`

Answer

B

Explanation

To solve the problem of finding the minimum size of the spot (b_min) in a pinhole camera, we will follow these steps:

Step 1: Understand the Geometry of the Pinhole Camera
In a pinhole camera, light passes through a small hole and projects an image on the opposite wall. The hole has a radius 'a', and the length of the camera is 'L'. When illuminated by a parallel beam of light of wavelength λ, the light spreads due to both geometrical effects and diffraction.

Step 2: Calculate the Geometrical Spread
The geometrical spread of light due to the radius of the hole can be considered as the diameter of the hole, which is:
\( \text{Geometrical Spread} = 2a \)

Step 3: Calculate the Diffraction Spread
The diffraction spread can be calculated using the formula for the angular width of the first minimum in the diffraction pattern:
\( \theta \approx \frac{\lambda}{a} \)
The linear width of the spot on the opposite wall due to diffraction is given by:
\( \text{Diffraction Spread} = L \cdot \theta = L \cdot \frac{\lambda}{a} \)

Step 4: Total Spread of the Spot
The total spread of the spot (b) on the opposite wall is the sum of the geometrical spread and the diffraction spread:
\( b = 2a + 2 \cdot \frac{L \lambda}{a} \)
This can be simplified to:
\( b = 2a + \frac{2L\lambda}{a} \)

Step 5: Minimize the Size of the Spot
To find the minimum size of the spot, we differentiate b with respect to 'a' and set the derivative to zero:
\( \frac{db}{da} = 2 - \frac{2L\lambda}{a^2} = 0 \)

Step 6: Solve for 'a'
Setting the derivative to zero gives:
\( 2 - \frac{2L\lambda}{a^2} = 0 \)
Rearranging this gives:
\( \frac{2L\lambda}{a^2} = 2 \)
\( a^2 = L\lambda \)
Taking the square root:
\( a = \sqrt{L\lambda} \)

Step 7: Substitute 'a' Back to Find b_min
Now substitute the value of 'a' back into the equation for b:
\( b = 2\sqrt{L\lambda} + \frac{2L\lambda}{\sqrt{L\lambda}} \)
This simplifies to:
\( b = 2\sqrt{L\lambda} + 2\sqrt{L\lambda} = 4\sqrt{L\lambda} \)

Step 8: Final Result for b_min
Since we are looking for the minimum size of the spot (b_min), we take half of the total diameter:
\( b_{\text{min}} = 2\sqrt{L\lambda} \)

Thus, the minimum size of the spot is:
\( b_{\text{min}} = 2\sqrt{L\lambda} \)