If the series limit frequency of the Lyman series is vL, then the series limit frequency of the Pfund seriesis :
vL/25
25vL
16vL
vL/16
The correct Answer is:A
To find the series limit frequency of the Pfund series given the series limit frequency of the Lyman series, we can follow these steps:
1. Understanding the Series Limit Frequencies:
The series limit frequency for a hydrogen atom transition can be calculated using the formula:
ν∝1n2f−n2i
where nf is the final energy level and ni is the initial energy level.
2. Identify the Values for Lyman Series:
For the Lyman series, the transitions end at nf=1 (ground state). The initial levels can be any ni≥2. The series limit corresponds to the transition from ni→∞:
νL∝112−∞2=11−∞→(approaches zero)
3. Identify the Values for Pfund Series:
For the Pfund series, the transitions end at nf=5. The initial levels can be any ni≥6. The series limit corresponds to the transition from ni→∞:
νP∝152−∞2=125−∞→(approaches zero)
4. Setting Up the Ratio:
To find the relationship between the frequencies of the two series:
νPνL=1/(52−∞2)1/(12−∞2)
Simplifying this gives:
νPνL=1/(25−∞)1/(1−∞)=1/251=125
5. Finding the Series Limit Frequency of Pfund:
If we denote the series limit frequency of the Lyman series as νL, then:
νP=νL⋅125
This means:
νP=νL25
Final Answer:
Thus, the series limit frequency of the Pfund series is:
νP=νL25
The series limit frequency for a hydrogen atom transition can be calculated using the formula:
ν∝1n2f−n2i
where nf is the final energy level and ni is the initial energy level.
For the Lyman series, the transitions end at nf=1 (ground state). The initial levels can be any ni≥2. The series limit corresponds to the transition from ni→∞:
νL∝112−∞2=11−∞→(approaches zero)
For the Pfund series, the transitions end at nf=5. The initial levels can be any ni≥6. The series limit corresponds to the transition from ni→∞:
νP∝152−∞2=125−∞→(approaches zero)
To find the relationship between the frequencies of the two series:
νPνL=1/(52−∞2)1/(12−∞2)
Simplifying this gives:
νPνL=1/(25−∞)1/(1−∞)=1/251=125
If we denote the series limit frequency of the Lyman series as νL, then:
νP=νL⋅125
This means:
νP=νL25
Thus, the series limit frequency of the Pfund series is:
νP=νL25
Topper's Solved these Questions
Similar Questions
Knowledge Check
If v1 is the frequency of the series limit of lyman seies, v2 is the freqency of the first line of lyman series and v3 is the fequecny of the series limit of the balmer series, then
Av1−v2=v3Bv1=v2−v3C1v2=1v1+1v3D1v1=1v2+1v3Let v1 be the frequency of series limit of Lyman series, v2 the frequency of the first line of Lyman series and v3 the frequency of series limit of Balmer series. Then which of the following is correct ?
Av1−v2=v3Bv2−v1=v3Cv3=12(v1+v2)Dv1+v2=v3
The relation between λ1= wavelength of series limit of Lyman series ,λ2=the wavelength of the series limit of Balmer sereis & λ3= the wavelength of first line of Lyman series:
View SolutionIf the series limit of Lyman series for Hydrogen atom is equal to the series limit Balmer series for a hydorgen like atom, then atomic number of this hydrogen-like atom will be
View SolutionThe frequency of the first line in Lyman series in the hydrogen spectrum is v. What is the frequency of the corresponding line in the spectrum of doubly ionized Lithium?
View SolutionThe frequency of the first line in Lyman series in the hydrogen spectrum is v. What is the frequency of the corresponding line in the spectrum of doubly ionized Lithium?
View SolutionThe differece is the frequency of series limit of lyman series and balmar series is equal to the frequency of the first line of the lyman series Explain
View SolutionThe mode of the following series is 36. Find the missing frequency in it :
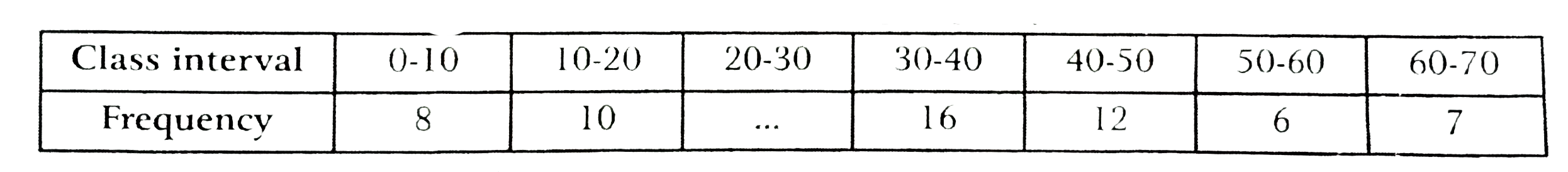
View Solution
