Question

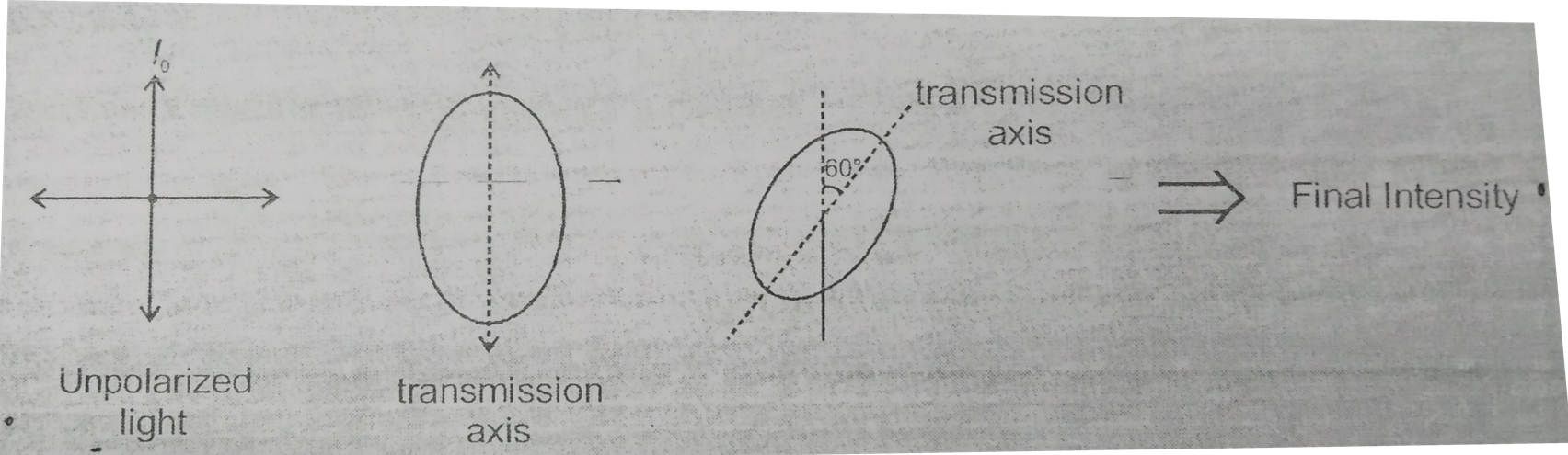
Unpolarized light of intensity I is incident on a system of two polarizers, A followed by B. The intensity of emergent light is $\frac{I}{2}$. If a third polarizer C is placed between A and B, the intensity of emergent light is reduced to $\frac{I}{3}$. The angle between the polarizers A and C is $\theta$. Then :

Options

A. `costheta=((1)/(3))^(1//4)`
B. `costheta=((1)/(3))^(1//2)`
C. `costheta=((2)/(3))^(1//2)`
D. `costheta=((2)/(3))^(1//4)`

Answer

D

Explanation

To solve the problem, we will analyze the situation step by step using the principles of polarization and the Malus's Law.

Step 1: Understanding the Initial Setup
We have unpolarized light of intensity \( I \) incident on two polarizers, A and B. The intensity of light after passing through both polarizers is given as \( \frac{I}{2} \).

Step 2: Intensity After First Polarizer
When unpolarized light passes through the first polarizer (A), the intensity of the emergent light is given by:
\( I_A = \frac{I}{2} \)
This is because unpolarized light passing through a polarizer reduces its intensity to half.

Step 3: Intensity After Second Polarizer
Since the intensity after the first polarizer is \( \frac{I}{2} \) and the light passes through the second polarizer (B), we can assume that the angle between A and B is \( 0^\circ \) (they are aligned). Therefore, the intensity after the second polarizer is:
\( I_B = I_A \cdot \cos^2(0^\circ) = \frac{I}{2} \cdot 1 = \frac{I}{2} \)

Step 4: Introducing the Third Polarizer
Now, we introduce a third polarizer (C) between A and B. Let the angle between polarizer A and C be \( \theta \). The intensity after passing through polarizer C can be calculated using Malus's Law.

Step 5: Intensity After Polarizer C
The intensity after passing through polarizer C is given by:
\( I_C = I_A \cdot \cos^2(\theta) = \frac{I}{2} \cdot \cos^2(\theta) \)

Step 6: Intensity After Second Polarizer (B)
Now, the light passing through polarizer C will then pass through polarizer B. The angle between polarizer C and B is also \( \theta \) (since A and B are parallel). Thus, the intensity after passing through B is:
\( I_B = I_C \cdot \cos^2(\theta) = \left(\frac{I}{2} \cdot \cos^2(\theta)\right) \cdot \cos^2(\theta) = \frac{I}{2} \cdot \cos^4(\theta) \)

Step 7: Setting Up the Equation
According to the problem, the intensity after passing through the two polarizers (A and B with C in between) is given as \( \frac{I}{3} \). Therefore, we can set up the equation:
\( \frac{I}{2} \cdot \cos^4(\theta) = \frac{I}{3} \)

Step 8: Solving for \( \cos^4(\theta) \)
Dividing both sides by \( I \) (assuming \( I \neq 0 \)):
\( \frac{1}{2} \cdot \cos^4(\theta) = \frac{1}{3} \)
Multiplying both sides by 2:
\( \cos^4(\theta) = \frac{2}{3} \)

Step 9: Finding \( \theta \)
Taking the fourth root of both sides:
\( \cos(\theta) = \left(\frac{2}{3}\right)^{1/4} \)

Step 10: Conclusion
Thus, the angle \( \theta \) can be found using:
\( \theta = \cos^{-1}\left(\left(\frac{2}{3}\right)^{1/4}\right) \)