An insulated container of gas has two chambers separated by an insulating partition. One of the chambers has volume V1 and contains ideal gas at pressure P1 and temperature T1.The other chamber has volume V2 and contains ideal gas at pressure P2 and temperature T2. If the partition is removed without doing any work on the gas, the final equilibrium temperature of the gas in the container will be
T1T2(P1V1+P2V2)P1V1T2+P2V2T1
P1V1T1+P2V2T2P1V1+P2V2
P1V1T2+P2V2T1P1V1+P2V2
T1T2(P1V1+P2V2)P1V1T1+P2V2T2
The correct Answer is:A
To find the final equilibrium temperature T of the gases in the insulated container after the partition is removed, we can follow these steps:
1. Understanding the System:
- We have two chambers in an insulated container.
- Chamber 1 has volume V1, pressure P1, and temperature T1.
- Chamber 2 has volume V2, pressure P2, and temperature T2.
- The partition is insulating, meaning no heat transfer occurs between the two chambers.
2. Applying the First Law of Thermodynamics:
- The first law states that the change in internal energy ΔU is equal to the heat added to the system ΔQ minus the work done by the system ΔW:
ΔU=ΔQ−ΔW
- Since the system is insulated, ΔQ=0, and if no work is done on the gases, ΔW=0. Therefore, we have:
ΔU=0
3. Setting Up the Energy Balance:
- The total internal energy before mixing must equal the total internal energy after mixing:
n1CvT1+n2CvT2=(n1+n2)CvT
- Here, n1 and n2 are the number of moles of gas in chambers 1 and 2, respectively, and Cv is the specific heat at constant volume.
4. Simplifying the Equation:
- Since Cv is common to both sides, it can be canceled out:
n1T1+n2T2=(n1+n2)T
5. Solving for Final Temperature T:
- Rearranging gives:
T=n1T1+n2T2n1+n2
6. Finding the Number of Moles:
- Using the ideal gas law PV=nRT, we can express n1 and n2:
n1=P1V1RT1,n2=P2V2RT2
7. Substituting into the Temperature Equation:
- Substitute n1 and n2 into the equation for T:
T=(P1V1RT1)T1+(P2V2RT2)T2P1V1RT1+P2V2RT2
8. Simplifying the Expression:
- This simplifies to:
T=P1V1T2+P2V2T1P1V1+P2V2
Final Result:
The final equilibrium temperature T of the gases in the container is given by:
T=P1V1T2+P2V2T1P1V1+P2V2
- We have two chambers in an insulated container.
- Chamber 1 has volume V1, pressure P1, and temperature T1.
- Chamber 2 has volume V2, pressure P2, and temperature T2.
- The partition is insulating, meaning no heat transfer occurs between the two chambers.
- The first law states that the change in internal energy ΔU is equal to the heat added to the system ΔQ minus the work done by the system ΔW:
ΔU=ΔQ−ΔW
- Since the system is insulated, ΔQ=0, and if no work is done on the gases, ΔW=0. Therefore, we have:
ΔU=0
- The total internal energy before mixing must equal the total internal energy after mixing:
n1CvT1+n2CvT2=(n1+n2)CvT
- Here, n1 and n2 are the number of moles of gas in chambers 1 and 2, respectively, and Cv is the specific heat at constant volume.
- Since Cv is common to both sides, it can be canceled out:
n1T1+n2T2=(n1+n2)T
- Rearranging gives:
T=n1T1+n2T2n1+n2
- Using the ideal gas law PV=nRT, we can express n1 and n2:
n1=P1V1RT1,n2=P2V2RT2
- Substitute n1 and n2 into the equation for T:
T=(P1V1RT1)T1+(P2V2RT2)T2P1V1RT1+P2V2RT2
- This simplifies to:
T=P1V1T2+P2V2T1P1V1+P2V2
The final equilibrium temperature T of the gases in the container is given by:
T=P1V1T2+P2V2T1P1V1+P2V2
Topper's Solved these Questions
Similar Questions
An ideal gas has pressure P, volume V and temperature T. Select the correct option.
View SolutionTwo adiabatic containers have volumes V1 and V2 respectively. The first container has monoatomic gas at pressure p1 and temperature T1. The second container has another monoatomic gas at pressure p2 and temperature T2. When the two containers are connected by a narrow tube, the final temperature and pressure of the gases in the containers are P and T respectively. Then
View SolutionA uniform tube of volume V contains an ideal monatomic gas at a uniform pressure P the temperature of the gas varies linearly along te length of the tube ad T and 2 T are the temperature at the ends.
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View SolutionA container with insulating walls is divided into two equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated . If the valve is suddenly opened, the pressure and temperature of the gas will be
View SolutionFigure shows volume - temperature (V-T) graph for same mass of an ideal gas at two different pressures P1and P2. Hence
View SolutionSome ideal monoatomic gas A in an enclosure has a pressure P and the temperature T . Another ideal monoatomic gas B enclosed in a container of the same volume has a pressure of 2P and temperature T2. The ratio of the average kinetic energy per molecule of gas A to gas B is
View SolutionFollowing figure shows on adiabatic cylindrical container of volume V0 divided by an adiabatic smooth piston (area of cross-section = A ) in two equal parts. An ideal gas (Cp/Cy=λ) is at pressure P1 and temperature T1 in left part and gas at pressure P2 and temperature T2 in right part. The piston is slowly displaced and released at a position where it can stay in equilibrium. The final pressure of the two parts will be (Suppose x = displacement of the piston)

View SolutionAn inflated rubber ballon contains one mole of an ideal gas , has a pressure P, volume V and temperature T. If the temperature rises to 1.1 T, and the volume isincreased to 1.05 V, the final pressure will be
View SolutionThe container shown in figure has two chambers separated by a partition of volumes V1=2.0L and V2=3.0L. The chambers contain μ14.0 and μ2=5.0 mole of a gas at pressure p1=1.00 atm and P2=2.00 atm. Calculate the pressure after the partition is removed and the mixture attains equilibrium.
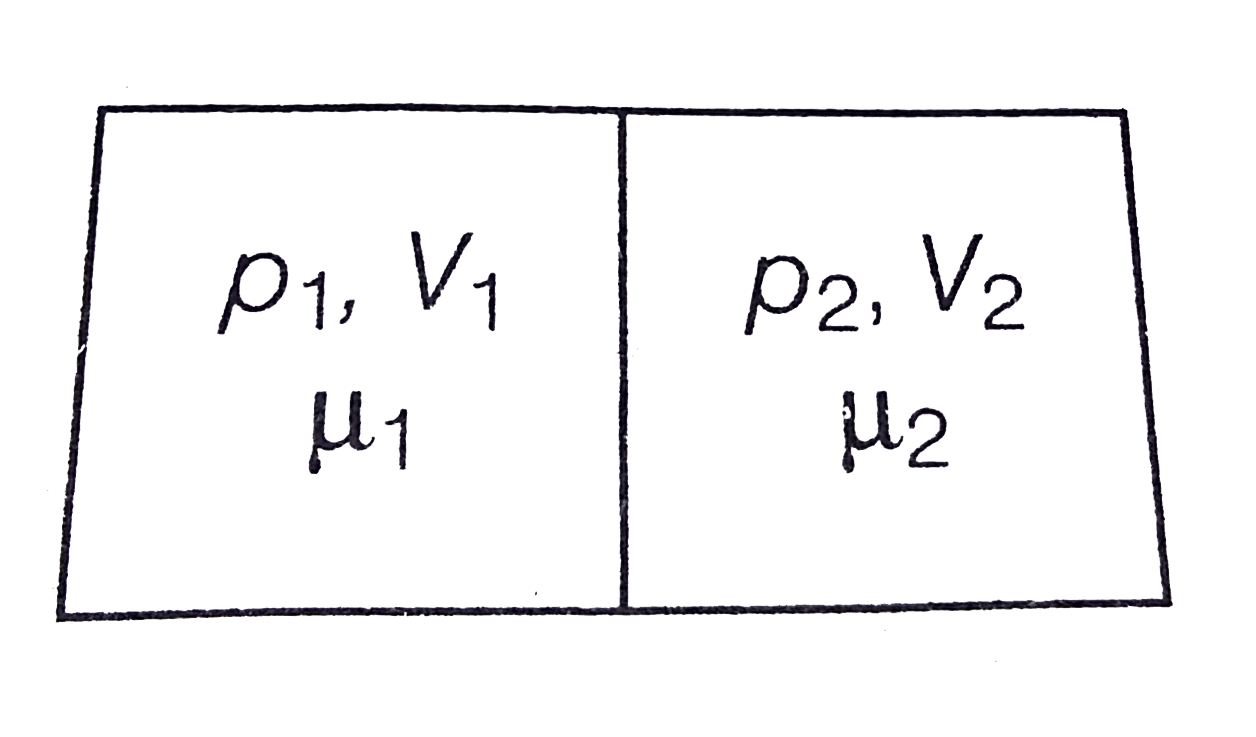
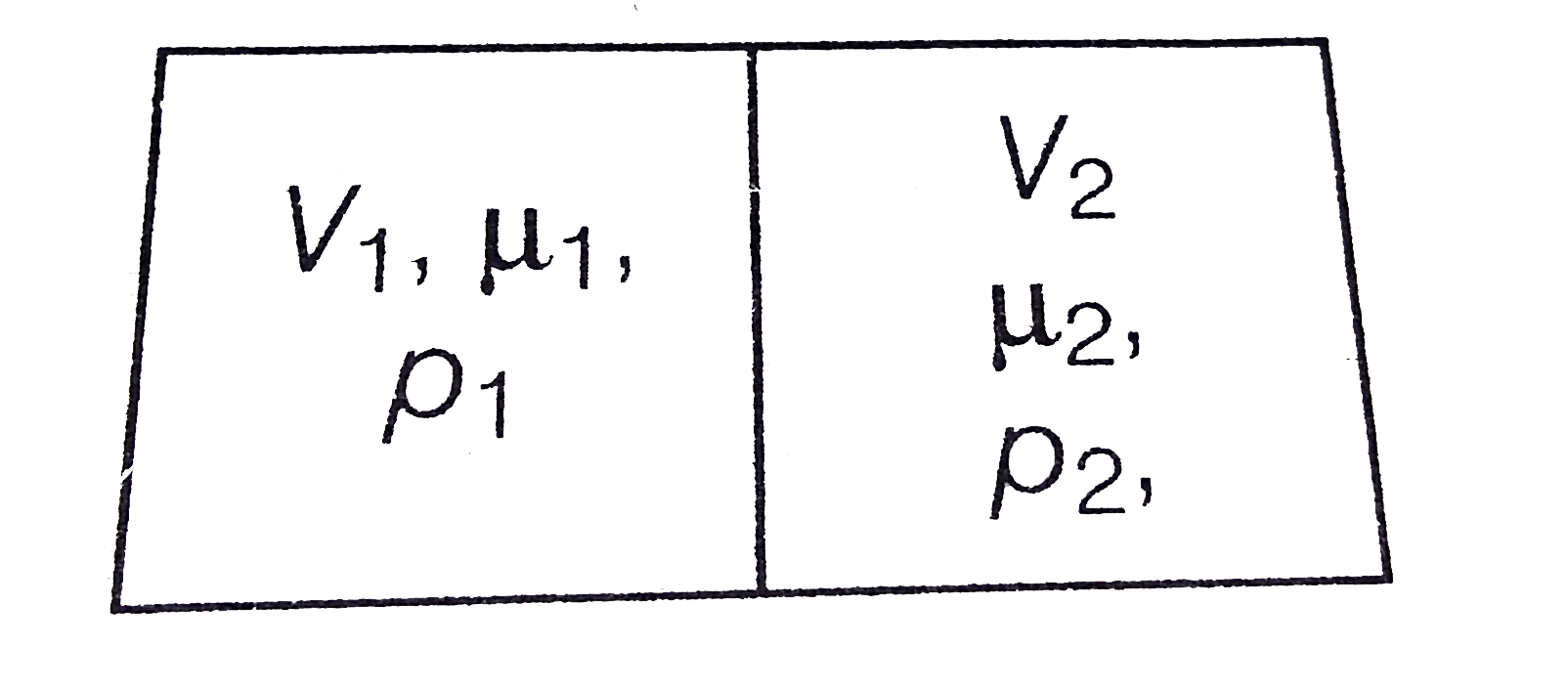
View Solution
