A particle is moving with velocity →v=k(yˆi+xˆj), where k is a constant . The genergal equation for its path is
y=x2+constant
y2=x+constant
xy=constant
y2=x2+constant
The correct Answer is:D
To find the general equation for the path of a particle moving with the given velocity vector →v=k(^y+^x), where k is a constant, we can follow these steps:
Step 1: Identify the components of the velocity vector
The velocity vector can be expressed in terms of its components:
→v=ky^i+kx^j
This means:
- The x-component of the velocity vx=ky
- The y-component of the velocity vy=kx
Step 2: Write the differential forms of the components
From the definitions of velocity, we can write:
vx=dxdt=ky(1)
vy=dydt=kx(2)
Step 3: Form the ratio of the differentials
To eliminate dt, we can form the ratio of the two equations:
dydt÷dxdt=kxky
This simplifies to:
dydx=xy
Step 4: Rearrange the equation
Rearranging gives:
ydy=xdx
Step 5: Integrate both sides
Now, we integrate both sides:
∫ydy=∫xdx
This results in:
y22=x22+C
where C is the constant of integration.
Step 6: Simplify the equation
Multiplying through by 2 to eliminate the fractions gives:
y2=x2+2C
We can denote 2C as a new constant k:
y2=x2+k
Final Result
Thus, the general equation for the path of the particle is:
y2=x2+C
where C is a constant.
---
The velocity vector can be expressed in terms of its components:
→v=ky^i+kx^j
This means:
- The x-component of the velocity vx=ky
- The y-component of the velocity vy=kx
From the definitions of velocity, we can write:
vx=dxdt=ky(1)
vy=dydt=kx(2)
To eliminate dt, we can form the ratio of the two equations:
dydt÷dxdt=kxky
This simplifies to:
dydx=xy
Rearranging gives:
ydy=xdx
Now, we integrate both sides:
∫ydy=∫xdx
This results in:
y22=x22+C
where C is the constant of integration.
Multiplying through by 2 to eliminate the fractions gives:
y2=x2+2C
We can denote 2C as a new constant k:
y2=x2+k
Thus, the general equation for the path of the particle is:
y2=x2+C
where C is a constant.
Topper's Solved these Questions
Similar Questions
A particle is moving in a plane with velocity given by →u=u0ˆi+(aωcosωt)ˆj, where ˆi and ˆj are unit vectors along x and y axes respectively. If particle is at the origin at t=0. Calculate the trajectory of the particle :-
View SolutionAn alpha particle moving with the velocity →v=uˆi+ˆj ,in a uniform magnetic field →B=Bˆk . Magnetic force on alpha particle is
View Solution
Knowledge Check
The value of (ˆk׈j).ˆi+ˆj.ˆk =
A−1B1C0D2
A particle is moving with a constant acceleration →a=ˆi−2ˆjm/s2 and instantaneous velocity →v=ˆi+2ˆj+2ˆkm/s then rate of change of speed of particle 1 second after this instant will be :
View SolutionA plane mirror is moving with velocity 4(ˆi)+4(ˆj)+8(ˆk). A point object in front of the mirror moves with a velocity 3(ˆi)+4(ˆj)+5(ˆk). Here, ˆk is along the normal to the plane mirror and facing towards the object. The velocity of the image is
View SolutionA plane mirror is moving with velocity 4(ˆi)+4(ˆj)+8(ˆk). A point object in front of the mirror moves with a velocity 3(ˆi)+4(ˆj)+5(ˆk). Here, ˆk is along the normal to the plane mirror and facing towards the object. The velocity of the image is
View SolutionA particle is moving with speed 6m/s along the direction of →A=2ˆi+2ˆj−ˆk, then its velocity is :
View SolutionA particle is moving in x-y plane. At an instant, it has velocity (4ˆi+4ˆj)m/s and acceleration (3ˆi+5ˆj)m/s2 At that instant, the radius of curvature of its path will be :
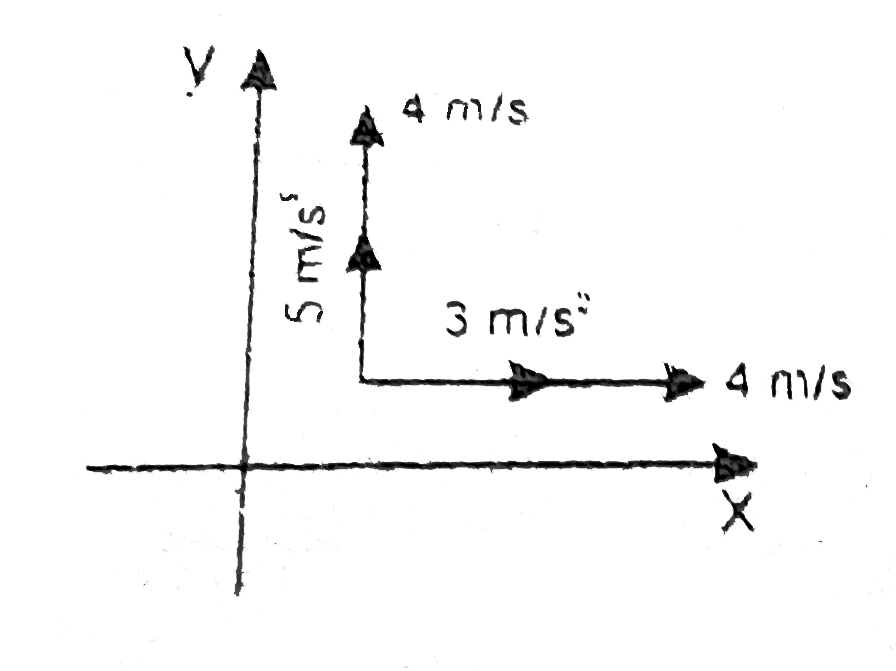
View Solutionˆi+ ˆj + ˆk =
View SolutionFind the kinetic energy of a particle of mass 200g moving with velocity 3ˆi−5ˆj+4ˆkm/s.
View Solution
