A diatomic ideal gas is used in a Carnot engine as the working substance. If during the adiabatic expansion part of the cycle the volume of the gas increase from V to 32V, the efficiency of the engine is
0.5
0.75
0.99
0.25
The correct Answer is:B
To solve the problem, we need to find the efficiency of a Carnot engine using a diatomic ideal gas as the working substance, given that the volume of the gas increases from V to 32V during the adiabatic expansion.
1. Understand the relationship during adiabatic expansion:
The relationship between temperature and volume for an adiabatic process is given by:
TVγ−1=constant
where γ=CpCv. For a diatomic ideal gas, γ=75.
2. Set up the equation for the initial and final states:
Let T1 be the initial temperature and T2 be the final temperature. The initial volume is V1=V and the final volume is V2=32V. Thus, we can write:
T1V75−1=T2(32V)75−1
Simplifying gives:
T1V25=T2(3225V25)
3. Cancel the common terms:
Since V25 appears on both sides, we can cancel it:
T1=T2⋅3225
4. Calculate 3225:
We know that 32=25, so:
3225=(25)25=22=4
Therefore, we have:
T1=4T2
5. Determine the efficiency of the Carnot engine:
The efficiency η of a Carnot engine is given by:
η=1−T2T1
Substituting T1=4T2 into the efficiency formula:
η=1−T24T2=1−14=34
6. Final result:
Therefore, the efficiency of the Carnot engine is:
η=0.75
The relationship between temperature and volume for an adiabatic process is given by:
TVγ−1=constant
where γ=CpCv. For a diatomic ideal gas, γ=75.
Let T1 be the initial temperature and T2 be the final temperature. The initial volume is V1=V and the final volume is V2=32V. Thus, we can write:
T1V75−1=T2(32V)75−1
Simplifying gives:
T1V25=T2(3225V25)
Since V25 appears on both sides, we can cancel it:
T1=T2⋅3225
We know that 32=25, so:
3225=(25)25=22=4
Therefore, we have:
T1=4T2
The efficiency η of a Carnot engine is given by:
η=1−T2T1
Substituting T1=4T2 into the efficiency formula:
η=1−T24T2=1−14=34
Therefore, the efficiency of the Carnot engine is:
η=0.75
Topper's Solved these Questions
Similar Questions
A monoatomic ideal gas is used in a carnot engine as the working substance. If during the adiabatic expansion the volume of the gas increases from V8 to V, the efficiency of the engine is
View SolutionDuring an adiabatic reversibly expansion of an ideal gas
View SolutionA diatomic gas is used in car not heat engine having efficiency 80% . Find the ratio of initial volume to final volume of gas during adiabatic expansion.
View SolutionDuring adiabatic expansion of ideal gas, which is correct ?
View SolutionConsider an ideal gas confined in an isolated closed chamber. As the gas undergoes an adiabatic expansion, the average time of collision between molecules increase as Vq, where V is the volume of the gas. The value of q is : (γ=CpCv)
View SolutionAn engine operates by taking a monatomic ideal gas through the cycle shown in the figure. The percentage efficiency of the engine is close to ____________
 View Solution
View SolutionA cycle followed by an engine (made of one mole of perfect gas in a cylinder with a piston) is shown in figure.
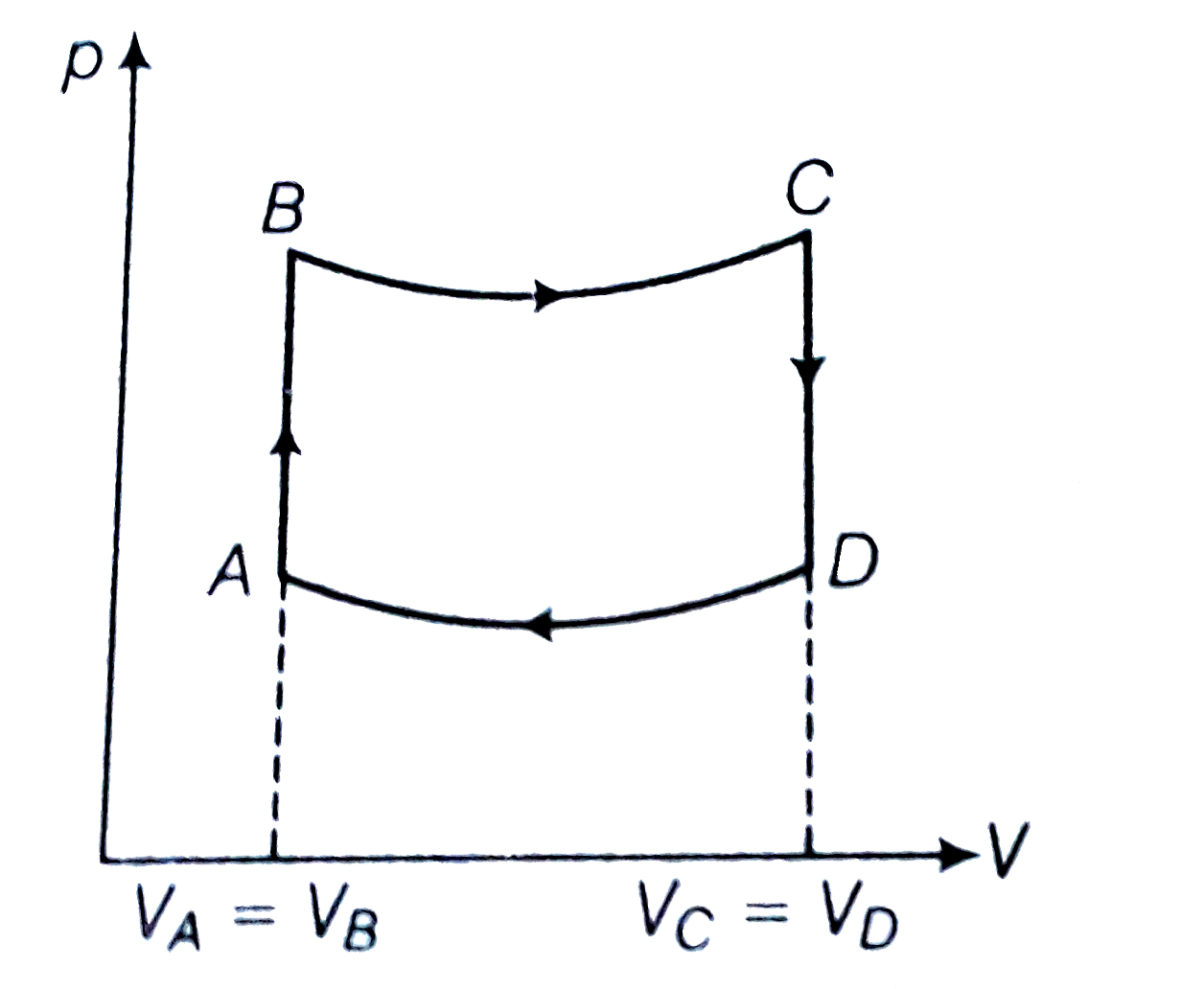
A to B volume constant, B to C adiabatic, C to D volume constant and D to A adiabatic
VC=VD=2VA=2VB
(a) In which part of the cycle heat is supplied to the engine form outside?
(b) In which part of the cycle heat is being given to the surrounding by the engine ?
(c ) What is the work done by the engine in one cycle ? Write your answer in term of pA,pB,VA ?
(d) What is the efficiency of the engine ?
(γ=53 for the gas), (CV=32R for one mole )View SolutionAssertion:- During adiabatic expansion of an ideal gas, temperature falls but entropy remains constant.
Reason:- During adiabatic expansion, work is done by the gas using a part of internal energy and no heat exchange teakes place the system and the surrounding.View SolutionThe temperature of an ideal gas undergoing adiabatic expansion varies with volume as T∝V−34, then the value of CPCV for the gas is
View SolutionAn ideal gas A and a real gas B have their volumes increases from V→2V under isothermal condtitions. The increase in internal energy
View Solution

