Two projectiles are thrown with different velocities and at different angles so as to cover the same maximum height. Show that the sum of the times taken by each to reach the highest point is equal to the total time taken by either of the projectiles.
To solve the problem, we need to show that the sum of the times taken by each projectile to reach the highest point is equal to the total time taken by either of the projectiles. Let's denote the two projectiles as Projectile 1 and Projectile 2.
Step 1: Define Variables
Let:
- u1 = initial velocity of Projectile 1
- θ1 = angle of projection of Projectile 1
- u2 = initial velocity of Projectile 2
- θ2 = angle of projection of Projectile 2
- g = acceleration due to gravity
Step 2: Maximum Height Calculation
The maximum height h reached by a projectile is given by the formula:
h=u2sin2θ2g
For Projectile 1:
h1=u21sin2θ12g
For Projectile 2:
h2=u22sin2θ22g
Given that both projectiles reach the same maximum height, we have:
h1=h2⟹u21sin2θ12g=u22sin2θ22g
This simplifies to:
u21sin2θ1=u22sin2θ2(1)
Step 3: Time to Reach Maximum Height
The total time of flight T for a projectile is given by:
T=2usinθg
Thus, the time taken to reach the maximum height for Projectile 1 is:
T1=2u1sinθ1g
The time taken to reach the maximum height for Projectile 2 is:
T2=2u2sinθ2g
The time to reach the maximum height for each projectile is half of the total time:
t1=T12=u1sinθ1g
t2=T22=u2sinθ2g
Step 4: Sum of Times to Reach Maximum Height
Now, we need to show that:
t1+t2=T
Substituting the expressions for t1 and t2:
t1+t2=u1sinθ1g+u2sinθ2g=u1sinθ1+u2sinθ2g
Step 5: Relate the Sine Terms
From equation (1), we know that:
u1sinθ1=u2sinθ2
Thus, we can replace u2sinθ2 with u1sinθ1:
t1+t2=u1sinθ1+u1sinθ1g=2u1sinθ1g
This is equal to T1 (the total time of flight for Projectile 1):
T1=2u1sinθ1g
Thus, we have:
t1+t2=T1
Conclusion
Therefore, we have shown that the sum of the times taken by each projectile to reach the highest point is equal to the total time taken by either of the projectiles.
Let:
- u1 = initial velocity of Projectile 1
- θ1 = angle of projection of Projectile 1
- u2 = initial velocity of Projectile 2
- θ2 = angle of projection of Projectile 2
- g = acceleration due to gravity
The maximum height h reached by a projectile is given by the formula:
h=u2sin2θ2g
For Projectile 1:
h1=u21sin2θ12g
For Projectile 2:
h2=u22sin2θ22g
Given that both projectiles reach the same maximum height, we have:
h1=h2⟹u21sin2θ12g=u22sin2θ22g
This simplifies to:
u21sin2θ1=u22sin2θ2(1)
The total time of flight T for a projectile is given by:
T=2usinθg
Thus, the time taken to reach the maximum height for Projectile 1 is:
T1=2u1sinθ1g
The time taken to reach the maximum height for Projectile 2 is:
T2=2u2sinθ2g
The time to reach the maximum height for each projectile is half of the total time:
t1=T12=u1sinθ1g
t2=T22=u2sinθ2g
Now, we need to show that:
t1+t2=T
Substituting the expressions for t1 and t2:
t1+t2=u1sinθ1g+u2sinθ2g=u1sinθ1+u2sinθ2g
From equation (1), we know that:
u1sinθ1=u2sinθ2
Thus, we can replace u2sinθ2 with u1sinθ1:
t1+t2=u1sinθ1+u1sinθ1g=2u1sinθ1g
This is equal to T1 (the total time of flight for Projectile 1):
T1=2u1sinθ1g
Thus, we have:
t1+t2=T1
Therefore, we have shown that the sum of the times taken by each projectile to reach the highest point is equal to the total time taken by either of the projectiles.
Topper's Solved these Questions
- Motion in a PlaneBOOK - SL ARORACHAPTER - Motion in a PlaneEXERCISE - Excercise
397 Videos
- Motion in a PlaneBOOK - SL ARORACHAPTER - Motion in a PlaneEXERCISE - Excercise
397 Videos
- MECHANICSBOOK - SL ARORACHAPTER - MECHANICSEXERCISE - Exercise
341 Videos
- MOTION IN ONE DIMENSIONBOOK - SL ARORACHAPTER - MOTION IN ONE DIMENSIONEXERCISE - problems for self practice
66 Videos
Similar Questions
Two projectiles are projected from the same point different velocities and different angles of projection .If the maximum height attained by both the projectiles is same, show that sum of the times taken by each to reach the highest point is equal to the total time of flight of either of the projectiles .
View SolutionProve that time taken by a body to rise to highest point is always equal to the time taken by it to fall through he same height.
View Solution
Knowledge Check
In question number nine the time taken by the bady to reach the highest point is
A1.43 sB4.1 sC1.24 sDnone of theseA projectile is thrown at angle β with vertical.It reaches a maximum height H.The time taken to reach the hightest point of its path is
A√HgB√2HgC√H2gD√2HgcosθIf time taken by the projectile to reach Q is T, than PQ=
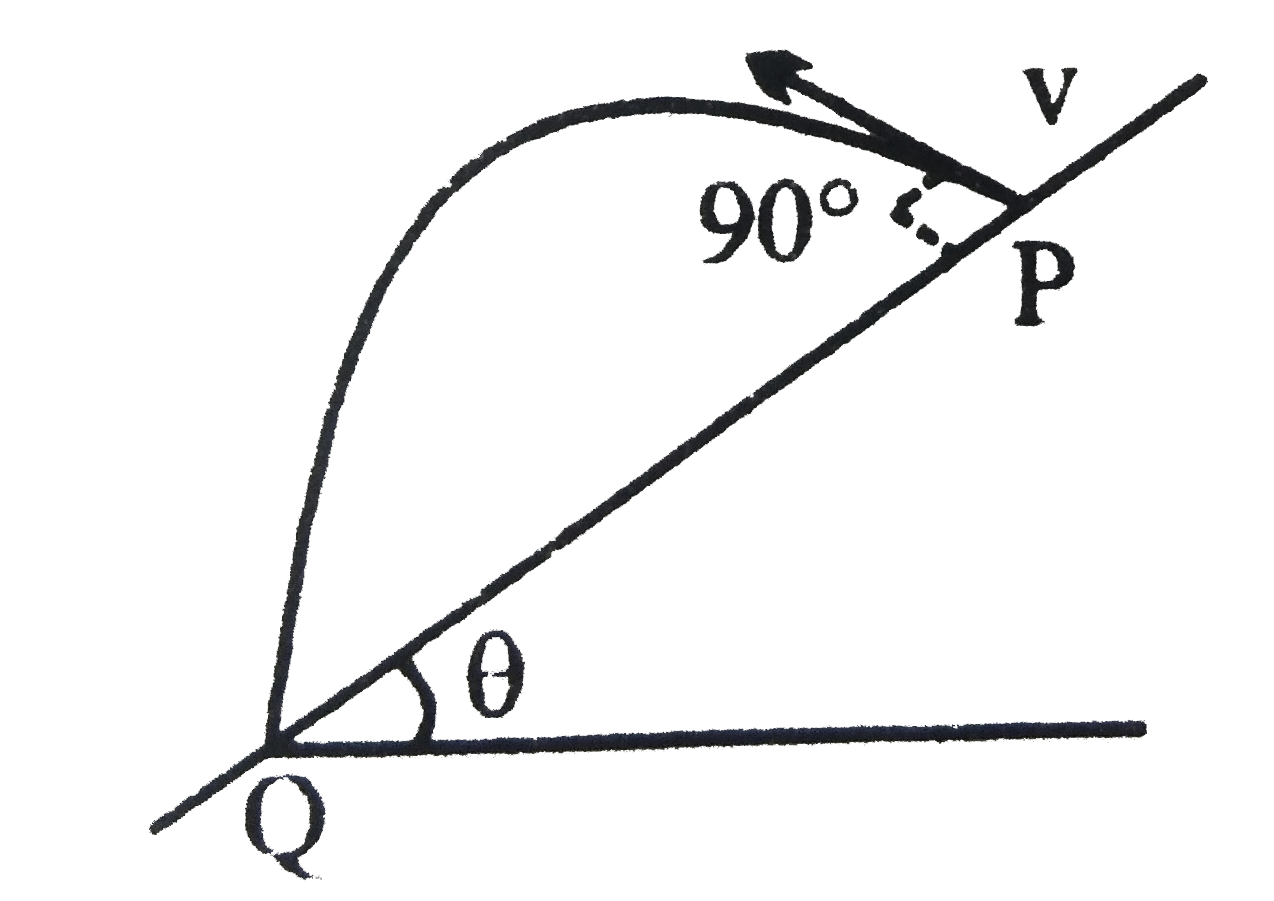 ATvsinθBTvcosθCTvsecθDTvtanθ
ATvsinθBTvcosθCTvsecθDTvtanθ
Show that there are two values of time for which is projectile is at the same height. Also show that the sum of these two times is equal to the time of flight.
View SolutionA projectile thrown with velocity v making angle θ with vertical gains maximum height H in the time for which the projectile remains in air, the time period is
View SolutionA particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View SolutionTwo projectiles are fired at different angles with the same magnitude of velocity such that they have the same range. At what angles they might have been projected ?
View SolutionTwo projectile are projected with the same velocity. If one is projected at an angle of 30∘ to the horizontal. The ratio if maximum heights reached, is:
View Solution