Give the concept of gravitational field.
Step-by-Step Solution:
1. Definition of Gravitational Field:
A gravitational field is a region of space surrounding a mass where other masses experience a force due to gravity. It describes the influence that a mass exerts on other masses in its vicinity.
2. Force Exerted by a Mass:
According to Newton's law of universal gravitation, any mass m1 will exert a gravitational force on another mass m2. This force can be expressed mathematically as:
F=G⋅m1⋅m2r2
where F is the gravitational force, G is the gravitational constant, r is the distance between the centers of the two masses, m1 and m2.
3. Gravitational Field Strength:
The gravitational field strength g at a distance r from a mass m1 can be defined as the force experienced by a unit mass placed at that point. It is given by:
g=Fm2=G⋅m1r2
Here, g is the gravitational field strength, and it is independent of the mass m2.
4. Unit of Gravitational Field:
The unit of gravitational field strength is Newton per kilogram (N/kg). This indicates the force experienced by a mass of one kilogram in the gravitational field.
5. Conceptual Understanding:
The gravitational field can be thought of as a way to visualize how masses influence each other through gravitational forces. It helps in understanding gravitational phenomena without needing to calculate the force between every pair of masses directly.
A gravitational field is a region of space surrounding a mass where other masses experience a force due to gravity. It describes the influence that a mass exerts on other masses in its vicinity.
According to Newton's law of universal gravitation, any mass m1 will exert a gravitational force on another mass m2. This force can be expressed mathematically as:
F=G⋅m1⋅m2r2
where F is the gravitational force, G is the gravitational constant, r is the distance between the centers of the two masses, m1 and m2.
The gravitational field strength g at a distance r from a mass m1 can be defined as the force experienced by a unit mass placed at that point. It is given by:
g=Fm2=G⋅m1r2
Here, g is the gravitational field strength, and it is independent of the mass m2.
The unit of gravitational field strength is Newton per kilogram (N/kg). This indicates the force experienced by a mass of one kilogram in the gravitational field.
The gravitational field can be thought of as a way to visualize how masses influence each other through gravitational forces. It helps in understanding gravitational phenomena without needing to calculate the force between every pair of masses directly.
Topper's Solved these Questions
Similar Questions
Knowledge Check
What is the intensity of gravitational field of the centre of a spherical shell
AGm/r2BgCZeroDNone of theseIf gravitational field due to uniform thin hemispherical shell at point P is I, then the magnitude of gravitational field at Q is (Mass of hemispherical shell is M, radius is R)
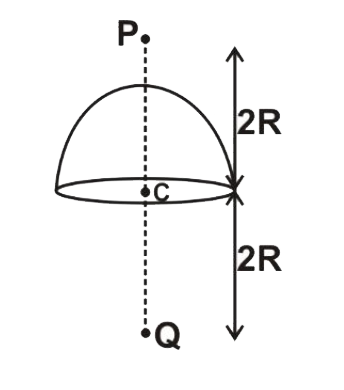 AGM2R2−IBGM2R2+ICGM4R−ID2I−GM2R2
AGM2R2−IBGM2R2+ICGM4R−ID2I−GM2R2The S.I. units of intensity of gravitational field
AJ/kgBJ/kgs2CN/kgDm/s3
Define intensity of gravitational field at any point. Is it a scalar or vector ?
View SolutionWhat is Gravitational field?
View SolutionDefine the term gravitational field.
View SolutionAssertion : Gravitational field is zero both at centre and infinity.
Reason : The dimensions of gravitational field is [LT−2]
View SolutionThe intensity of the gravitational field of the earth is maximum at
View Solution