Explain what happens when the load on a metal wire suspended from a rigid support is gradually increased. Illustrate your answer with a suitable stress-strain graph.
Step-by-Step Solution:
1. Understanding Stress and Strain:
- Stress is defined as the force applied per unit area (σ = F/A), where F is the force and A is the cross-sectional area of the wire.
- Strain is the measure of deformation representing the displacement between particles in a material (ε = ΔL/L₀), where ΔL is the change in length and L₀ is the original length.
2. Initial State:
- When a metal wire is suspended from a rigid support and no load is applied, it remains in its original shape and length.
3. Applying Load:
- As a load is gradually applied to the wire, the wire begins to stretch. This is the elastic region of the material where it will return to its original shape once the load is removed.
4. Elastic Limit:
- As the load continues to increase, the stress in the wire increases linearly with strain. This linear relationship is described by Hooke's Law (σ = Eε), where E is the Young's modulus of the material.
- The point at which the material can no longer return to its original shape is called the elastic limit.
5. Yield Point:
- Beyond the elastic limit, the wire enters the plastic region where permanent deformation occurs. The yield point is the point at which the wire will deform permanently.
6. Ultimate Tensile Strength:
- As the load is further increased, the wire reaches its ultimate tensile strength, which is the maximum stress that the material can withstand while being stretched before necking begins.
7. Necking and Fracture:
- After reaching the ultimate tensile strength, the wire will begin to neck, which is a localized reduction in cross-section. Eventually, the wire will fracture if the load continues to increase.
8. Stress-Strain Graph:
- The stress-strain graph illustrates these stages:
- The initial linear portion represents the elastic region.
- The point where the curve starts to bend indicates the yield point.
- The peak of the curve represents the ultimate tensile strength.
- The downward slope after the peak indicates necking and eventual fracture.
Stress-Strain Graph Illustration:
- X-axis: Strain (ε)
- Y-axis: Stress (σ)
- Regions:
- Elastic Region: Linear increase (Hooke's Law)
- Yield Point: Change from linear to plastic deformation
- Ultimate Tensile Strength: Peak of the graph
- Necking and Fracture: Decrease in stress after the peak
- Stress is defined as the force applied per unit area (σ = F/A), where F is the force and A is the cross-sectional area of the wire.
- Strain is the measure of deformation representing the displacement between particles in a material (ε = ΔL/L₀), where ΔL is the change in length and L₀ is the original length.
- When a metal wire is suspended from a rigid support and no load is applied, it remains in its original shape and length.
- As a load is gradually applied to the wire, the wire begins to stretch. This is the elastic region of the material where it will return to its original shape once the load is removed.
- As the load continues to increase, the stress in the wire increases linearly with strain. This linear relationship is described by Hooke's Law (σ = Eε), where E is the Young's modulus of the material.
- The point at which the material can no longer return to its original shape is called the elastic limit.
- Beyond the elastic limit, the wire enters the plastic region where permanent deformation occurs. The yield point is the point at which the wire will deform permanently.
- As the load is further increased, the wire reaches its ultimate tensile strength, which is the maximum stress that the material can withstand while being stretched before necking begins.
- After reaching the ultimate tensile strength, the wire will begin to neck, which is a localized reduction in cross-section. Eventually, the wire will fracture if the load continues to increase.
- The stress-strain graph illustrates these stages:
- The initial linear portion represents the elastic region.
- The point where the curve starts to bend indicates the yield point.
- The peak of the curve represents the ultimate tensile strength.
- The downward slope after the peak indicates necking and eventual fracture.
- X-axis: Strain (ε)
- Y-axis: Stress (σ)
- Regions:
- Elastic Region: Linear increase (Hooke's Law)
- Yield Point: Change from linear to plastic deformation
- Ultimate Tensile Strength: Peak of the graph
- Necking and Fracture: Decrease in stress after the peak
Topper's Solved these Questions
- Mechanical Properties of SolidsBook:SL ARORAChapter:Mechanical Properties of SolidsExercise:Example
Explore 113 Videos
- Mechanical Properties of fluidsBook:SL ARORAChapter:Mechanical Properties of fluidsExercise:Exercise
Explore 459 Videos
- MECHANICSBook:SL ARORAChapter:MECHANICSExercise:Exercise
Explore 341 Videos
Similar Questions
One end of a uniform rod of mass M and cross-sectional area A is suspended from a rigid support and an equal mass M is suspended from the other end, what is the stress at the mid point of the rod.
View SolutionTwo wires of same length and material but of different radii are suspended from a rigid support. Both carry the same load. Will the stress, strain and extension in them be same or different ?
View Solution
Knowledge Check
Two wires of the same length and material but different radii r1 and r2 are suspended from a rigid support both carry the same load at the lower end. The ratio of the stress devedoped in the second wire to that developed in the first wire is
Ar1r2Br21r22C(r1r2)5/2D(r1r2)1/2A steel wire can withstand a load up to 2940N. A load of 150kg is suspended from a rigid support. The maximum angle through which the wire can be displaced from the mean position, so that the wire dose not break when the load passs through the position of equilibrium, is
A30∘B60∘C80∘D85∘An Aluminum and Copper wire of same cross sectional area but having lengths in the ratio 2:3 are joined end to end. This composite wire is hung form a rigid support and a load is suspended form the free end. If the increases in length of the composite wire is 2.1mm the increses in lenghts of Aluminimum and Copper wirs are
A0.7mm,1.4mmB0.9mm,1.2mmC1.0mm,1.1mmD1.6mm,1.5mm
When a load of 10 kg is suspended on a metallic wire, its length increase by 2 mm. The force constant of the wire is
View SolutionWhat happens when chromatids fail to segregate during cell division cycle ?
Explain your answer with an example.
View SolutionWhat happens to the eye when you enter a darkened cinema hall from bright sunshine ? Give reason for your answer.
View SolutionA unifrom rod is fixed at one end to a rigid support, its temperture is gradually increased the representaion of graph strain (e) versus increment in temperature Δθ is
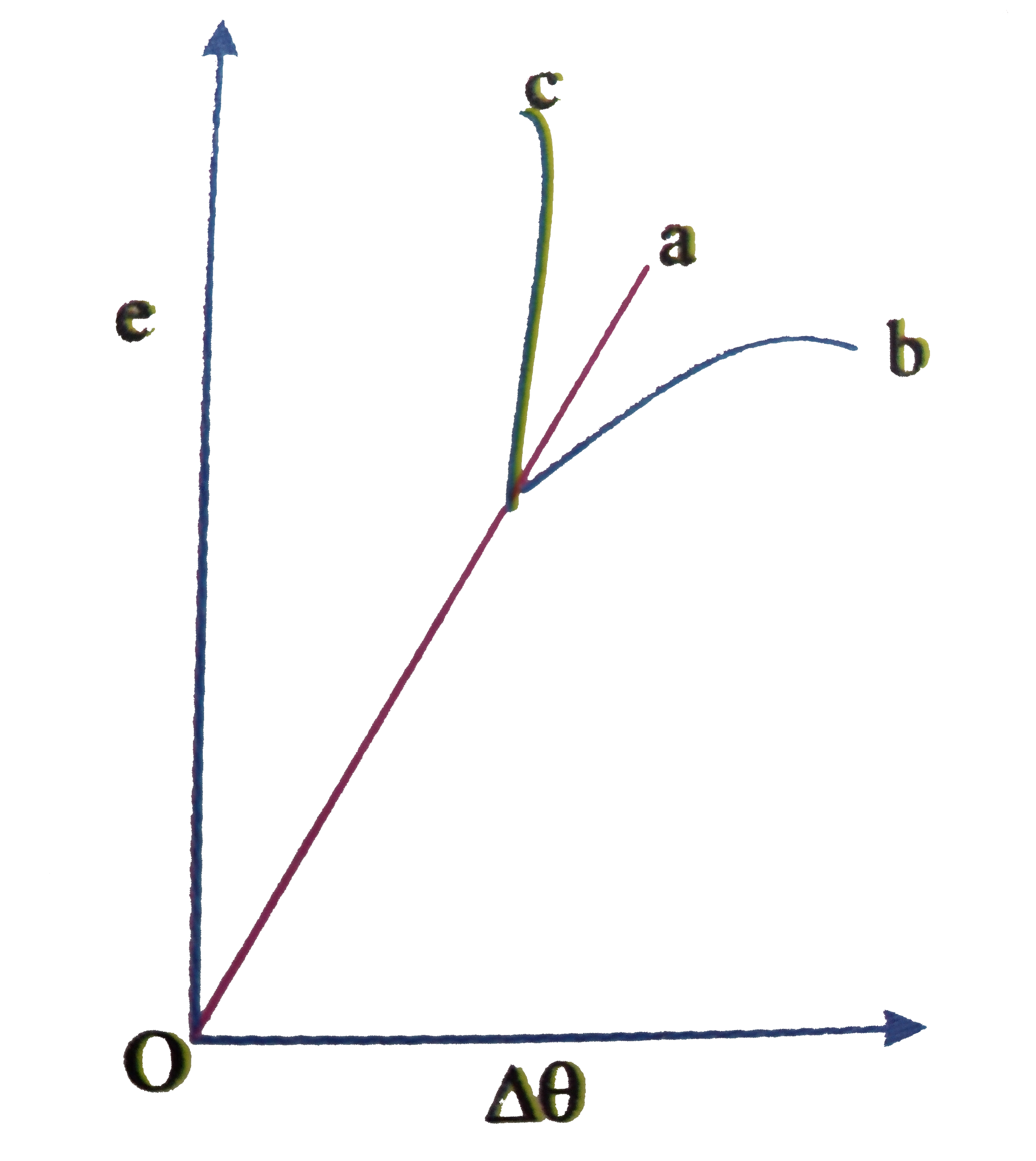
View SolutionA copper wire and a steel wire of the same length and same cross-section are joined end to end to form a composite wire. The composite wire is hung form a rigid support and a load is suspended form the other end. If the increses in length of the composite wire is 2.4mm. then the increases in lenghts of steel and copper wires are
View Solution
