Explain an experiment for the determination of Young's modulus of the material of a wire
Step-by-Step Solution for Determining Young's Modulus of a Wire
Step 1: Understanding Young's Modulus
- Young's modulus (Y) is defined as the ratio of stress to strain in a material. It can be expressed mathematically as:
Y=StressStrain=F/AΔL/L
where:
- F = applied force
- A = cross-sectional area of the wire
- ΔL = change in length of the wire
- L = original length of the wire
Step 2: Setting Up the Experiment
- Take a wire of known length L and securely attach it to a fixed point at one end.
- At the other end, attach a thin span or a weight. The total weight (force) applied will be the sum of the weight of the span and any additional weights added.
Step 3: Measuring the Original Length
- Measure the original length L of the wire using a measuring scale or vernier calipers for better precision.
Step 4: Applying the Force
- Attach a known mass m to the span. The force F acting on the wire can be calculated as:
F=(M+m)⋅g
where g is the acceleration due to gravity (approximately 9.81m/s2).
Step 5: Measuring the Elongation
- After applying the weight, allow the wire to come to rest and measure the new length L′ of the wire.
- Calculate the change in length ΔL using:
ΔL=L′−L
Step 6: Measuring the Cross-Sectional Area
- To find the cross-sectional area A of the wire, measure its diameter d using a screw gauge. The cross-sectional area can be calculated as:
A=πd24
Step 7: Calculating Young's Modulus
- Now that you have all the necessary values, substitute them into the Young's modulus formula:
Y=F⋅LA⋅ΔL
- Calculate Y to find the Young's modulus of the wire material.
- Young's modulus (Y) is defined as the ratio of stress to strain in a material. It can be expressed mathematically as:
Y=StressStrain=F/AΔL/L
where:
- F = applied force
- A = cross-sectional area of the wire
- ΔL = change in length of the wire
- L = original length of the wire
- Take a wire of known length L and securely attach it to a fixed point at one end.
- At the other end, attach a thin span or a weight. The total weight (force) applied will be the sum of the weight of the span and any additional weights added.
- Measure the original length L of the wire using a measuring scale or vernier calipers for better precision.
- Attach a known mass m to the span. The force F acting on the wire can be calculated as:
F=(M+m)⋅g
where g is the acceleration due to gravity (approximately 9.81m/s2).
- After applying the weight, allow the wire to come to rest and measure the new length L′ of the wire.
- Calculate the change in length ΔL using:
ΔL=L′−L
- To find the cross-sectional area A of the wire, measure its diameter d using a screw gauge. The cross-sectional area can be calculated as:
A=πd24
- Now that you have all the necessary values, substitute them into the Young's modulus formula:
Y=F⋅LA⋅ΔL
- Calculate Y to find the Young's modulus of the wire material.
Topper's Solved these Questions
- Mechanical Properties of SolidsBOOK - SL ARORACHAPTER - Mechanical Properties of SolidsEXERCISE - Example
113 Videos
- Mechanical Properties of fluidsBOOK - SL ARORACHAPTER - Mechanical Properties of fluidsEXERCISE - Exercise
459 Videos
- MECHANICSBOOK - SL ARORACHAPTER - MECHANICSEXERCISE - Exercise
341 Videos
Similar Questions
Knowledge Check
To determine Young's modulus of the material of a wire,
Atwo wires of same length and radius are takenBSingle straight wire of uniform cross section is takenCthree wires of different lengths but of same material are takenDtwo springs of same length but different materials are takenThe Young's modulus of the material of a wire is equal ot the
Astress required to increase its length four timesBstress required to produce unit strainCstrain produced in itDhalf the strain produced in itIn an experiment to determine the Young's modulus of the material of a wire, the length of the wire and the suspended mass are doubled. Then the Young's modulus of the wire
Abecomes double'sBbecomes four timeCremains unchangedDbecomes half
As temperture increases the Young's modulus of the material of a wire
View SolutionIn the experiment to determine Young's modulus of the material of a wire under tension
used in the arrangement as shown. The percentage error in the measurement
of length is a in the measurement of the radius of the wire is b and in the
measurement of the change in length of the wire is c. Percentage error in the measurement
of Young's modulus for a given load is
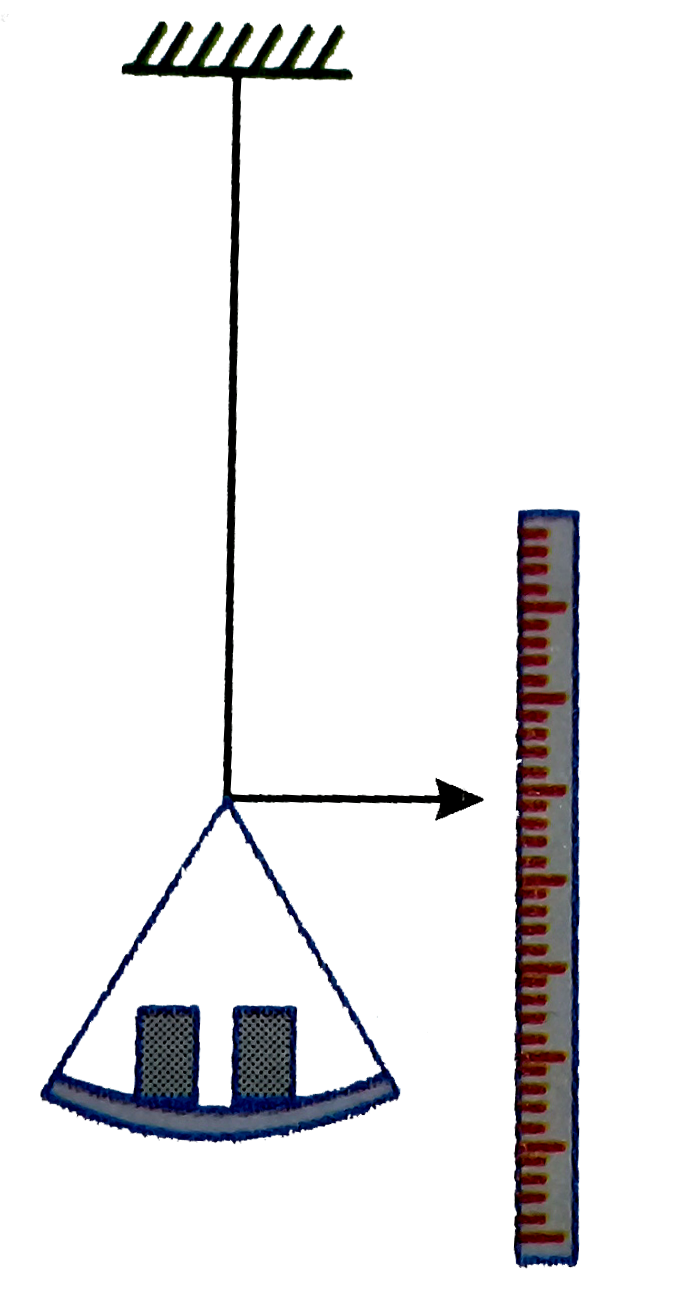
View SolutionIn the experiment to determine Young's modulus of the material of a wire under tension used in the arrangement as shown. The percentage error in the measurement of length is a in the measurement of the radius of the wire is b and in the measurement of the change in length of the wire is c. Percentage error in the measurement of Young's modulus for a given load is

View SolutionHow many number of wires are used in Searl's experiment for determining Young's modulus of elasticity ?
View SolutionWhich of the following apparatus is used to determine the Young's modulus of the material of a gives given wires?
View Solution