A copper ball 2 cm in radius is heated in a furnace at 327∘C If its emissivity is 0.3, at what rate does it radiate energy ?
1.72W
2.76W
11.0W
2.15W
To solve the problem of determining the rate at which a copper ball radiates energy, we will use the Stefan-Boltzmann law. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Given Data
- Radius of the copper ball, r=2cm=0.02m
- Temperature of the furnace, T=327∘C
- Emissivity, ϵ=0.3
Step 2: Convert Temperature to Kelvin
To use the Stefan-Boltzmann law, we need to convert the temperature from Celsius to Kelvin:
T(K)=T(°C)+273
T=327+273=600K
Step 3: Calculate the Surface Area of the Sphere
The surface area A of a sphere is given by the formula:
A=4πr2
Substituting the radius:
A=4π(0.02)2
Calculating A:
A=4π(0.0004)=0.005024m2
Step 4: Use the Stefan-Boltzmann Law
The rate of energy radiation dqdt is given by:
dqdt=ϵσAT4
Where:
- σ=5.67×10−8W/m2K4 (Stefan-Boltzmann constant)
Substituting the values:
dqdt=0.3×(5.67×10−8)×(0.005024)×(600)4
Step 5: Calculate T4
Calculating 6004:
6004=129600000000
Step 6: Substitute and Calculate
Now substituting back into the equation:
dqdt=0.3×(5.67×10−8)×(0.005024)×(129600000000)
Calculating the multiplication:
dqdt=0.3×5.67×10−8×0.005024×129600000000
Calculating step by step:
1. 5.67×10−8×0.005024≈2.855×10−10
2. 2.855×10−10×129600000000≈37.02
3. Finally, 0.3×37.02≈11.106
Final Result
Thus, the rate at which the copper ball radiates energy is approximately:
dqdt≈11.106W
Conclusion
The rate of energy radiation from the copper ball is approximately 11.1 Watts.
---
- Radius of the copper ball, r=2cm=0.02m
- Temperature of the furnace, T=327∘C
- Emissivity, ϵ=0.3
To use the Stefan-Boltzmann law, we need to convert the temperature from Celsius to Kelvin:
T(K)=T(°C)+273
T=327+273=600K
The surface area A of a sphere is given by the formula:
A=4πr2
Substituting the radius:
A=4π(0.02)2
Calculating A:
A=4π(0.0004)=0.005024m2
The rate of energy radiation dqdt is given by:
dqdt=ϵσAT4
Where:
- σ=5.67×10−8W/m2K4 (Stefan-Boltzmann constant)
dqdt=0.3×(5.67×10−8)×(0.005024)×(600)4
Calculating 6004:
6004=129600000000
Now substituting back into the equation:
dqdt=0.3×(5.67×10−8)×(0.005024)×(129600000000)
Calculating the multiplication:
dqdt=0.3×5.67×10−8×0.005024×129600000000
Calculating step by step:
1. 5.67×10−8×0.005024≈2.855×10−10
2. 2.855×10−10×129600000000≈37.02
3. Finally, 0.3×37.02≈11.106
Thus, the rate at which the copper ball radiates energy is approximately:
dqdt≈11.106W
The rate of energy radiation from the copper ball is approximately 11.1 Watts.
Topper's Solved these Questions
- Thermal Properties of MatterBOOK - SL ARORACHAPTER - Thermal Properties of MatterEXERCISE - Exercise
449 Videos
- System of particles & rotational MotionBOOK - SL ARORACHAPTER - System of particles & rotational MotionEXERCISE - EXERCISE
353 Videos
- THERMODYNAMICSBOOK - SL ARORACHAPTER - THERMODYNAMICSEXERCISE - Exercise
342 Videos
Similar Questions
A thin square steel plate 10 cm on a side is heated in a black smith's forge to temperature of 800∘C. If the emissivity is 0.60, what is the total rate of radiation of energy ?
View SolutionA spherical ball of radius 1cm coated with a metal having emissivity 0.3 is maintained at 1000 K temperature and suspended in a vacuum chamber whose walls are maintained at 300 K temperature. Find rate at which electrical energy is to be supplied to the ball to keep its temperature constant.
View Solution
Knowledge Check
A metal ball of surface area 200cm2 and temperature 527∘C is surrounded by a vessel at 27∘C . If the emissivity of the metal is 0.4, then the rate of loss of heat from the ball is (σ=5.67×10−8J/m2−s−k4)
A108WB168WC182WD192WAssertion : When an iron rod is heated in a furnace, the radiation emitted goes from a lower frequency to a higher frequency as the temperature increases.
Reason : The energy of a quantum of radiation is proportional to its frequency.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.A spherical body of 5 cm radius is maintained at a temperature of 327∘C. The wavelength at which maximum energy radiated will be nearly (b=2.898×10−3m K)
A482 ÅB4.82 ÅC482 μ mD4.82 μ m
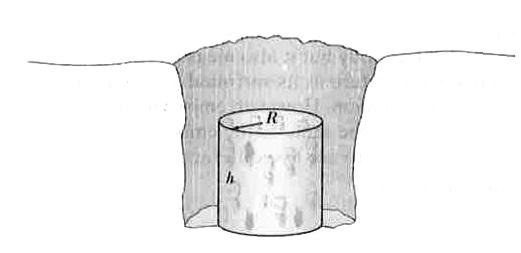
Unlike most other plants, a special type of cabbage called skunk cabbage can regulate its internal temperature ( set at T = 22∘C )by altering the rate at which it produces energy. If it becomes covered with snow, it can increase that its thermal radiation metls the snow in order to re-expose the plant to sunlight. Let's model a skunk cabbage with a cylinder of height h = 5.0 cm and radius R = 1.5 cm and assume it is surrounded by a snow wall at temperature Tenv=−3.0∘C (Figure) . If the emissivity ε is 0.80, what is the net rate of energy exchange via thermal radiation between the plant's curved side and the snow ?
View SolutionA blackened solid copper sphere of radius 2 cm is placed in an evacuated enclosure whose walls are kept at 100∘C. At what rate must energy be supplied to the sphere to keep its temperature constant at 127∘C ? Stefan constant =5.67×10−8Jm−2K−4.
View SolutionA metal ball of surface area 200cm2 and temperature 527∘C is surrounded by a vessel at 27∘C . If the emissivity of the metal is 0.4, then the rate of loss of heat from the ball is (σ=5.67×10−8J/m2−s−k4)
View SolutionA copper block of mass 2.5kg is heated in a furnace to a temperature of 500∘C and then placed on a large ice block. What is the maximum amount (approx.) of ice that can melt? (Specific heat copper =0.39J/g∘C heat of fusion of water =335J/g).
View SolutionA black body maintained at a certain temperature radiates heat energy at the rate Q watt. If its surface is smoothened so as to lower its emissivitiy by 10%, what will be the increase in its rate of radiation at double the initial temperature?
View Solution
