The time-period of a simple pendulum is 2 s and it can go to and fro from equilibrium position at maximum distance of 5 cm. If at the start of the motion the pendulum is in the position of maximum displacement towards the right of the equilibrium position, then write the displacement equation of the pendulum.
To find the displacement equation of the pendulum, we can follow these steps:
Step 1: Identify the given values
- Time period (T) = 2 seconds
- Maximum displacement (Amplitude, A) = 5 cm
Step 2: Calculate the angular frequency (ω)
The angular frequency (ω) can be calculated using the formula:
ω=2πT
Substituting the value of T:
ω=2π2=πrad/s
Step 3: Write the general equation of displacement
The general equation for the displacement of a simple harmonic oscillator is given by:
y(t)=Asin(ωt+ϕ)
where:
- y(t) is the displacement at time t,
- A is the amplitude,
- ω is the angular frequency,
- ϕ is the phase constant.
Step 4: Determine the phase constant (φ)
Since the pendulum starts at maximum displacement towards the right, we can use the cosine function instead of sine. At maximum displacement, the sine function is at its maximum when the phase is π2:
y(t)=Asin(ωt+π2)
Using the identity sin(x+π2)=cos(x), we can rewrite the equation as:
y(t)=Acos(ωt)
Step 5: Substitute the values of A and ω
Substituting A=5 cm and ω=π:
y(t)=5cos(πt)
Final Answer
The displacement equation of the pendulum is:
y(t)=5cos(πt)cm
---
- Time period (T) = 2 seconds
- Maximum displacement (Amplitude, A) = 5 cm
The angular frequency (ω) can be calculated using the formula:
ω=2πT
Substituting the value of T:
ω=2π2=πrad/s
The general equation for the displacement of a simple harmonic oscillator is given by:
y(t)=Asin(ωt+ϕ)
where:
- y(t) is the displacement at time t,
- A is the amplitude,
- ω is the angular frequency,
- ϕ is the phase constant.
Since the pendulum starts at maximum displacement towards the right, we can use the cosine function instead of sine. At maximum displacement, the sine function is at its maximum when the phase is π2:
y(t)=Asin(ωt+π2)
Using the identity sin(x+π2)=cos(x), we can rewrite the equation as:
y(t)=Acos(ωt)
Substituting A=5 cm and ω=π:
y(t)=5cos(πt)
The displacement equation of the pendulum is:
y(t)=5cos(πt)cm
Topper's Solved these Questions
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Problem
23 Videos
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Problems for self practice
67 Videos
- OSCILLATIONSBOOK - SL ARORACHAPTER - OSCILLATIONSEXERCISE - Type D Based on Other Examples of SHM
2 Videos
- MOTION IN ONE DIMENSIONBOOK - SL ARORACHAPTER - MOTION IN ONE DIMENSIONEXERCISE - problems for self practice
66 Videos
- Physical worldBOOK - SL ARORACHAPTER - Physical worldEXERCISE - Exercise
49 Videos
Similar Questions
Time period of a simple pendulum is 2s and it can go to and fro from equilibrium position at a maximum distance of 6cm. If at the start of the motion the pendulum is in the position of maximum displacement towards the right of the equilibrium position, then write the displacement equation of the pendulum.
View SolutionTime period of a back performing SHM is 2 second and it can travel to and fro from equilibrium position upto maximum 5cm. At start the pendulum is at maximum displacement on right side of equilibrium position. Find displacement and time relation.
View Solution
Knowledge Check
The maximum displacement of the constituents of the medium from their equilibrium positions is called
AwavelengthBfrequencyCamplitudeDangular wave numberThe periodic time of a simple pendulum is 6.28 sec and the amplitude of oscillation is 3 cm. The maximum acceleration of the pendulum is
A5cm/s2B4cm/s2C3cm/s2D2cm/s2A pendulum is displaced to an angle θ from its equilibrium position, then it will pass through its mean position with a velocity v equal to
A√2glB√2glsinθC√2glcosθD√2gl(1−cosθ)
For a simple pendulum, the time period is 3 s and has a maximum displacement of 5 cm on either side of its mean position. If at the beginning of the motion, the pendulum is at maximum displacement from its mean position, towards its right, then derive the displacement equation of the pendulum.
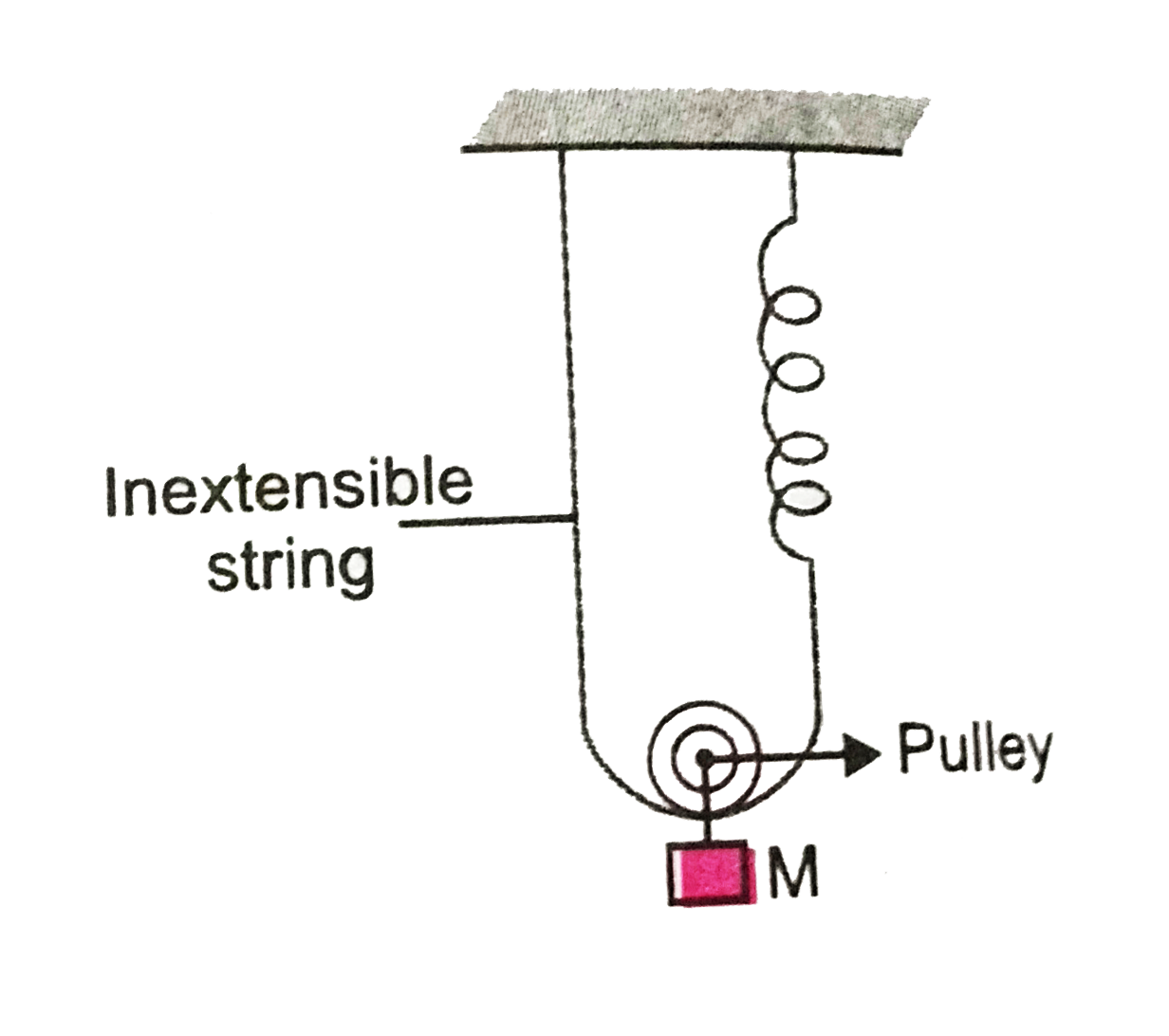
View SolutionFind the time period of mass M when displaced from its equilibrium position and then released for the system shown in figure.

View SolutionFind the time period of mass M when displaced from its equilibrium position and then reelased for the system shown in figure.
View SolutionA simple pendulum has length L and period T. As it passes through its equilibrium position, the string is suddenly clamped at its midpoint. The period then becomes
View SolutionA simple pendulum oscillates in a vertical plane. When it passes through the mean position, the tension in the string is 3 times the weight of the pendulum bob.what is the maximum displacement of the pendulum with respect to the vertical
View Solution