A massless spring of natural length of 0.5 m and spring constant 50 N/m has one end fixed and the other end attached to a mass of 250 g. The spring mass system is on a smooth floor. The mass is pulled until the length of the spring is 0.6 m and then released from rest. The kinetic energy of the mass when the length of the spring is 0.5 m is
0.25 J
2.25 J
6.25 J
9 J
To solve the problem, we need to calculate the kinetic energy of a mass attached to a spring when the spring returns to its natural length after being stretched. Here are the steps to find the solution:
Step 1: Identify the Given Data
- Natural length of the spring, L0=0.5m
- Spring constant, k=50N/m
- Mass attached to the spring, m=250g=0.25kg
- Stretched length of the spring, L=0.6m
Step 2: Calculate the Displacement from the Natural Length
The displacement x from the natural length when the spring is stretched to 0.6 m is:
x=L−L0=0.6m−0.5m=0.1m
Step 3: Calculate the Potential Energy Stored in the Spring
When the spring is stretched, it stores potential energy given by the formula:
PE=12kx2
Substituting the values:
PE=12×50N/m×(0.1m)2=12×50×0.01=0.25J
Step 4: Understand Energy Conservation
When the mass is released from the stretched position and moves back to the natural length of the spring (0.5 m), all the potential energy converts into kinetic energy (KE) at that point. Thus:
KE=PE=0.25J
Step 5: Conclusion
The kinetic energy of the mass when the length of the spring is 0.5 m is:
Kinetic Energy=0.25J
---
- Natural length of the spring, L0=0.5m
- Spring constant, k=50N/m
- Mass attached to the spring, m=250g=0.25kg
- Stretched length of the spring, L=0.6m
The displacement x from the natural length when the spring is stretched to 0.6 m is:
x=L−L0=0.6m−0.5m=0.1m
When the spring is stretched, it stores potential energy given by the formula:
PE=12kx2
Substituting the values:
PE=12×50N/m×(0.1m)2=12×50×0.01=0.25J
When the mass is released from the stretched position and moves back to the natural length of the spring (0.5 m), all the potential energy converts into kinetic energy (KE) at that point. Thus:
KE=PE=0.25J
The kinetic energy of the mass when the length of the spring is 0.5 m is:
Kinetic Energy=0.25J
Topper's Solved these Questions
Similar Questions
When a 1.0kg mass hangs attached to a spring of length 50cm, the spring stretches by 2cm. The mass is pulled down until the length of the spring becomes 60cm. What is the amount of elastic energy stored in the spring in this condition. if g=10m/s2.
View SolutionTwo blocks A and B each of mass m are connected by a massless spring of natural length L and spring constant k. The blocks are initially resting on a smooth horizontal floor with the spring at its natural length. A third identical block C also of mass m moves on the floor with a speed v along the line joining A and B and collides with A. Then
The kinetic energy of the A-B system at maximum compression of the spring is mv2/4
The maximum compression of the spring v√m/3k<br>Thek∈etice≠rgyoftheA−Bsystematmaxiμmcompression<br>Themaxiμmcoppressionofthespr∈gisvsqrt(m//k)
View Solution
Knowledge Check
When a 20 g mass hangs attached to one end of a light spring of length 10 cm, the spring stretches by 2 cm. The mass is pulled down until the total length of the spring is 14 cm. The elastic energy, (in Joule) stored in the spring is :
A4×10−2B4×10−3C8×10−2D8×10−3If the system is suspended by the mass m the length of the spring is l1. If it is inverted and hung by mass M, the length of the spring is l2. Find the natural length of the spring.
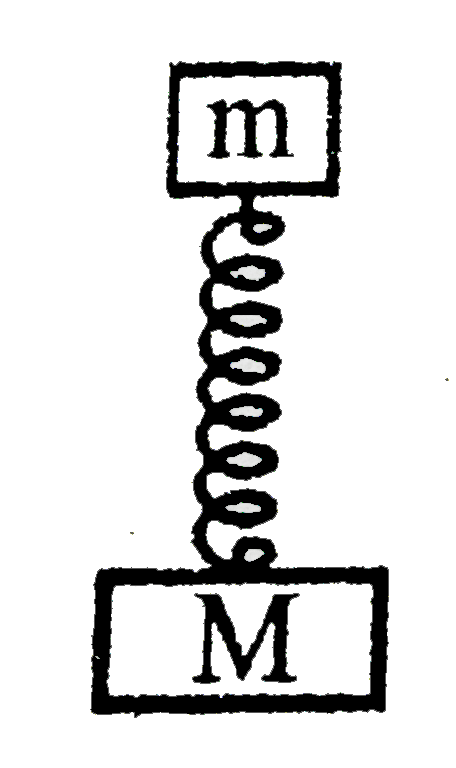 Aml1+Ml2m+MBml2+Ml1m+MCml1−Ml2m−MDml2−Ml1m−M
Aml1+Ml2m+MBml2+Ml1m+MCml1−Ml2m−MDml2−Ml1m−MA spring has natural length 40cm and spring constant 500N/m. A block of mass 1kg is attached at one end of the spring and other end of the spring is attached to a ceiling. The block is relesed from the position, where the spring has length 45cm.
Athe block will perform SHM of amplitude 5cmBthe block will have maximum velocity 30√5cm/sCthe block will have maximum acceleration 15m/s2Dthe minimum elastic potential energy of the spring will be zero
A planck of mass 5kg is placed on a frictionless horizontal plane. Further a block of mass 1kg is placed over the plank. A massless spring of natural length 2m is fixed to the plank by its one end. The other end of spring is compressed by the block by half of spring's natural length. They system is now released from the rest. What is the velocity of the plank when block leaves the plank? (The stiffness constant of spring is 100N/m)

View SolutionA massless spring of length l and spring constant k is placed vertically on a table. A ball of mass m is just kept on top of the spring. The maximum velocity of the ball is
View SolutionTwo masses m and 2m are attached to two ends of an ideal spring as shown in figure. When thye spring is in the compressed state, the energy of the spring is 60 J, if the spring is released, then at its natural length

View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionA weightless spring of length 60 cm and force constant 200 N/m is kept straight and unstretched on a smooth horizontal table and its ends are rigidly fixed. A mass of 0.25 kg is attached at the middle of the spring and is slightly displaced along the length. The time period of the oscillation of the mass is
View Solution
