A person P is 600 m away from the station. When train is approaching station with 72 km/h, it blows a whistle of frequency 800 Hz when 800 m away from the station. Find the frequency heard by the person. Speed of sound = 340 ms−1.
800 Hz
839.5 Hz
829.5 Hz
843.5 Hz
To solve the problem of finding the frequency heard by person P when a train approaches the station, we will use the Doppler effect formula. Here’s the step-by-step solution:
Step 1: Identify the given data
- Distance of person P from the station = 600 m
- Distance of the train from the station when it blows the whistle = 800 m
- Speed of the train (source) = 72 km/h
- Frequency of the whistle (source frequency, f₀) = 800 Hz
- Speed of sound (v) = 340 m/s
Step 2: Convert the speed of the train from km/h to m/s
To convert the speed from km/h to m/s, we use the conversion factor 518:
Speed of train(vs)=72km/h×518=20m/s
Step 3: Use the Doppler effect formula
The formula for the frequency heard by the observer when the source is moving towards the observer is:
f′=f0v+vov−vs
Where:
- f′ = frequency heard by the observer
- f0 = frequency of the source (800 Hz)
- v = speed of sound (340 m/s)
- vo = speed of the observer (0 m/s, since the person is stationary)
- vs = speed of the source (20 m/s)
Step 4: Substitute the values into the formula
Substituting the known values into the formula:
f′=800Hz×340m/s+0m/s340m/s−20m/s
f′=800Hz×340320
Step 5: Calculate the frequency
Now, we calculate f′:
f′=800Hz×1.0625=850Hz
Conclusion
The frequency heard by person P is approximately 850 Hz.
---
- Distance of person P from the station = 600 m
- Distance of the train from the station when it blows the whistle = 800 m
- Speed of the train (source) = 72 km/h
- Frequency of the whistle (source frequency, f₀) = 800 Hz
- Speed of sound (v) = 340 m/s
To convert the speed from km/h to m/s, we use the conversion factor 518:
Speed of train(vs)=72km/h×518=20m/s
The formula for the frequency heard by the observer when the source is moving towards the observer is:
f′=f0v+vov−vs
Where:
- f′ = frequency heard by the observer
- f0 = frequency of the source (800 Hz)
- v = speed of sound (340 m/s)
- vo = speed of the observer (0 m/s, since the person is stationary)
- vs = speed of the source (20 m/s)
Substituting the known values into the formula:
f′=800Hz×340m/s+0m/s340m/s−20m/s
f′=800Hz×340320
Now, we calculate f′:
f′=800Hz×1.0625=850Hz
The frequency heard by person P is approximately 850 Hz.
Similar Questions
A train is moving with speed 72 km/h towards a hill blows a whistle of frequency 1240Hz. the frequency of echo heard by driver is(speed of sound=330m/s)
View SolutionA train producing frequency of 640Hz is moving towards point c with speed 72km/hr. A person is sitting 1732m from point c as shown. Find the frequency heard by person if speed of sound is 330m/s.
View Solution
Knowledge Check
A person is standing on a railway platform.An engine blowing a whistle of frequency 640 Hz approaches him with a speed of 72 km /hr,The frequency of the note heard by the person is (velocity of sound is 340 m/s )
A650 HzB660 HzC675 HzD680 HzA train is approaching towards a platform with a speed of 10ms−1, while blowing a whistle of frequency 340 Hz. What is the frequency of whistle heard by a stationary observer on the platform ?
(given, speed of sound =340ms−1)A330 HzB350 HzC340 HzD360 HzA train is moving with a uniform speed of 33 m/s and an observer is approaching the train with the same speed. If the train blows a whistle of frequency 1000 Hz and the velocity of sound is 333 m/s, then the apparent frequency of the sound that the observer hears is
A1220 HzB1099 HzC1110 HzD1200 Hz
A train moving a uniform velocity of 180km/h, approaches another train standing on a platform. An observer O moves with a uniform velocity of 36km/h towards , as shown in figure. Both the trains are blowing whistles of same frequency 120 Hz. When O is 600 m away from and distance between and is 800 m, the number of beats heard by O is x. Find x.
[Speed of the sound = 330 m/s]
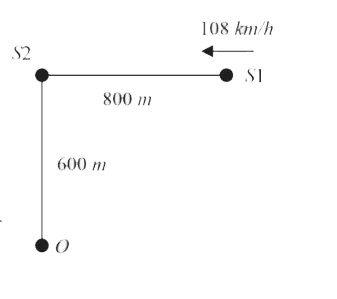
View SolutionA car moving at 108kmh−1 finds another car in front of it going in the same direction at 72kmh−1. The first car sounds a horn that has a dominant frequency of 800 Hz. What will be the apparent frequency heard by the driver in the front car ? Speed of sound in air =330ms−1
View SolutionAn observer on a railway station platform observed that as the train passed the station at 96 kmph, the frequency of the whistle appeared to drop by 400 Hz. Find the frequency of the whistle. (Velocity of sound =1200km/h)
View SolutionA person going away from a factory on his scooter at a speed of 36 km/hr listens to the siren of the factory. If the actual frequency of the siren is 700 Hz and a wind is blowing along the direction of the scooter at 36 km//hr, find the observed frequency heard by the person. (Given speed of sound =340m/s)
View SolutionA train S1, moving with a uniform velocity of 108 km/h, approaches another train S2 standing on a platform. An observer O moves with a uniform velocity of 36 km/h towards S2, as shown in figure. Both the trains are blowing whistles of same frequency 120Hz. When O is 600 m away form S2 and distance between S1 and S2 is 800 m, the number of beats heard by O is ....... [Speed of the sound = 330 m/s]
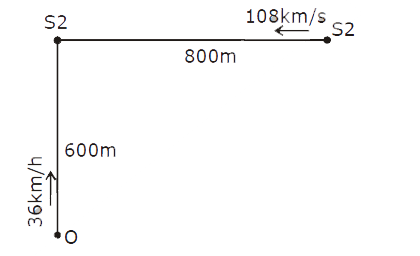
View Solution
