Passage -II The figure shows the variation of potential energy of a particle as a function of x, the X-coordinate of the region. It has been assumed that potential energy depends only on X. For all other values of x, U is zro, i.e. for x<−10 and x>15,U=0.
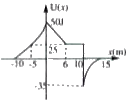
If the particle is isolated and its total mechanical energy is 60 J, then
the particle can be found anywhere from −∞<x<∞
the particle's maximum KE is 95 J
the particle 's KE is not getting zero anywhere onX-axis
all of the above are true
The correct Answer is:D
Topper's Solved these Questions
- WORK -POWER-ENERGYBOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - LECTURE SHEET (EXERCISE-VI POWER STRAIGHT OBJECTIVE TYPE QUESTIONS)
6 Videos
- WORK -POWER-ENERGYBOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - LECTURE SHEET (EXERCISE -VII VERTICAL CIRCLE AND SPRINGS STRAIGHTS OBJECTIVE TYPE QUESTIONS)
15 Videos
- WORK -POWER-ENERGYBOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - LECTURE SHEET (EXERCISE-IV POTENTIAL ENERGY STRAIGHT OBJECTIVE TYPE QUESTIONS)
8 Videos
- VISCOSITY BOOK - AAKASH SERIESCHAPTER - VISCOSITY EXERCISE - Questions for Descriptive Answers
3 Videos
- WORK POWER ENERGYBOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 3
61 Videos
Similar Questions
Figure shows the variation of potential energy of a particle as a function of x, the x-coordinate of the region. It has been assumed that potential energy depends only on x. For all other values of x, U is zero, i.e., x←10 and x>15, U=0.
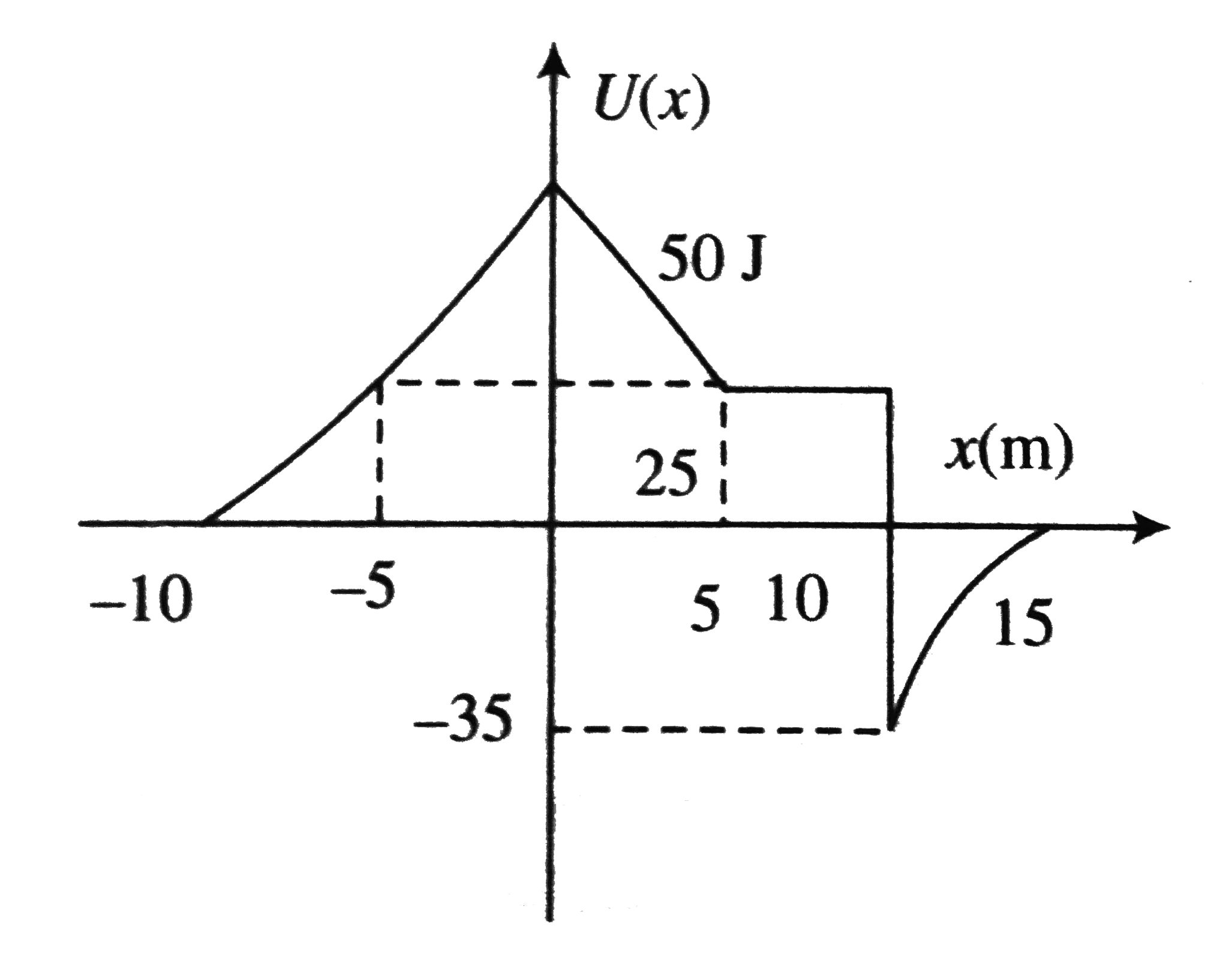
If the particle is isolated and its total mechanical energy is 60J, then
View SolutionThe figure shows the variation of potential energy of a particle as a function pf x, the x-coordination of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.

If total mechanical energy of the particle is 25J, then it can found in the region
View Solution
Knowledge Check
Figure shows the variation of potential energy of a particle as a function of x, the x-coordinate of the region. It has been assumed that potential energy depends only on x. For all other values of x, U is zero, i.e., x←10 and x>15, U=0.

If the particle is isolated and its total mechanical energy is 60J, thenA(a) The particle can be found anywhere from −∞<x<∞.B(b) The particle's maximum kinetic energy is 95J.C(c) The particle's kinetic energy is not getting zeroD(d) All of the aboveThe figure shows the variation of potential energy of a particle as a funcation pf x, the x-coordination of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.

If total mechanical energy of the particle is 25J, then it can found in the regionA−10<x<−5 and 6<x<15B−10<x<0 and 6<x<10C−5<x<6D−10<x<10Figure shows the variation of potential energy of a particle as a function of x, the x-coordinate of the region. It has been assumed that potential energy depends only on x. For all other values of x, U is zero, i.e., x←10 and x>15, U=0.
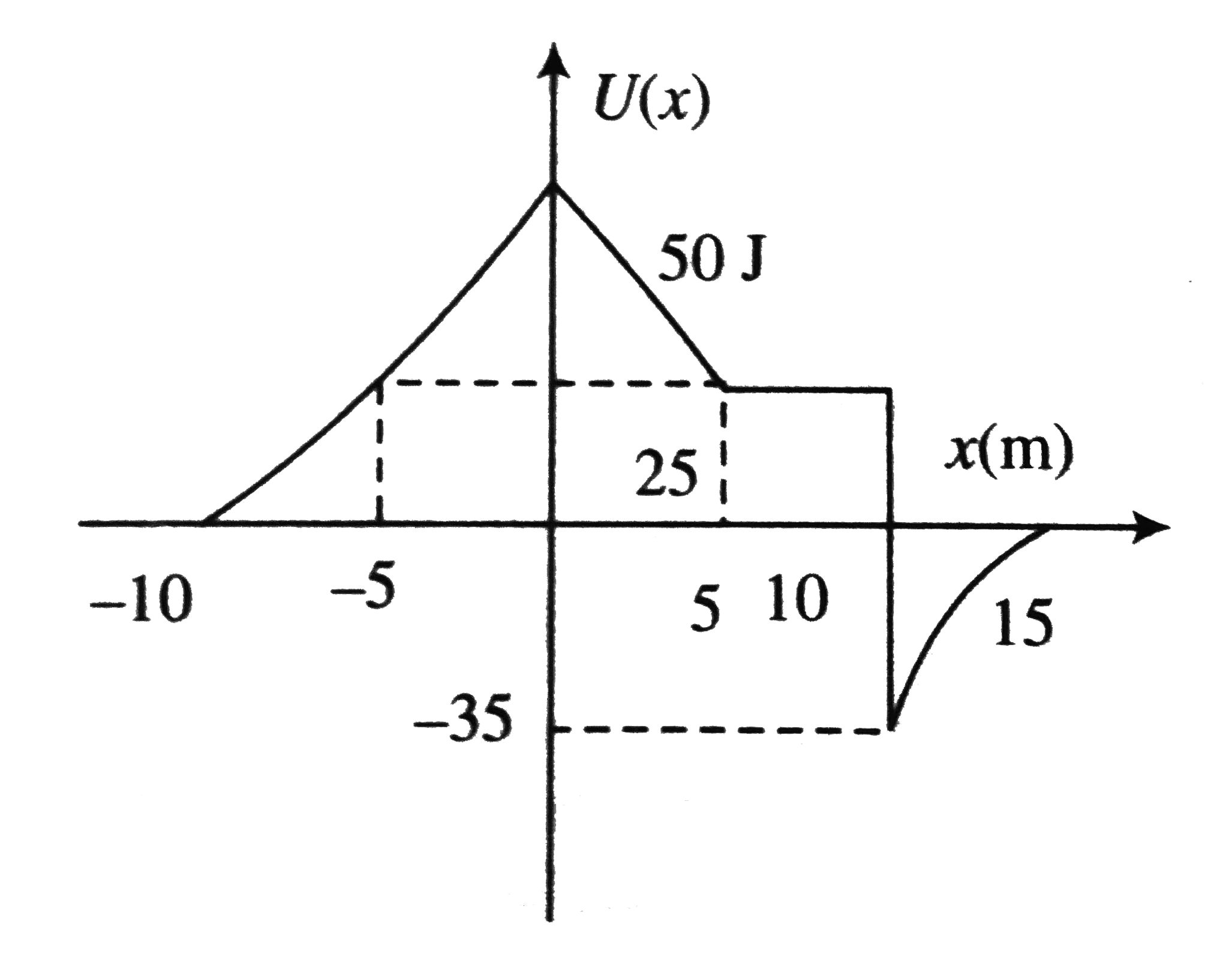
If the total mechanical energy of the particle is −40J, then it can be found in regionA(a) x←10 and x>15B(b) −10<x←5 and 6<x<15C(c) 10<x<15D(d) It is not possible
The figure shows the variation of potential energy of a particle as a funcation of x, the x-coordinate of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.
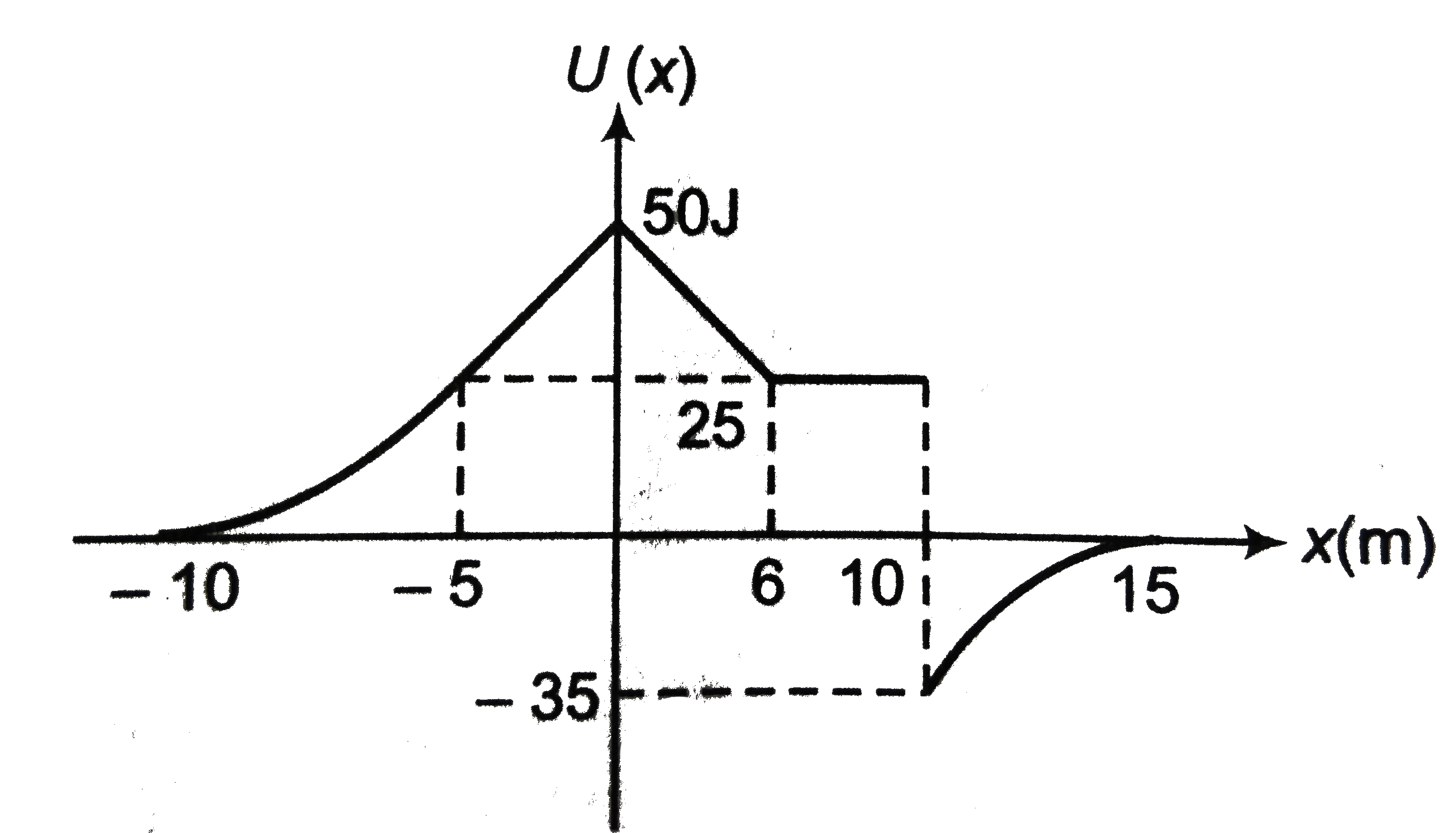
If total mechanical energy of the particle is −40J, then it can be found in region.
View SolutionThe figure shows the variation of potential energy of a particle as a funcation pf x, the x-coordination of the region. It has been assumed that potential energy depends only on x. For all other values x,U is zero. i.e. forx<−10 and x>15,U=0.

If total mechanical energy of the particle is −40J, then it can be found in region.
View SolutionThe potential energy of a particle (Ux) executing SHM is given by
View SolutionIf x,F and U denote the dispalcement, force acting on and potential energy of a particle then
View SolutionThe potential energy of a particle is given by formula U=100−5x+100x2,whereU and are .
View Solution
