A body of mass 6g is in rectilinear motion according to the laws=−1+(t+1)+(t+1)3 (s is in centimetres and t, in seconds). Find the kinetic enery of the body one second after it begins to move.
To find the kinetic energy of a body with a mass of 6g one second after it begins to move, we will follow these steps:
Step 1: Understand the given function
The position of the body is given by the equation:
s=−1+(t+1)+(t+1)3
where s is in centimeters and t is in seconds.
Step 2: Differentiate the position function to find velocity
The velocity v is the derivative of the position s with respect to time t:
v=dsdt
Differentiating s:
1. The derivative of −1 is 0.
2. The derivative of (t+1) is 1.
3. For (t+1)3, we use the chain rule:
ddt((t+1)3)=3(t+1)2⋅ddt(t+1)=3(t+1)2⋅1=3(t+1)2
Putting it all together:
v=0+1+3(t+1)2=1+3(t+1)2
Step 3: Calculate the velocity at t=1 second
Substituting t=1 into the velocity equation:
v=1+3(1+1)2=1+3(2)2=1+3⋅4=1+12=13cm/s
Step 4: Calculate the kinetic energy
The kinetic energy KE is given by the formula:
KE=12mv2
where m is the mass in kilograms. Since the mass is given in grams, we convert it:
m=6g=0.006kg
Now substituting the values:
KE=12⋅0.006⋅(13)2
Calculating (13)2:
(13)2=169
Now substituting:
KE=12⋅0.006⋅169=0.003⋅169=0.507J
Final Answer
The kinetic energy of the body one second after it begins to move is:
KE=0.507J
The position of the body is given by the equation:
s=−1+(t+1)+(t+1)3
where s is in centimeters and t is in seconds.
The velocity v is the derivative of the position s with respect to time t:
v=dsdt
1. The derivative of −1 is 0.
2. The derivative of (t+1) is 1.
3. For (t+1)3, we use the chain rule:
ddt((t+1)3)=3(t+1)2⋅ddt(t+1)=3(t+1)2⋅1=3(t+1)2
v=0+1+3(t+1)2=1+3(t+1)2
Substituting t=1 into the velocity equation:
v=1+3(1+1)2=1+3(2)2=1+3⋅4=1+12=13cm/s
The kinetic energy KE is given by the formula:
KE=12mv2
where m is the mass in kilograms. Since the mass is given in grams, we convert it:
m=6g=0.006kg
KE=12⋅0.006⋅(13)2
Calculating (13)2:
(13)2=169
Now substituting:
KE=12⋅0.006⋅169=0.003⋅169=0.507J
The kinetic energy of the body one second after it begins to move is:
KE=0.507J
Topper's Solved these Questions
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.6 (APPLICATION OF THE DERIVATIVE)
3 Videos
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.7 (ADDITIONAL PROBLEMS)
7 Videos
- DIFFERENTIATION OF FUNCTIONS BOOK - IA MARONCHAPTER - DIFFERENTIATION OF FUNCTIONS EXERCISE - 2.4 (DIFFERENTIATION OF INVERSE, IMPLICIT AND PARAMETRICALLY REPRESENTED FUNCTIONS)
2 Videos
- BASIC CLASSES OF INTEGRABLE FUNCTIONS BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS
5 Videos
- IMPROPER INTEGRALSBOOK - IA MARONCHAPTER - IMPROPER INTEGRALSEXERCISE - 8.4 ADDITIONAL PROBLEMS
1 Videos
Similar Questions
Knowledge Check
A body whose mass is 3kg performs rectilinear motion according to the formula s=1+t+t2, where s is measured the kinetic energy 12mv2 and t in second.
Determine the kinetic energy 12mv2 of the body in 5sec after its start.A1.815×105 ergB1.815×107 ergC1.715×1015 ergD1.815×108 ergA body o fmass 6 kg moves in a staight line according to the equation x=t3−75t, where x denotes the distance in metre and t the time in second. The force on the body at t=4s is
A64 NB72 NC144 ND36 NIf a particle moves according to the law s=6t2−t32, then the time at which it is momentarily at rest is
At=0 onlyBt=8 onlyCt=0,8Dnone of these
A particle is moving such that s=t3−6t2+18t+9, where s is in meters and t is in meters and t is in seconds. Find the minimum velocity attained by the particle.
View SolutionA particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionA particle is moving in a straight line according to the law S=t3+2t2+3t−4, where S is displacement and t is time. Find the velocity and acceleration when t=2.
View SolutionA body moves in a straight line according to the law s=t3−4t2−3t. What are the values of time and acceleration (time, acceleration), each time when velocity of the body is zero ?
View SolutionIn the figure shown q is in coulomb and t in second. At time t=1s
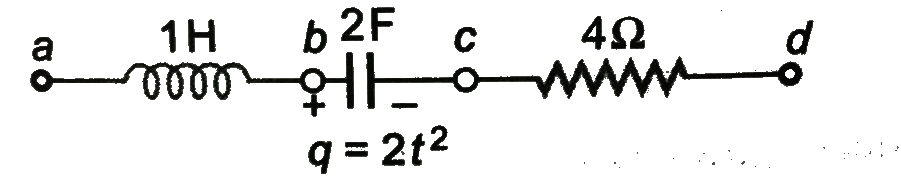
View Solution
