According to Bohr.s theory, En = total energy, Kn = kinetic energy, Vn = potential energy, rn = radius of nth orbit. Match the following.

The correct Answer is:A-R ; B-Q ; C-P , D-S
According to Bohr.s theory , kinetic energy
(Kn)=Ze22r
Potential energy (Vn)=−Ze2r,
Total energy (En)=−Ze22r
A) VnKn=−Ze2/rZe/2r=−2
B) rn∝n2andEn∝1n2 , i.e. , En∝1rn
C) Angular momentum =√l(l+1)h2π
The lowest orbital is 1s for which l=0
Hence angular momentum =0
D)rn∝1Z or 1rn∝Z∴y=1
Similar Questions
According to Bohr's theory
En= Total energy Kn= Kinetic energy
Vn= Potential energy rn=Radius of nth orbit
Match the following :
Column -I Column -II
(A) Vn/Kn=? ( p ) 0
(B ) If radius of nth orbit ∝Enx,x=? ( q) -1
( C ) Angular momentum in lowest orbital ( r ) - 1
(D ) 1rn∝Z4,y=? ( s ) 1
View SolutionAccording to Bohr’s theory En= Total energy, Kn= Kinetic energy, Vn= Potential energy Rn= Radius of nth orbit:

View Solution
Knowledge Check
According to bohr's theory of H atom, for the electron in the nth allowed orbit
Alinear momentum is proportional to (1/n)Bthe K.E. is proportional to (1/n2)Cthe angular momentum is proportional to nDall of theseAccording to the Bohr theory of Hydrogen atom, the speed of the electron, its energy and the radius of its orbit varies with the principal quantum number n, respectively, as
A1n,1n2,n2B1n,n2,1n2Cn2,1n2,n2Dn,1n2,1n2Potential energy (PEn) and kinetic energy (KEn) of electron in nth orbit are related as
APEn=KEnBPEn=−2KEnCPEn=2KEnDPEn=KEn
" Energy of the nth orbit "-
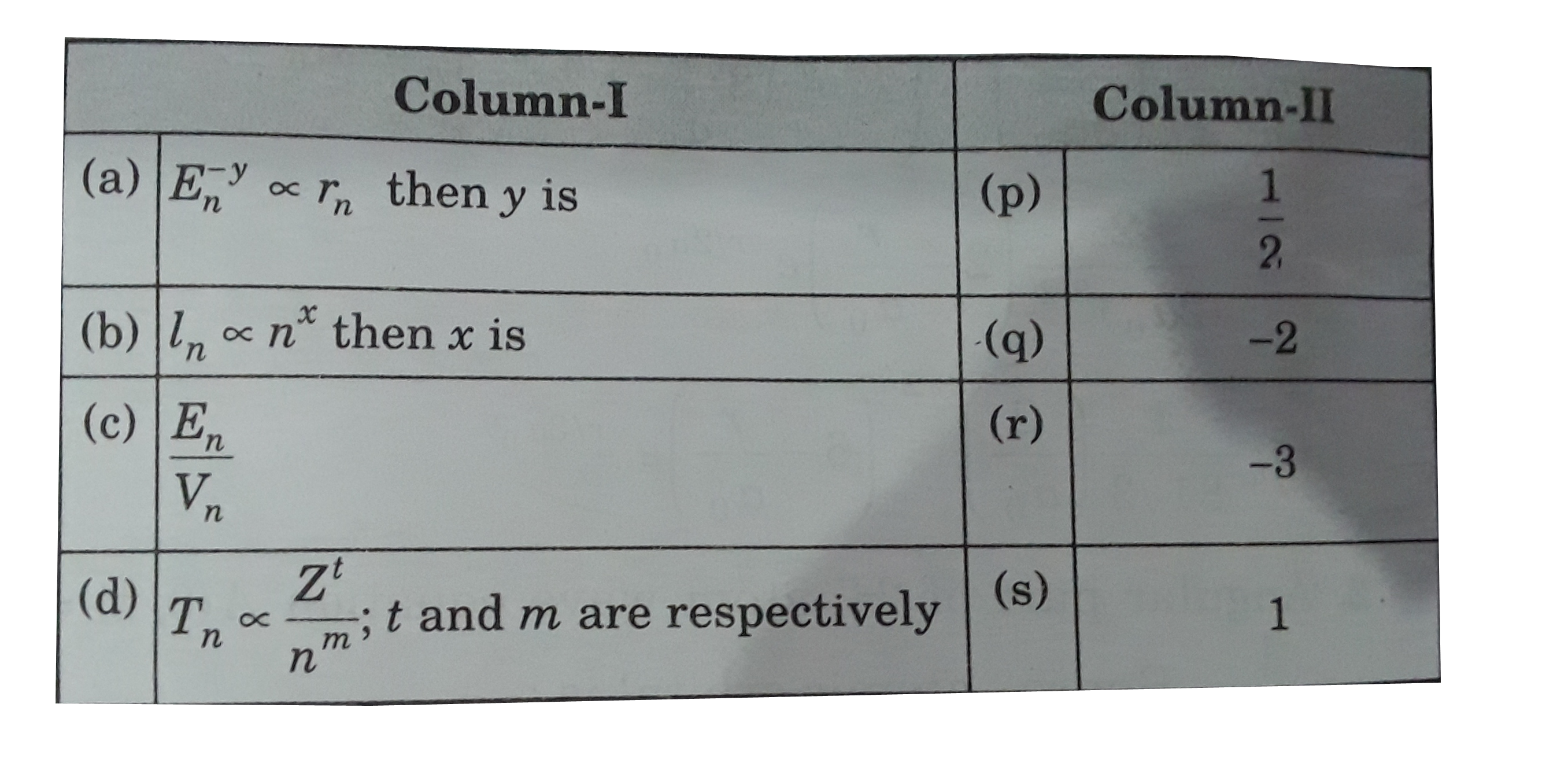
View SolutionEn= total energy , ln angular momentum
Kn=K.E.,Vn=P.E.,
Tn= time period , rn= radius of nth orbit
View SolutionWhen we write expression for energy of electron in nth orbit of hydrogen atom we take zero potential energy at n=∞, But potential energy depends on point in reference. If we take total energy of electron in n=1 orbit as zero then , match the following :

View SolutionPotential energy (PEn) and kinetic energy (KEn) of electron in nth orbit are related as
View SolutionAccording to Bohr's theory, the radius of the nth orbit of an atom of atomic number Z is proportional
View Solution
