A sphere can roll on a surface inclined at an angle θ if the friction coefficient is more than 17gtanθ. If a sphere is released from rest on the incline.
it will stay at rest
it will make pure translational motion
it will translate and rotate about the centre
the angular momentum of the sphere about its centre will remain constant
The correct Answer is:C
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISE - 1B51videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISE - 2106videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE99videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH SERIESCHAPTER - SURFACE TENSIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS )8videos
SURFACE TENSION
- BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) PRACTICE SHEET (ADVANCED) Integer/Subjective Type Questions2videos
THERMAL PROPERTIES OF MATTER
Similar Questions
A sphere can roll on a surface inclined at an angle θ if the friction coefficient is more than 27gsinθ. Suppose the friction coefficient is 17gsinθ, and a sphere is released from rest on the incline,
View Solution_E01_099_Q01.png)
A sylindrical vessel filled with water is released on an inclined surface of angle θ as shown in figure the friction coefficient of surface with vessel is μ(<tanθ) then the constant angle made by the surface of water with the incline will beView Solution
Knowledge Check
- Question 1 - Select One
A sphere can roll on a surface inclined at an angle θ if the friction coefficient is more than 27gsinθ. Suppose the friction coefficient is 17gsinθ, and a sphere is released from rest on the incline,
Ait will stay at restBit will translate and rotate about the centreCit will make pure translational motionDthe angular momentum of the sphere about its centre will remian constant. - Question 2 - Select One
A sphere can roll without slipping on a surface inclined at an angle θ if the fiiction coefficient is more than (2/7)g sin θ Suppose the friction coefficient is (l/7)g sin θ If a sphere is released from rest on this incline then which ofthe following are possible situations:
AIt will stay at restBItwill make pure translational motionCIt will translate and rotate about the centreDThe angular momentum of the spheres about its centre will remain constant - Question 3 - Select One
A sphere can roll on a surface inclined at an angle θ, if the friction coefficient μ>(2/7)gsinθ. Now suppose the friction coefficient is (1/7)gsinθ and the sphere is released from rest on the incline, then
Ait will stay at restBit will make pure translational motionCit will translate and rotate about the centreDthe angular momentum of he sphere about its centre will remains constant
Figure shows smooth inclined plane fixed in a car accelerating on a horizontal road. The angle of incline θ is related to the acceleration a of the car as a=gtanθ. If the sphere is set in pure rolling on the incline
View SolutionA plank of mass M is placed over smooth inclined plane and sphere is also placed over the plank. Friction is sufficient between sphere and plank. If plank and sphere are released from rest, the frictional force on sphere is -
.View SolutionA cylinderical vessel filled with water is released on a fixed inclined surface of angle θ as shown in figure. The friction coefficient of surface with vessel in μ(<tanθ). Then the constant with the incline will be (Neglect the viscosity of liquid)
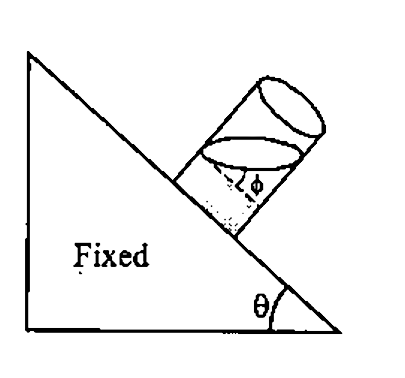View Solution-
A sylindrical vessel filled with water is released on an inclined surface of angle θ as shown in figure the friction coefficient of surface with vessel is μ(<tanθ) then the constant angle made by the surface of water with the incline will beView Solution A plank of mass M is placed over smooth inclined plane and sphere is also placed over the plank. Friction is sufficient between sphere and plank. If plank and sphere are released from rest, the frictional force on sphere is -
.View Solution