Six forces are acting on a particle. Angle between two adjacent force is 60∘. Five of the forces have magnitude F1 and the sixth has magnitude F2. The resultant of all the forces will have magnitude of Solution in Telugu
zero
F1+F2
F1−F2
F2
The correct Answer is:C
Step by step video, text & image solution for Six forces are acting on a particle. Angle between two adjacent force is 60^(@). Five of the forces have magnitude F_(1) and the sixth has magnitude F_(2). The resultant of all the forces will have magnitude of by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- VECTORSBOOK - AAKASH SERIESCHAPTER - VECTORSEXERCISE - Lecture Sheet (Exercise-I) (Addition of Vectors) (More than correct answer type Questions)
2 Videos
- VECTORSBOOK - AAKASH SERIESCHAPTER - VECTORSEXERCISE - Lecture Sheet (Exercise-I) (Integer Type Questions)
3 Videos
- UNITS AND MEASUREMENTS BOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTS EXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED))
14 Videos
- VISCOSITY BOOK - AAKASH SERIESCHAPTER - VISCOSITY EXERCISE - Questions for Descriptive Answers
3 Videos
Similar Questions
From figure the correct relation is
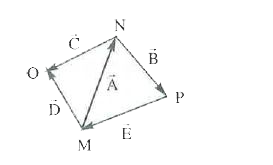
View SolutionFour forces of magnitudes P, 2P, 3p and 4P act along the four sides of a square ABCD in cyclic order. If the magnitude and direction of their resultant is n√2Pand15n find the value of n
View SolutionTwo clocks are being tested against a standard clock located in a national laboratory At 12 : 00 : 00 noon by standard clock, the readins of the two clocks are
DayClock - IClock - IIMonday12:00:0510:15:06Tuesday12:01:1510:14:59Wednesday11:59:0810:15:18Thursday12:01:5010:15:07Thursday12:01:5010:15:07Friday11:59:1510:14:53Saturday12:01:3010:15:24Sunday12:01:1910:15:11
From the Observations carefully read the following conclusive statements.
Statement I : Zero - error in clock II is much longer than zero - error in clock I.
Statement II : Zero - error can always be corrected by applying necessary corrective measures
Statement III : Precision of clock - I is more than precision of clock - II
Statement IV : Precision of clock - II is more than precision of clock - I
Pick up the correction option from the following.
View SolutionAn iron sphere of mass 20×10−3 kg falls through a viscous liquid with terminal velocity 0.5 m/sec. Find the terminal velocity of another iron sphere of mass 54×10−2 kg.
View SolutionTwo forces, each of magnitude F have a resultant of the same magnitude F. The angle between the two forces is
View SolutionTwo forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
View SolutionSix forces are acting on a particle. Angle between two adjacent force is 60∘. Five of the forces have magnitude F1 and the sixth has magnitude F2 . The resultant of all the forces will have magnitude of:
View SolutionTwo forces with equal magnitudes F act on body and the magnitude of the resultant forces is (F)/(3). The angle between the two forces is
View SolutionTwo forces each of magnitude 2N, act at an angle of 60∘. What is the magnitude of the resultant force ?
View Solutionयदि एक बिंदु कण पर कार्यरत दो बलों →P व →P के परिणामी बल का परिमाण ∣∣→P∣∣ है, तो दोनों बलों के बीच कोण होगा :
View SolutionIf two forces are acting at a point such that the magnitude of each force is 2N and the magnitude of their resultant is also 2N, then the angle between the two forces is
View Solutionयदि एक कण पर लगे परिमाण P तथा P√3 के दो बलों के परिणामी बल का परिमाण P हो, तो उनके बीच का कोण है
View SolutionTwo forces of equal magnitude act simultaneously on a partical. If the resultant of the forces is equal to the magnitude of each of then the angle between the forces is
View SolutionTwo forces of magnitude F having a resultant of the same magnitude of ,F the angle between the two forces is ______
View SolutionFive forces on a particle of m mass placed at the original point are superposed in such a way that they 72^(@) Is angled at an angle of. Magnitude of four forces F_(1) And the magnitude of a force F_(2) Is, the value of the resulting acceleration on the particle will be
View Solution
