When a coil joined to a cell, the current through the coil grows with a time constant . After what time, the current will reach 10% of its steady-state value ?
Given by
, applying log on both sides
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-IA
33 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-IB
18 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
32 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
20 Videos
Similar Questions
Knowledge Check
When a coil is joined to a cell, the current through the cell grows with a tiem constant τ. The current will reach 10% of its steady-state value of time
A0.1τBτIn(0.1)CτIn(0.9)DτIn(10−9)When a coil is joined to a cell, current in it grows with a time constant τ. The coil is disconnected from the cell before the current has reached its steady-state value, and, it is then short-circuited. The current will now decrease with a time constant
AτB>τC<τDeither (b) or (c) depending on the instant at which it was disconnected from the cellIn the circuit shown in fig.. Time constant and steady state current will be
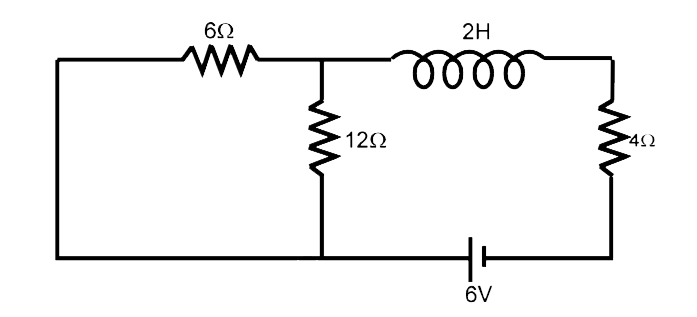 A0.25s,0.75AB0.75s,0.25AC0.25s,0.25AD0.5s,0.5A
A0.25s,0.75AB0.75s,0.25AC0.25s,0.25AD0.5s,0.5A
In the circuit shown in figure, time constant and steady state current will be
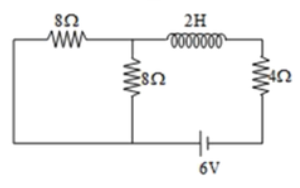
View SolutionHow many time constants one should wait for the current in an LR-circuit to grow within 0.1% of its steady state value.
View SolutionA coil of self-inductance 50 henry is joined to the terminals of a battery of e.m.f. 2 volts through a resistance of 10ohm and a steady current is flowing through the circuit. If the battery is now disconnected, the time in which the current will decay to 1/e of its steady value is
View SolutionA coil of inductance 300mH and resistance 2 Omega. The current reaches half of its steady state value in
View SolutionA coil of self-inductance 50 henry is joined to the terminals of a battery of e.m.f. 2 volts through a resistance of 10ohm and a steady current is flowing through the circuit. If the battery is now disconnected, the time in which the current will decay to 1/e of its steady value is
View Solution
