A uniform wire of length L us bent in the form of a circle. The shift in its centre of mass is
A
B
C
D
Text Solution
The correct Answer is:C
|
Topper's Solved these Questions
- CENTRE OF MASSBOOK - AAKASH SERIESCHAPTER - CENTRE OF MASSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - I (MAIN))
10 Videos
- CENTRE OF MASSBOOK - AAKASH SERIESCHAPTER - CENTRE OF MASSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) LECTURE SHEET (ADVANCED)
9 Videos
- CENTRE OF MASSBOOK - AAKASH SERIESCHAPTER - CENTRE OF MASSEXERCISE - PRACTICE SHEET (EXERCISE - II) LEVEL - I
2 Videos
- CALORIMETRY BOOK - AAKASH SERIESCHAPTER - CALORIMETRY EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) (LECTURE SHEET (ADVANCED)(Matrix Type Questions)
1 Videos
- COLLISIONS BOOK - AAKASH SERIESCHAPTER - COLLISIONS EXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
4 Videos
Similar Questions
Knowledge Check
A thick straight wire of length πm is fixed at its midpoint and then bent in the form of a cricle. The shift in its centre of mass is
AπmB0.5mC2mDπ2mA uniform wire of length l is bent into the shape of 'V' as shown. The distance of its centre of mass from the vertex A is :-
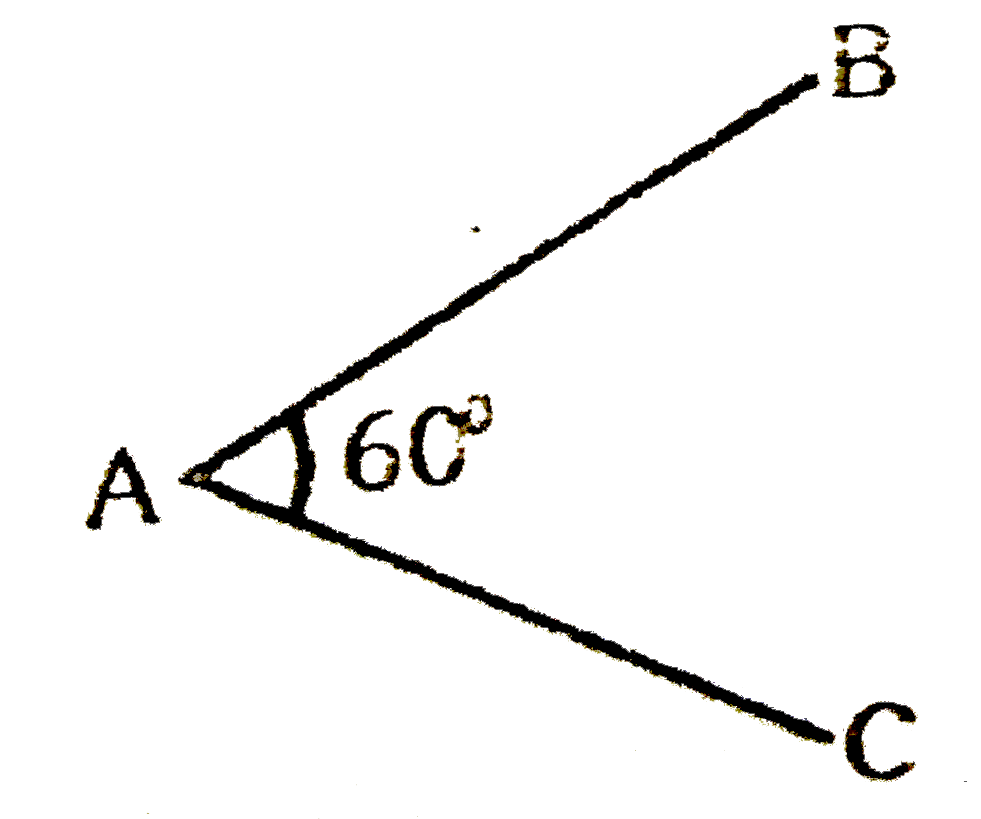 Al/2Bl√34Cl√38DNone of these
Al/2Bl√34Cl√38DNone of theseA thin wire of length l and mass m is bent in the form of a semicircle as shown in the figure. Its moment of inertia about an axis joining its free ends will be
 AMl22BMl2π2C2Ml2π2DMl22π2
AMl22BMl2π2C2Ml2π2DMl22π2
A uniform wire of resistance 36Ω is bent in the form of a circle. The Effective resistance between A and B is (O is the centre of circle):
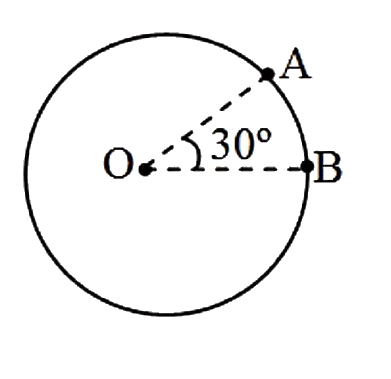
View SolutionA uniform wire of resistance 36Ω is bent in the form of a circle. The Effective resistance between A and B is (O is the centre of circle):

View SolutionA wire of length 56 m is bent in the form of a circle.Find the radius of the circle.Also find its area.
View SolutionThe wire of length L is bent in the form of square and circle.The ratio of their magnetic moment at their centre is
View SolutionA wire of length l and mass m is bent in the form of a semicircle. The gravitational field intensity at the centre of semicircle is

View Solution
