Two slabs A & B having lengths l1andl2, respectively, and same cross - section have thermal conductivities K1andK2 respectively. They are placed in contact and a constant temperature difference is maintained across the combination. The ratio of the quantities of heat flowing through A and B in a given time is
K1l1:K2l2
K1l2:K2l1
K1l1:K2l2
l:l
The correct Answer is:D
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - THERMOMETRY (LECTURE SHEET) Straight Objective Type Questions
15 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - THERMOMETRY (LECTURE SHEET) More than One correct answer Type Questions
5 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXERCISE-2
106 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE
99 Videos
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - EXERCISE - 3
33 Videos
Similar Questions
Two rods P and Q of equal lengths have thermal conductivities K1andK2 and cross-sectional areas A1andA2 respectively. If the temperature difference across the ends of each rod is the same, then the condition for which the rate of flow of heat through each of them will be equal, is
View SolutionTwo rods P and Q of equal lengths have thermal conductivities K1andK2 and cross-sectional areas A1andA2 respectively. If the temperature difference across the ends of each rod is the same, then the condition for which the rate of flow of heat through each of them will be equal, is
View Solution
Knowledge Check
Two identical rods with different thermal conductivities k1 & k2 and different temperatures are first placed along length and then along area, then the ratio of rates of heat flow in both cases is
A4k1k2(k1+k2)2BnoneC(k1+k2k1−k2)Dk1k2(k1+k2)2Two cylindrical rods of lengths l1 and l2, radii r1 and r2 have thermal conductivities k1 and k2 respectively. The ends of the rods are maintained at the same temperature difference. If l1=2l2 and r1=r2/2, the rates of heat flow in them will be the same if k1/k2 is:
A1B2C4D8A composite slab consists of two slabs A and B of different materials but of the same thickness placed one on top of the other. The thermal conductivities of A and B are k1 and K2 respectively. A steady temperature difference of 12∘C is maintained across the composite slab. If k1=K2/2, the temperature difference across slab A will be:
 A4∘CB8∘CC12∘CD16∘C
A4∘CB8∘CC12∘CD16∘C
Two slabs A and B of different materials but of the same thicknesss are joined end to end to form a composite slab. The thermal conductivities of A and B are K1 and K2 respectively. A steady temperature difference of 12∘C is maintained across the composite slab. If K1=K22, the temperature difference across slabs A is
View SolutionTwo rods with the same dimensions have thermal conductivities in the ratio 1 : 2. they are arranged between heat reservoirs with the same temperature difference, in two different configurations, A and B. The rates of heat flow in A and B are IAandIB respectively. The ratio IAIB) is equal to
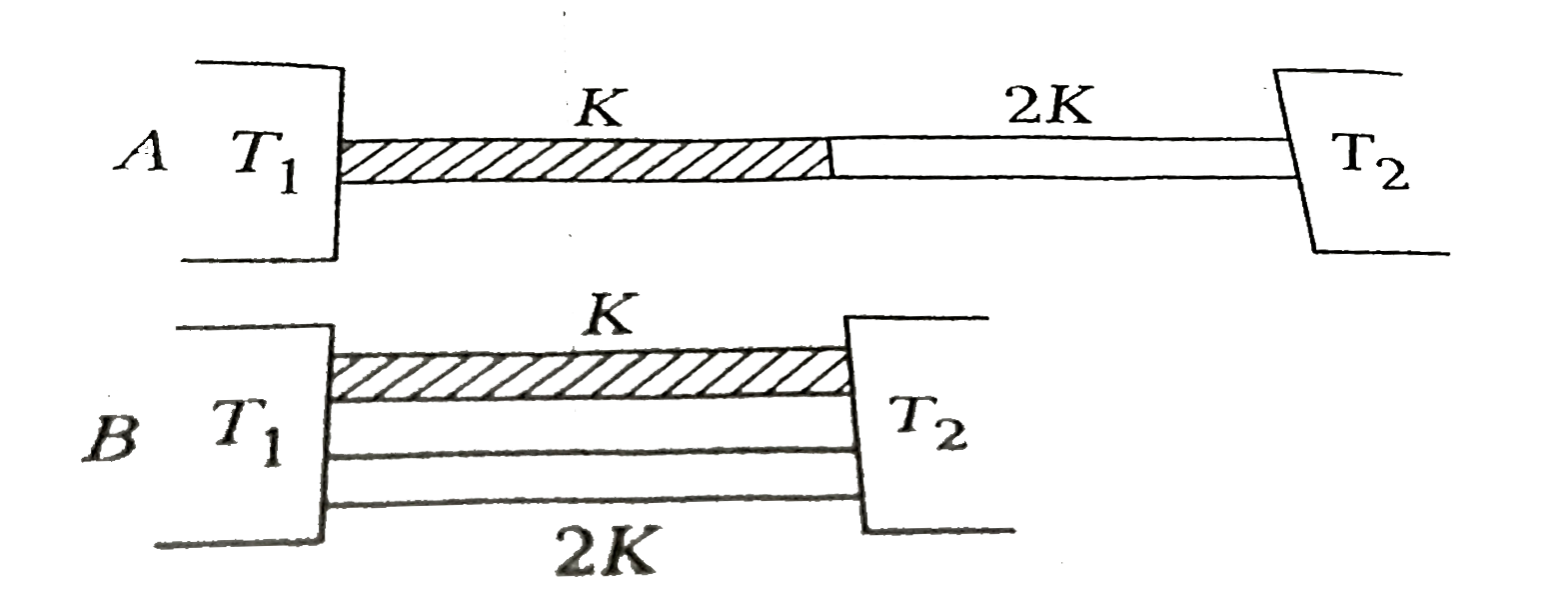
View SolutionTwo slabs A and B of different materials but of the same thickness are joined end to end to form a composite slab. The thermal conductivities of A and B are K1 and K2 respectively. A steady temperature difference of 12∘C is maintained across the composite slab. If K1=K22, the temperature difference across slab A is
View SolutionTwo rods of length l1andl2 and coefficients of thermal conductivities k1andK2 are kept touching each other. Both have the same area of cross-section. The equivalent of thermal conductivity is
View SolutionTwo rods with the same dimensions have thermal conductivities in the ratio 1 : 2. they are arranged between heat reservoirs with the same temperature difference, in two different configurations, A and B. The rates of heat flow in A and B are IAandIB respectively. The ratio IAIB) is equal to

View Solution
