The mass of a particle is 1kg and it is moving along x-axis. The period of its small oscillation is $\frac{π}{2}$ . Find its potential energy:

$- sin 2 x$

$- 16 sin x$

$- 16 cos x$

$- 4 cos 2 x$

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

The mass of particle is $1 k g$ it is moving along x- axis The period of its small osciltion is $\frac{π}{2}$ . Find the its potential energy:
A $- 4 sin 2 x$
B $- 16 sin x$
C $- 16 cos x$
D $- 4 cos 2 x$
The potential energy of a particle of mass 1 kg in motin along the x-axis is given by
U = 4(1-cos2x)J
Here, x is in meter. The period of small osciallationis (in second) is
A $2 π)$
B $π$
C $\frac{π}{2}$
D $\sqrt{2 π}$
A particle of mass $1 k g$ is moving X-axis. Its velocity is $6 m / s$ at $x = 0$ . Acceleration-displacement curve and potential energy-dispalcement curve of the particle are shown :
.
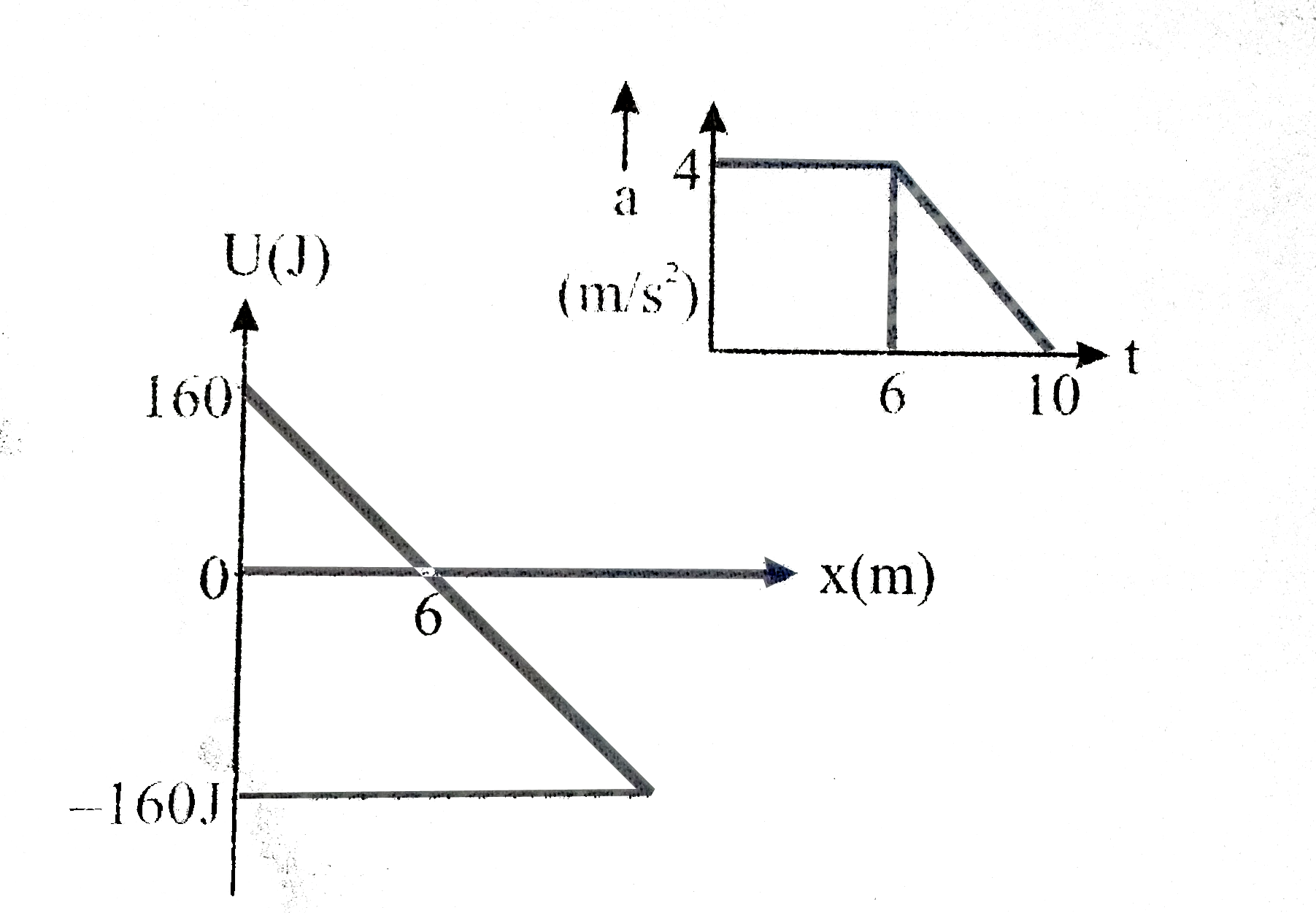Athe work done by all the forces is $704 J$
Bthe work done by external forces is $350 J$
Cthe work done by external forces is $384 J$
Dthe work done by conservation forces is $300 J$ .