Let x1,x2,x3 be the points where f(x)=|1−|x−4∣∣,x∈R is not differentiable then f(x1)+f(x2)+f(x3) =
Text Solution
The correct Answer is:1
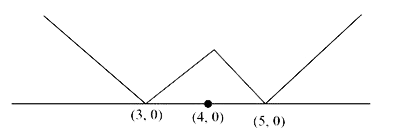
Function is not differentiable at x=3,4,5
So, f(3)+f(4)+f(5)
0+1+0=1
|
Similar Questions
Knowledge Check
Let f(x)=||x|−1|, then points where, f(x) is not differentiable is/are
A0,±1B±1C0D1The number of points of f(x)=|x-1|+|x-3|+sinx, x in [0,4), where f(x) is not differentiable, is
A0B1C2D3Let f:(−∞,∞)→(−∞,∞) be given by
f(x)=x|x|+|x−1|−|x−2|2+|x−3|3
If A denotes the set of points where f(.) is not differentiable, then A =A{3}B{1}C{0}D{2}
Let f(x)=||x|−1|, then points where, f(x) is not differentiable is/are
View SolutionLet f(x)=||x|−1|, then points where, f(x) is not differentiable is/are
View SolutionIf f(x)={x3,x2<1andx,x2≥1 then f(x) is differentiable at
View SolutionLet f(x)={2x+3 for x≤1 and ax2+bx for x>1 if f(x) is everywhere differentiable, prove that f(2)=−4.
View SolutionIf f(x)=|x+1|{|x|+|x−1|} then number of points of in [−2,2] where f(x) is differentiable is
View Solution
