Let f:[a,b]→R be a function such that , for c∈(a,b),f.(c)=f..(c)=f...(c)=f....(c)=f.....(c)=0. Then :
f has local extremum at x = c
f has neither local maximum nor local minimum at x = c
f is necessarily a constant function
It is difficult to say whether (A) or (B)
The correct Answer is:D
f.(C)=f..(C)=f...(C)=fiv(C)=fv(C)=0
Now if n is the least positive integer such that fn(C)≠0 , then it is not clear whether n is even or odd. So nothing can be said whether f(x) has local extrema at x= c or not .
Similar Questions
Lert f:[a,b]→ be a function such that for c∈(a,b),f′(c)=fii(c)=fiii(c)=fiv(c)=fv(c)=0. Then f (a)has a local extermum at x=⋅ f (b)has neither local maximum nor minimum at x=c f (c)is necessarily a constant function (d)it is difficult to say whether a)or(b).
View SolutionLert f:[a,b]→ be a function such that for c∈(a,b),f′(c)=fc=fc=fiv(c)=fv(c)=0. Then f (a)has a local extermum at x=⋅ f (b)has neither local maximum nor minimum at x=c f (c)is necessarily a constant function (d)it is difficult to say whether a)or(b).
View Solution
Knowledge Check
Let f:R→R be differentiable at c∈Randf(c)=0 . If g(x) = |f(x) |, then at x = c, g is
Anot differentiableBdifferentiable if f'(c)≠0Cnot differentiable if f'(c)=0Ddifferentiable if f'(c)=0Let f(x) be a polynomial function of second degree such that f(1) = f(-1). If a, b, c are in A.P., then f'(a), f'(b) and f'(c) are in :
AA.P.BG.P.CH.P.DA.G.P.Let f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
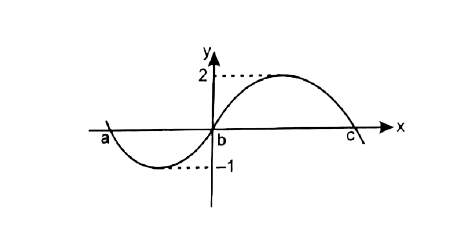 A∫caf(x)dx=∫cbf(x)dx+∫bcf(x)dxB∫caf(x)dx<aC∫baf(x)dx<∫bcf(x)dxD1b−a∫baf(x)dx>1c−b∫cbf(x)dx
A∫caf(x)dx=∫cbf(x)dx+∫bcf(x)dxB∫caf(x)dx<aC∫baf(x)dx<∫bcf(x)dxD1b−a∫baf(x)dx>1c−b∫cbf(x)dx
Let f:R→R be differentiable at c∈Randf(c)=0 . If g(x) = |f(x) |, then at x = c, g is
View SolutionLet f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
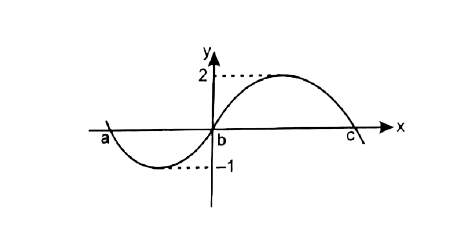
View SolutionLetfx) be a polynomial function of second degree. If f(1)=f(−1) and a, b,c are in A.P, the f'(a),f'(b)andf'(c) are in
View SolutionLet g(x) be the inverse of an invertible function f(x) which is differentiable at x=c . Then g′(f(x)) equal. (a)f′(c) (b) 1f′(c) (c) f(c) (d) none of these
View SolutionA function f(x) is differentiable at x=c(c∈R). Let g(x)=|f(x)|,f(c)=0 then
View Solution
