∠ABC and ∠ABD form a linear pair. If ∠ABC=30∘, then ∠ABD=...........
30∘
60∘
150∘
15∘
The correct Answer is:C
To solve the problem, we will use the property of linear pairs of angles. A linear pair of angles are two adjacent angles whose non-common sides form a straight line. This means that the sum of the angles in a linear pair is always equal to 180 degrees.
1. Identify the Given Information:
- We know that angle ABC and angle ABD form a linear pair.
- We are given that angle ABC = 30 degrees.
2. Use the Linear Pair Property:
- Since angle ABC and angle ABD form a linear pair, we can write the equation:
angle ABC+angle ABD=180∘
3. Substitute the Known Value:
- Substitute the value of angle ABC into the equation:
30∘+angle ABD=180∘
4. Solve for angle ABD:
- To find angle ABD, subtract 30 degrees from both sides of the equation:
angle ABD=180∘−30∘
- Calculate the right side:
angle ABD=150∘
5. Conclusion:
- Therefore, the measure of angle ABD is 150 degrees.
Final Answer:
angle ABD=150∘
- We know that angle ABC and angle ABD form a linear pair.
- We are given that angle ABC = 30 degrees.
- Since angle ABC and angle ABD form a linear pair, we can write the equation:
angle ABC+angle ABD=180∘
- Substitute the value of angle ABC into the equation:
30∘+angle ABD=180∘
- To find angle ABD, subtract 30 degrees from both sides of the equation:
angle ABD=180∘−30∘
- Calculate the right side:
angle ABD=150∘
- Therefore, the measure of angle ABD is 150 degrees.
angle ABD=150∘
Topper's Solved these Questions
- LINE AND ANGLESBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - LINE AND ANGLESEXERCISE - Skill Testing Exercise
21 Videos
- INTRODUCTION TO EUCLID.S GEOMETRYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - INTRODUCTION TO EUCLID.S GEOMETRYEXERCISE - MULTIPLE CHOICE QUESTIONS
3 Videos
- PROBABILITY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY EXERCISE - Multiple Choice Questions (MCQs)
10 Videos
Similar Questions
Knowledge Check
Angles x & y forms a linear pair and 2y−x=30∘, the value of y is
A70∘B110∘C210∘D60∘∠ABC is equal to
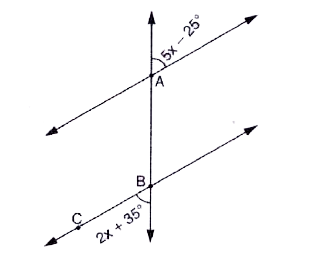 A75∘B105∘C115∘D85∘
A75∘B105∘C115∘D85∘In figure, if ∠AOB=90∘and∠ABC=30∘, then∠CAO is equal to
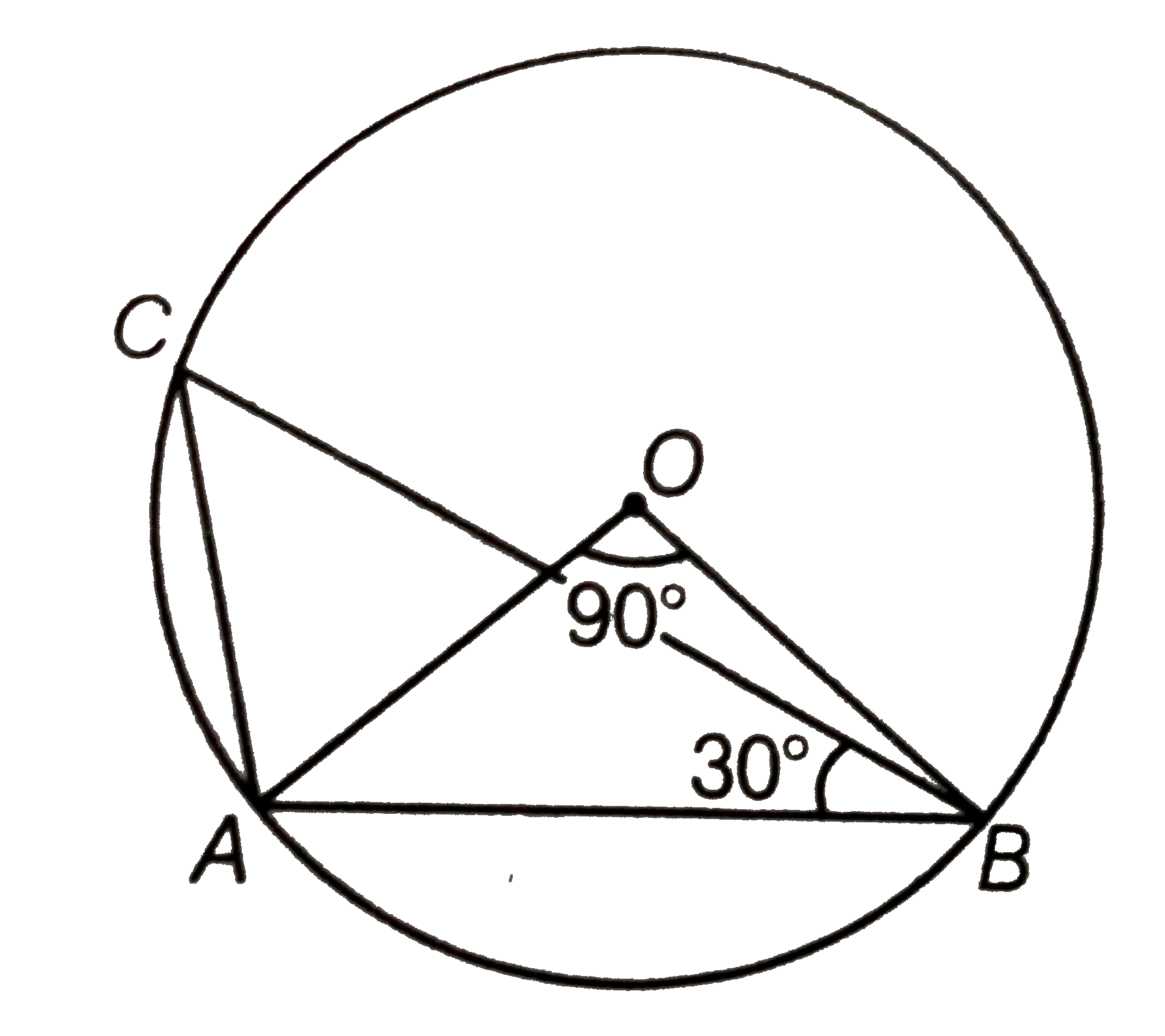 A30∘B45∘C90∘D60∘
A30∘B45∘C90∘D60∘
If ∠ABC=142∘ , find reflex ∠ABC.
View Solution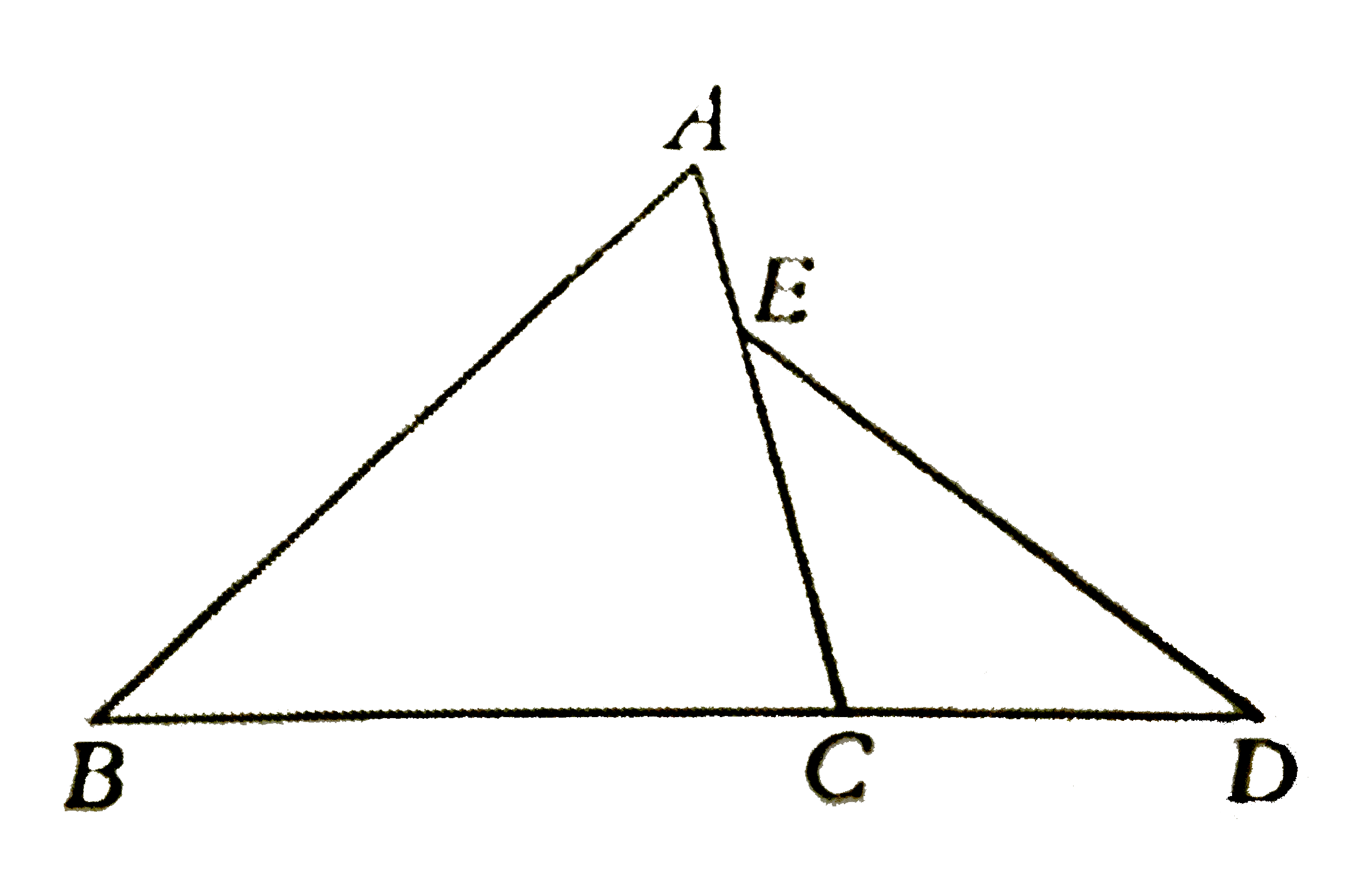
In the figure above, BC=AC, CD=CE. If ∠ABC=50∘, then find ∠CED.
View SolutionABCD is cylic if ∠ABC=50∘ and ∠DBC=60∘ then find ∠BCD
View SolutionIf figure, if∠ABC=20∘,then∠AOC is equal to
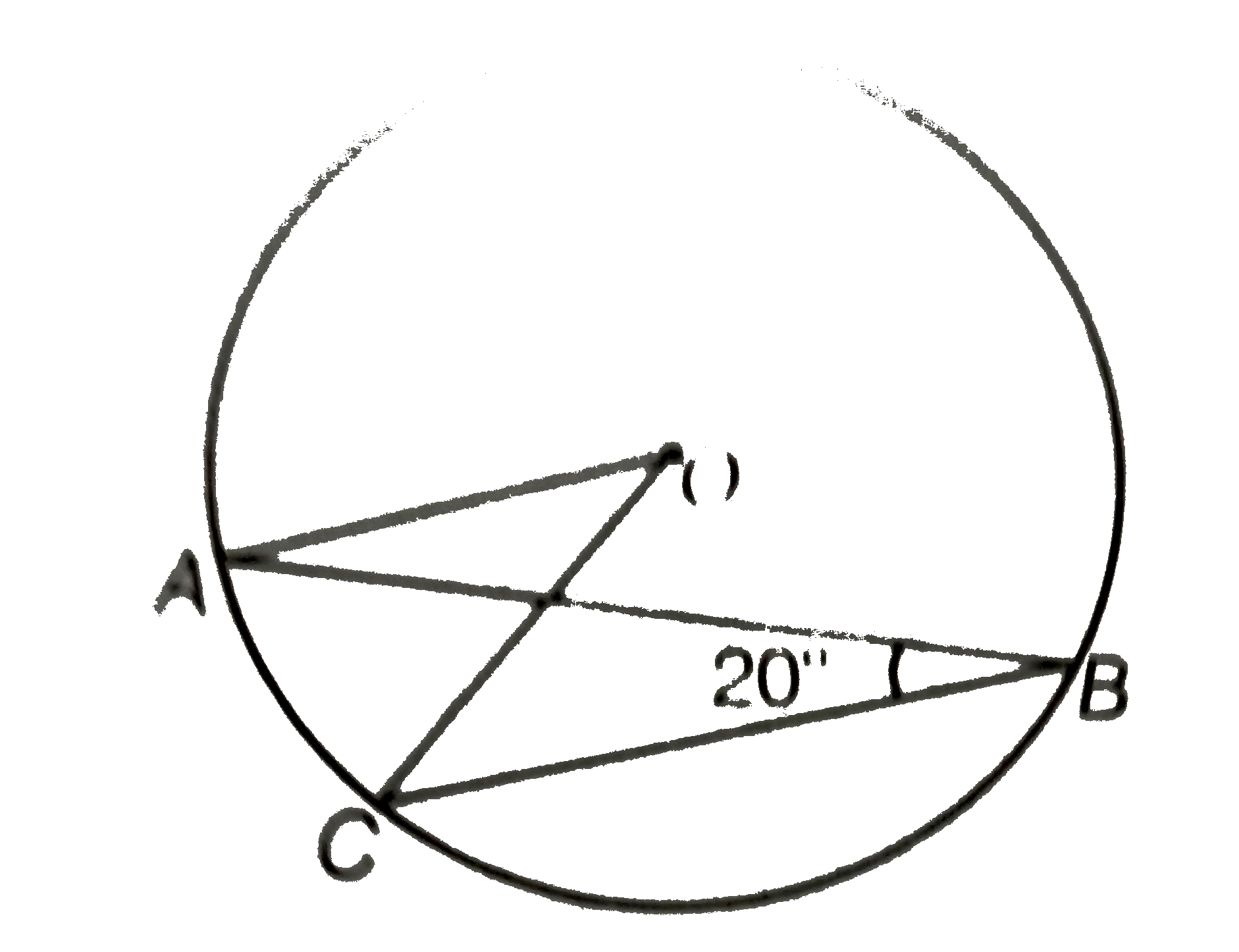
View Solution
In the above figure, ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯DE and ACE is a straight line . I f ∠ABC=30∘ and ∠CDE=20∘, then find ∠BCD.
View Solution
