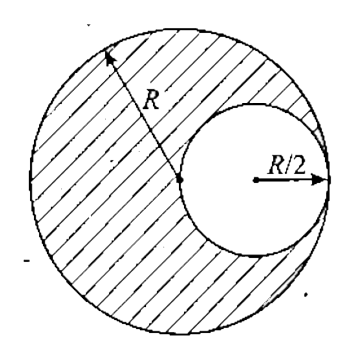
A uniform disc of diameter R/2 is put over another uniform disc of radius R of same thickness and density. The peripheries of the two discs touch each other. Locate the centre of mass of the system.
The correct Answer is:3R68 from the centre of larger disc towards the centre of the smaller disc
Similar Questions
A uniform disc of radius R is put over another uniform disc of radius 2R of the same thickness and density. The peripheries of the two discs touch each other. Locate the centre of mass of the system.
View SolutionA uniform disc of radius R is put over another unifrom disc of radius 2R of the same thickness and density. The peripheries of the two discs touch each other. Locate the centre of mass of the system.
View Solution
Knowledge Check
A uniform disc of mass m and diameter 2R moves forward towards another uniform disc of mass 2m & diameter 2R on a frictionless surface as shown in figure

When the first disc contacts the second, they stick to each other and move as a single object.Athe velocity of combined disc after the collision is V03Bthe angular velocity of combined disc after the collision is ω23CIf ω0=83V0R, the combined disc will not rotateDIf combined disc does not rotate, the energy loss is 199MV20
A disc of radis R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disc.
View SolutionA disc of radis R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disc.
View SolutionFrom a uniform disc of radius R, a circular section of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of mass of the resulting flat body.
View SolutionFrom a uniform disc of radius R, a circular section of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of mass of the resulting flat body.
View SolutionFrom a uniform disc of radius R, a circular section of radius R/2 is cut out. The centre of the hole is at R/2 from the centre of the original disc. Locate the centre of mass of the resulating flat body.
View SolutionA circular section of radius r si cut out of a uniform disc of radius R, the centre of the hole being R/2 from the centre of the original disc. Locate the centre of gravity of the remainder.
View SolutionFigure-4.11 shows a circular a disc of radius R from which a small disc is cut such that the periphery of the small disc touch the large disc and whose radius is R/2. Find the centre of mass of the remaining part of the disc.
View Solution
