A planet revolves around sun in an elliptical orbit of eccentricity .e.. If .T. is the time period of the planet then show that the time spent by the planet between the end of the minor axis and close to sun is $T (\frac{1}{4} - \frac{e}{2 π})$

Text Solution

Verified by Experts

$\frac{d A}{d t}$ =constant ,

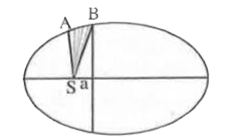
$\frac{t_{A B}}{T} = \frac{(Area) SAB}{(Area) ellipse} = \frac{\frac{π a b}{4} - \frac{1}{2} b (e a)}{π a b}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A planet revolves around the sun in elliptical orbit of eccentricity 'e'. If 'T' is the time period of the planet then the time spent by the planet between the end of the minor axis and close to the sun is
A $T (\frac{1}{4} - \frac{e}{2 π})$
B $\frac{T e}{π}$
C $(\frac{e}{π} - 1)$
D $\frac{π T}{e}$
Question 2 - Select One
A planet revolves around the sun in elliptical orbit of eccentricity 'e'. If 'T' is the time period of the planet then the time spent by the planet between the ends of the minor axis and major axis close to the sun is
A $\frac{T π}{2 e}$
B $T (\frac{2 e}{π} - 1)$
C $\frac{T e}{2 π}$
D $T (\frac{1}{4} - \frac{e}{2 π})$
Question 3 - Select One
A planet is revolving round the sun in an elliptical orbit, If v is the velocity of the planet when its position vector from the sun is r, then areal velocity of the planet is
A $| v \times r |$
B $2 | r \times v |$
C $∣ ∣ ∣ \frac{1}{2} (r \times v) ∣ ∣ ∣$
DNone of these

AAKASH SERIES-GRAVITATION-ADDITIONAL PRACTICE EXERCISE (LEVEL-II) PRACTICE SHEET (ADVANCED)Integer Type Questions

Home
Ask Doubt
Profile