If a simple pendulum has significant amplitude (up to a factor of 1/e of original) only in the period between t = 0 s to t = T sec, then T may be called the average life of the pendulum. When the spherical bob of the pendulum of mass 1kg suffers a retardation (due to viscous drag) proportional to into velocity with .b. as the constant of proportionality, the average life time of pendulum is (assuming damping is small) in seconds,
0.693b
b
1b
2b
The correct Answer is:D
Topper's Solved these Questions
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - GRAVITATION (KEPLER.S LAWS)
9 Videos
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - GRAVITATION (UNIVERSAL LAW OF GRAVITATION)
8 Videos
- APPENDICES BOOK - AAKASH SERIESCHAPTER - APPENDICES EXERCISE - OSCILLATIONS (SPRINGS)
18 Videos
- CALORIMETRY BOOK - AAKASH SERIESCHAPTER - CALORIMETRY EXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) (LECTURE SHEET (ADVANCED)(Matrix Type Questions)
1 Videos
Similar Questions
If a simple pendulum has significant amplitude (up to a factor of 1e of orginal) only in the period between t=0 sec to t=τ sec, then τ may be called the average life of pendulum. When the spherical bob of the pendulum suffers a retardation (due to viscous drag) proportional to its velocity, with 'b' as the constant of proportionality, the average life time of the pendulum is (assuming damping is small) in seconds:
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View Solution
Knowledge Check
If a simple pendulum has significant amplitude (up to a factor of 1/e of original) only in the period between t = Os to t=τs, then τ may be called the average life of the pendulum. When the spherical bob of the pendulum suffers a retardation (due to viscous drag) proportional to its velocity, with 'b' as the constant of proportionality, the average life time of the pendulum is (assuming damping is small) in seconds:
A(a) (0.693)/bB(b) bC(c )1bD(d) 2bA simple pendulum is set into vibrations. The bob of the pendulum comes to rest after some time due t
AAir frictionBMoment of inertiaCweight of the bobDCombination of all the aboveA simple pendulum has a time period T. If the support and the pendulum fall freely, the time period will be
AinfinityBgreater than TCless than TDzero
If T and T' are the periods of a simple pendulum and a conical pendulum of the sample length then .
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View SolutionIf the time period of a pendulum is 1sec, then what is the length of the pendulum at point of intersection of l−T and l−T2 graph.
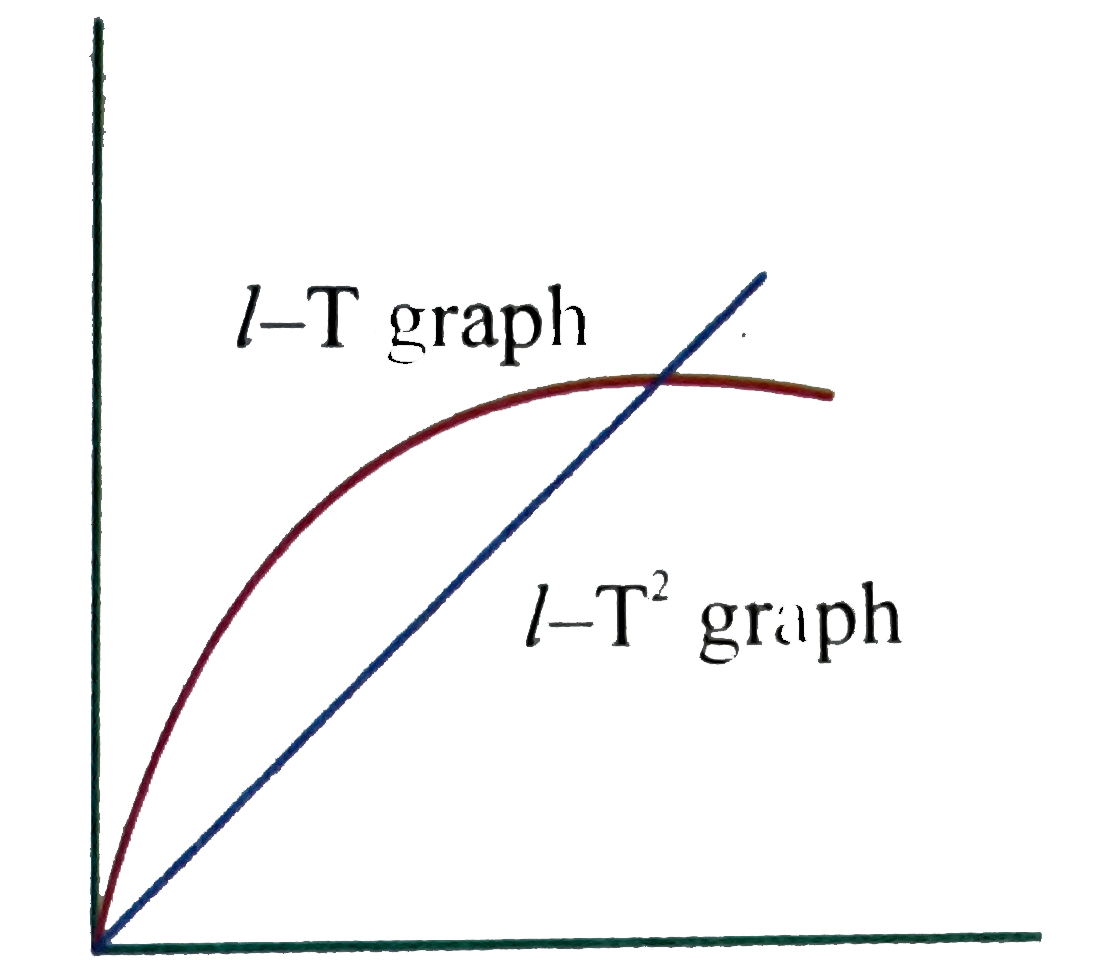
View SolutionA simple pendulum performs SHM about x=0 an amplitude a and time period T. the speed of pendulum at x=a2 will be
View SolutionA simple pendulum performs simple harmonic motion about x=0 with an amplitude a and time period T speed of the pendulum at x=a/2 will be
View Solution
