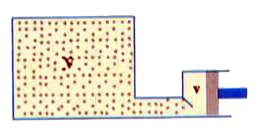
A gas is enclosed in a vessel of volume V at a pressure P. It is being pumped out of the vessel by means of a piston-pump with a stroke volume v. What is the final pressure in the vessel after n strokes of the pump ? Assume temperature remains constant.

Text Solution

Verified by Experts

According to ideal gas equation PV = $μ$ RT, at constant temperature for a given mass, $P V = P^{1} V^{1}$
Now as stroke volume is vm during 1st stroke for constant mass (say m) volume changes from V to (V + v) and so if pressure changes from P to $P_{1}$ , the above equation yields
$P V = P_{1} (V + v), i.e., P_{1} = P [\frac{V}{V + v}] ....(1)$
After the first stroke the gas left in the vessel has again volume V but at pressure $P_{1}$ (with mass $m_{1} < m$ ).
Now the second stroke will take place from these initial conditions and if $P_{2}$ is the pressure of the gas in the cylinder at the end of 2nd stroke,
$P_{1} V = P_{2} (V + v), i.e., P_{2} = P_{1} [\frac{V}{V + v}]$
Substituting the value of $P_{1}$ from equation (1) in the above $P_{2} = P {[\frac{V}{V + v}]}^{2}$
Repeating the same for n stroks, the pressure of the gas in the vessel after $n^{t h}$ stroke will be,
$P_{n} = P {[\frac{V}{V + v}]}^{n} = P {[\frac{1}{1 + (v / V)}]}^{n}$ .

Updated on:21/07/2023