Vector →A s 2cm long and is 60∘ above the x-axis in the first quadrant, vector →B is 2 cm long and is 60∘ below the x-axis is the fourth quadrant. Find →A+→B.
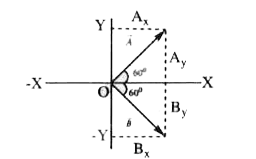
→R=→A+→B
→R=2cos60∘ˆi+2sin60ˆj+2cos60ˆi−2sin60ˆj
→R=4cos60ˆi=2ˆi
∴2cm, along x-axis
Topper's Solved these Questions
- MOTION IN A PLANE BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Exercise -I
91 Videos
- MOTION IN A PLANE BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Exercise -II
135 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE - 3
26 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Questions for Descriptive Answers
7 Videos
Similar Questions
Vector →A is 2cm long and is 60∘ above the x-axis in the first quadrant. Vactor →B is 2cm long and is 60∘ below the x-axis in the fourth quadrant. The sum →A+→B is a vector of magnitudes
View SolutionVector →A is 2 cm long and is 60° above the x - axis in the first quadrant, vector →B is 2cm long and is 60° below the x - axis in the fourth quadrant. Find →A+→B
View Solution
Knowledge Check
Vector →A is 2cm long and is 60∘ above the x-axis in the first quadrant. Vector →B is 2cm long and is 60∘ below the x-axis in the fourth quadrant. The sum →A+→B is a vector of magnitudes
A2cm along positive y-axisB2cm along positive x-axisC2cm along negative y-axisD2cm along negative x-axisA vector →A is along the positive x-axis and its vector product with another vector →B is zero, then vector →B could be
Aˆi+ˆjB4ˆiCˆj+ˆkD−7ˆkA vector →A is along the positive x- axis . If B is another vector such that →A×→B is zero , then B could be
A4jB−4iC−(i+j)D(j+k)
→Aand→B are two vectors such that →A is 2 m long and is 60∘ above the x - axis in the first quadrant →B is 2 m long and is 60∘ below the x - axis in the fourth quadrant .
Draw diagram to show →a+→B,→A−→Band→B−→A
(b) What is the magnitude and direction of →A+→B,→A−→Band→B−→A
View SolutionThe angle between vectors →Aand→B is 60∘ What is the ratio →A.→B and ∣∣→A×→B∣∣
View SolutionA vector →r is inclined to x-axis at 450 and y-axis at 600. If |→r|=8 units, find →r.
View SolutionA vector →r is inclined to x-axis at 450 and y-axis at 600. If |→r|=8 units, find →r.
View SolutionThe angle between vectors →Aand→B is 60∘ What is the ratio →A.→B and ∣∣→A×→B∣∣
View Solution
