A smll block of mass m slides along a smooth track as shown in the fig.
(i) If it starts from rest at P, what is the resultant force acting on it at Q?
(ii) At what height above the bottom of the loop should the block be released so that the force it exerts against the track at the top of the loop equals its weight?
By conservation of mechanical energy between points P and Q.
mg(5R)=mgR+12mv2
Now is case of circular motion,
N(or T) =mv2R+mgcosθ
And as at Q, θ=90∘

N=mv2R=m(8gR)R=8mg
So resultant force on m at Q.
F=√(8mg)2+(mg)2=(√65)mg (ii) At highest point
N=mv2R−mg
(asθ=180∘)
But according to given problem N = mg
somv2R=mg+mg,i.e,v=√2gR
If for achieving it h the height, by conservation of mechanical energy again
mgh.=12mv2+mg(2R)
orh.=2R+v22g=2R+2gR2g=3R
Topper's Solved these Questions
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - EXERCISE - I
78 Videos
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - EXERCISE - II (SCALARS (OR) DOT PRODUCT)
9 Videos
- WORK POWER ENERGYBOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 3
61 Videos
Similar Questions
A small block of mass m slides along a smooth track, as shown in fig. (i) If it starts from rest at P, what is the resulting force acting on it at Q ? (ii) at what height above the bottom of the loop should the block be released so that the force it exerts against the track at the top of the loop equals its weight?
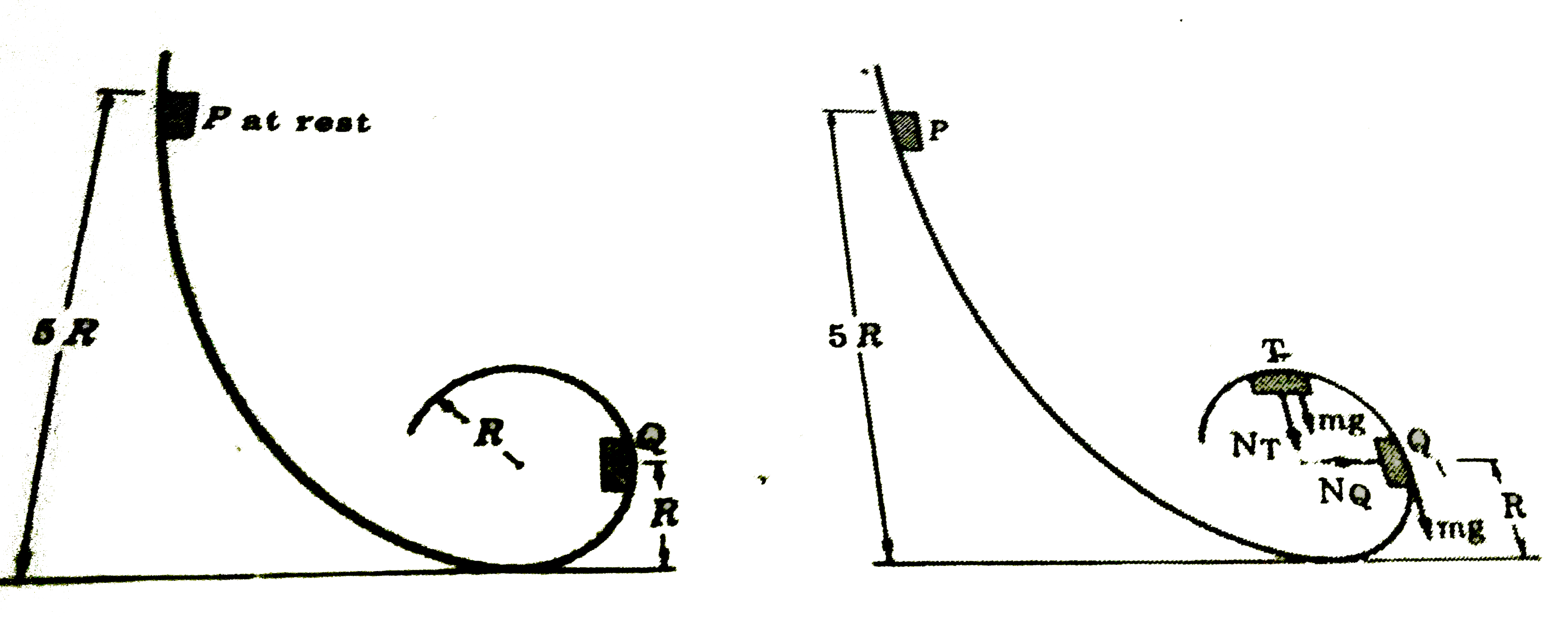
View SolutionA small block of mass m slides along a smooth frictionless track as shown.
(a) If it starts from rest at P, what is the resultant force acting on it at Q?
(b) At what height above the bottom should the block be released so that the force it exerts against the track at the top of the loop equals its weight?

View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
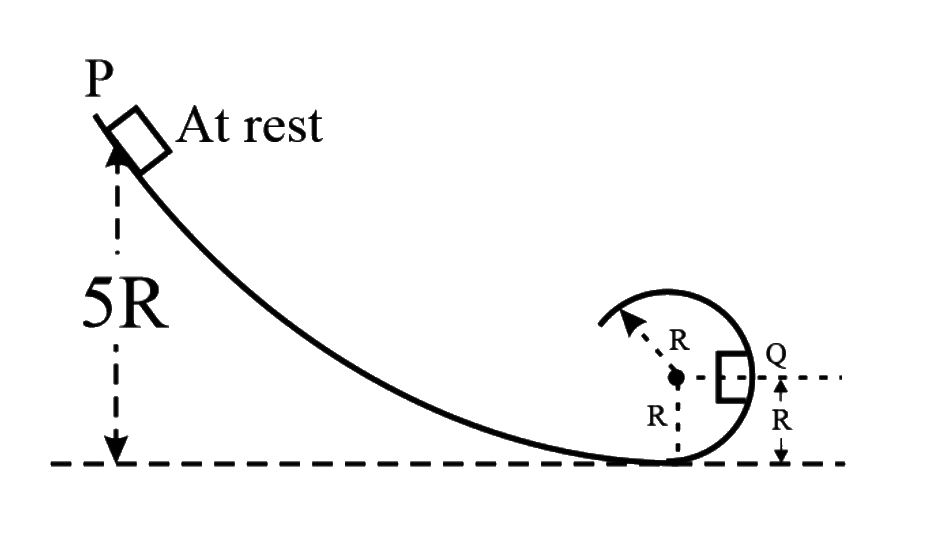
View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?

View SolutionA block of mass m slides along a frictionless surfce as shown in the figure. If it is releases from rest t A what is its speed at B?
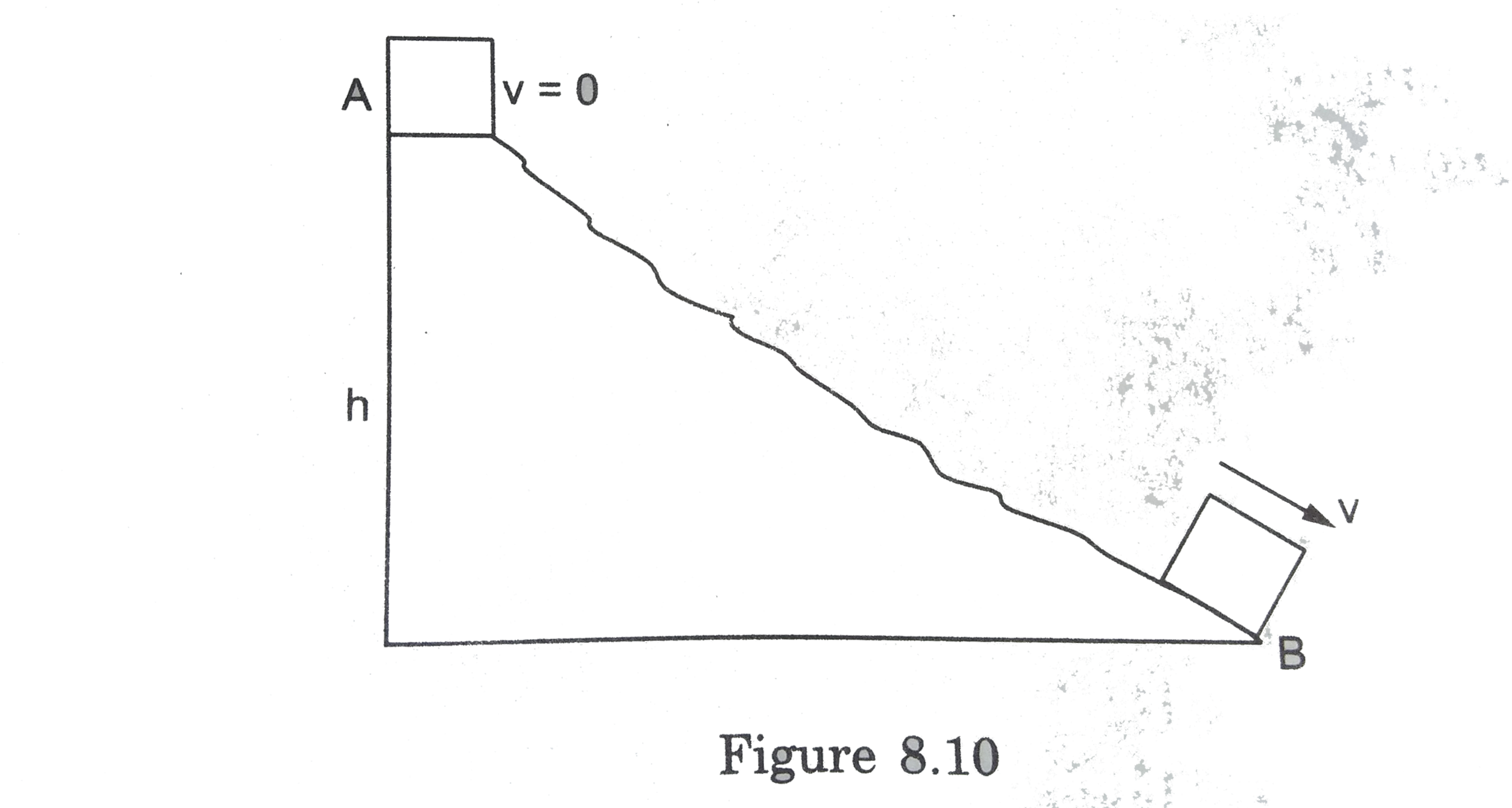
View SolutionA block of mass m slides along a frictionless surface as shown in the figure. If it is releases from rest from A what is its speed at B?

View SolutionA block of mass m is released along a smooth track shown in figure from its top point. Find the word done by gravity during its downward motion to the bottom of track.
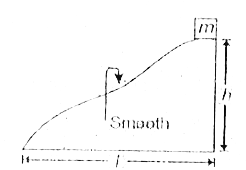
View SolutionA block of mass m is released along a smooth track shown in figure from its top point. Find the word done by gravity during its downward motion to the bottom of track.

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View Solution
