A particle A approach relative to a particle B with a velocity of 20m/s. If they collide head on and the coefficient of restitution is 1/4, the velocity with which A moves away from B after impact is Solution in Telugu
10m/s
15 m/s
5 m/s
2.5m/s
The correct Answer is:C
Step by step video, text & image solution for A particle A approach relative to a particle B with a velocity of 20m/s. If they collide head on and the coefficient of restitution is 1/4, the velocity with which A moves away from B after impact is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - PRACTICE EXERCISE (PERFECTLY INELASTIC COLLISIONS)5videos
WORK, POWER & ENERGY
- BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - PRACTICE EXERCISE (ELASTIC COLLISIONS)8videos
WORK, POWER & ENERGY
- BOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 361videos
WORK POWER ENERGY
Similar Questions
A shot moving with a velocity 140ms−1 collides a wooden block and comes to rest in it. If mass of the block is 13 times the mass of the shot, velocity of the block is
07:27View SolutionA particle of mass m moving with velocity u collides elastically with a particle of mass 3m at rest. After collision the heavier particle moves with velocity.
02:02View SolutionIf →A+→B=→Rand2→A+→B is perpendicular to →B then
04:30View SolutionA particle of mass 2kg moving with a velocity 5hatim//s collides head-on with another particle of mass 3kg moving with a velocity -2hatim//s . After the collision the first particle has speed of 1.6m//s in negative x-direction, Find (a) velocity of the centre of mass after the collision, (b) velocity of the second particle after the collision. (c) coefficient of restitution.
09:06View SolutionA particle moving with velocity (2hati-3hatj) m/s collides with a surface at rest in xz–plane as shown in figure and moves with velocity (2hati+2hatj) m/s after collision. Then coefficient of restitution is :
09:19View SolutionA 1 kg ball moving at 12 m//s collides head on with a 2g ball moving in the opposite direction at 24 m//s . The velocity of each ball after the impact, if the coefficient of restitution is 2//3 is
06:26View SolutionTwo particles A and B of mass 3kg and 4kg moving on a smooth horizontal surface with speed 5m//s and 2m//s respectively collides each other. Coefficient of restitution is e=(1)/(2) , then Velocity of A after collision
04:01View SolutionTwo particles A and B of mass 3kg and 4kg moving on a smooth horizontal surface with speed 5m/s and 2m/s respectively collides each other. Coefficient of restitution is e=12, then
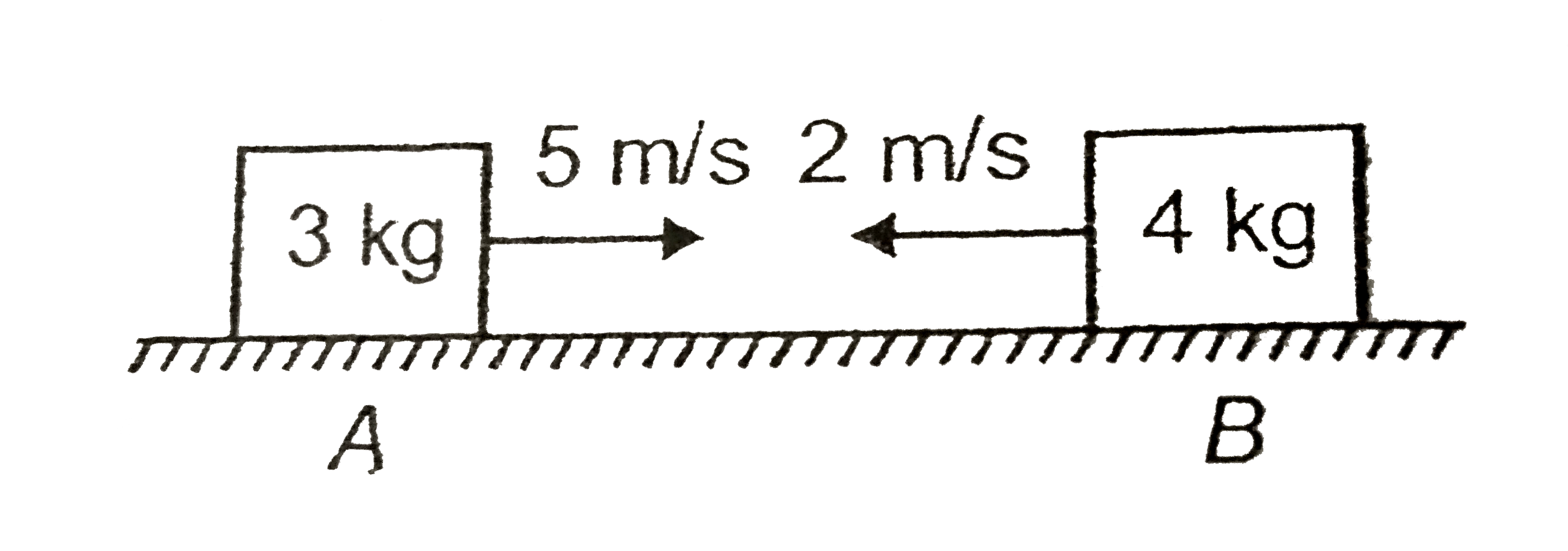
Velocity of B after collision04:01View Solution2kg ball moving at 6ms^(-1) collides head on with a 3kg ball moving in the opposite direction at 12m/s .The velocity of each ball after the impact, if the coefficient of restitution is 1/2, is ?
05:39View SolutionA 1kg ball moving at 12ms−1 collides head on with a 2kg ball moving in the opposite direction at 24 m/s. The velocity of each ball after the impact, if the coefficient of restitution is 2/3, is
View SolutionA 1kg ball moving at 12 ms^(-1) collides head on with a 2kg ball moving in the opposite direction at 24 m/s. The velocity of each ball after the impact, if the coefficient of restitution is 2/3, is
View SolutionA ball moving with velocity 2m/s collides head on with another stationary ball of double the mass . If coefficient of restitution is 0.5 then their velocities after collision will be ………. ms^(-1) .
04:45View SolutionA 1kg ball moving at 12ms^(-1) collides head on with a 2kg ball moving in the opposite direction at 24m/s. The velocity of each ball after the impact, if the coefficient of restitution is 2/3, is
06:02View SolutionA particle A approach relative to a particle B with a velocity of 20m/s. If they collide head on and the coefficient of restitution is 1/4, the velocity with which A moves away from B after impact is
09:49View SolutionA particle of mass 2kg moving with a velocity 5ˆim/s collides head-on with another particle of mass 3kg moving with a velocity −2ˆim/s. After the collision the first particle has speed of 1.6m/s in negative x-direction, Find
(a) velocity of the centre of mass after the collision,
(b) velocity of the second particle after the collision.
(c) coefficient of restitution.04:12View Solution

