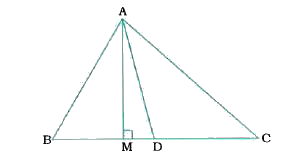
In the given figure, AD is a median of a DeltaABC and $A M ⊥ B C$ . Prove that :
$A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$
Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$

Answer

Step by step video, text & image solution for In the given figure, AD is a median of a DeltaABC and AM bot BC. Prove that : AC^(2)= AD^(2)+BC*DM+((BC)/(2))^(2) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

BOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - TEST YOUR SKILLS
29videos
TRIANGLES
BOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - PRACTICE THOROUGHLY
20videos
TRIANGLES
BOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.5
23videos
TRIANGLES
BOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - TEST YOUR SKILLS
5videos
SURFACE AREAS AND VOLUMES

In the given figure, AD is a median of a DeltaABC and $A M ⊥ B C$ . Prove that :
$A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$
Solution in Gujrati

The correct Answer is: $A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$

Topper's Solved these Questions

Similar Questions

KUMAR PRAKASHAN-TRIANGLES-EXERCISE 6.6

In the given figure, AD is a median of a DeltaABC and AM⊥BC. Prove that : AC2=AD2+BC⋅DM+(BC2)2 Solution in Gujrati

The correct Answer is:AC2=AD2+BC⋅DM+(BC2)2

Topper's Solved these Questions

Similar Questions

KUMAR PRAKASHAN-TRIANGLES-EXERCISE 6.6

In the given figure, AD is a median of a DeltaABC and $A M ⊥ B C$ . Prove that :
$A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$
Solution in Gujrati

The correct Answer is: $A C^{2} = A D^{2} + B C \cdot D M + {(\frac{B C}{2})}^{2}$