An aeroplane of mass M requires a speed v for take off. The length of runway is s and the coefficient of friction between the tyres and the ground is μ. Assuming that the plane accelerates uniformly during the take-off, the minimum force required by the engine of the plane for take off is:Solution in Telugu
M(v22s+μg)
M(v22s−μg)
M(2v2s+2μg)
M(2v2s−2μg)
The correct Answer is:A
Step by step video, text & image solution for An aeroplane of mass M requires a speed v for take off. The length of runway is s and the coefficient of friction between the tyres and the ground is mu. Assuming that the plane accelerates uniformly during the take-off, the minimum force required by the engine of the plane for take off is: by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - FRICTION (VERTICAL SURFACE)4videos
RIVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - FRICTION (INCLINED PLANE)30videos
RIVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - LAW OF MOTION (PSUEDO FORCE)12videos
RIVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE III64videos
RAY OPTICS
- BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - PRACTICE SHEET (ADVANCED) Integer Type Questions7videos
ROTATIONAL DYNAMICS
Similar Questions
A person stands in contact against a wall of cylindrical drum of radius r , rotating with an angular velocity ω . If is the coefficient of friction between the wall and the person, the minimum rotational speed which enables the person to remain stuck to the wall is
03:16View SolutionA block is pushed up a rough inclined plane of 45∘ with a velocity u. The block rises up and then comes down sliding to have a velocity u/2 at the bottom. The coefficient of sliding friction between block and the plane is
03:16View SolutionA body is placed on a smooth inclined plane of inclination 1 in x. The horizontal acceleration to be given to the inclined plane so that the body on it remains at rest with respect to inclined plane is
02:16View SolutionTwo plane mirrors are arranged at right angles to each other as shown in figure. A ray of light is incident on the horizontal mirror at an angle θ For what value of θ the ray emerges parallel to the incoming ray after reflection from the vertical mirror?
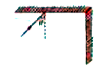 05:05View Solution
05:05View SolutionFour uniform solid spheres each of diameter √5 cm and mass 0.5 kg are placed with their centres at the corners of a square of side 4 cm. The moment of inertia of the system about the diagonal of the square is N×10−4kg m2, then the value of N is .
View SolutionAn aeroplane requires for take off a speed of 80km/h the run on the ground being 100 m The mass of aeroplane is 104 kg and ground is 0.2 What is the maximum force required by the engine of the plane for take off ?
02:35View SolutionAn aeroplane requires for take off a speed of 108 kmph the run on the ground being 100m mass of the plane is 104kg and the coefficinet of friction between the plane and the ground is 0.2. Assuming the plane accelerates uniformly the minimum force required is (g=10ms−2) .
View SolutionAn aeroplane needs to reach a speed of 324 km/hr before it can take off. It starts from rest and the engine of aeroplane produces a constant acceleration. If it spends 30 s on the runway then the minimum required runway length is -
02:35View SolutionAn aeroplane clears the runway path of 300m with a final take off speed of 234 kmph . If the total mass of the plane is 50000kg and friction between the wheels and the road is 0.4 , then calculate the thrust on the engine (g=10 ms^(-2)) .
02:48View SolutionAn aeroplane requires for take off a speed of 108 kmph the run on the ground being 100m. Mass of the plane is 104kg and the coefficient of friction between the plane and the ground is 0.2. Assuming the plane accelerates uniformly the minimum force required is (g=10ms−2)
View SolutionAn aeroplane of mass M requires a speed v for take off. The length of runway is s and the coefficient of friction between the tyres and the ground is mu . Assuming that the plane accelerates uniformly during the take-off, the minimum force required by the engine of the plane for take off is:
View SolutionAn aeroplane requires a speed of 72 kmph for a take off with 150m run on the ground. The mass of the plane is 1500kg and the coefficient of friction between the aeroplane and the ground is 0.4. Assuming that the aeroplane acceleration uniformly during take off, find the minimum force exerted by the engine of the aeroplane for take off.
View SolutionAn aeroplane of mass M requires a speed v for take off. The length of runway is s and the coefficient of friction between the tyres and the ground is μ. Assuming that the plane accelerates uniformly during the take-off, the minimum force required by the engine of the plane for take off is:
01:53View SolutionA jet plane starts from rest with an acceleration of 3 ms^(-2) and makes a run for 35 s before taking off. What is the minimum length of the runway and what is the velocity of the jet at take off ?
03:50View SolutionAn aeroplane requires a speed of 72kmph for a take off with 150m run on the ground. The mass of the plane is 1500kg and the coefficient of friction between the aeroplane and the ground is 0.4. Assuming that the aeroplane acceleration uniformly during take off, find the minimum force exerted by the engine of the aeroplane for take off.
04:11View Solution

