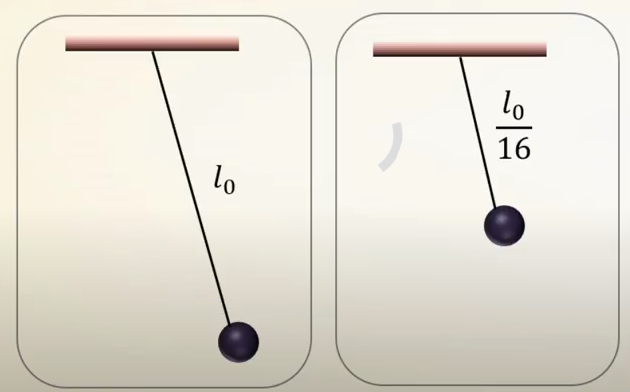
In a simple pendulum the period of oscillation (T ) is related to the length of the pendulum (L) as
LT=constant
L2T2=constant
LT2=constant
L2T=constant
The correct Answer is:C
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE -I Equation of S.H.M
51 Videos
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE -I Simple pendulum
24 Videos
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE II
175 Videos
- OSCILLATIONBOOK - AAKASH SERIESCHAPTER - OSCILLATIONEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
12 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
Similar Questions
Knowledge Check
When the length of a simple pendulum is decreased by 600 mm, the period of oscillation is halved. The original length of the pendulum was
A800 mmB1000 mmC1200 mmD240 mmConsider a simple pendulum. The period of oscillation of the simple pendulum depends on its length and acceleration due to gravity. Then the expression for its time period is
At=kg√lBt=k√lgCt=k√lgDt=kl√gThe time period of oscillation of a simple pendulum having length Land mass off the bob mis given as T. Ifthe length of the pendulum is increased to 4L and the mass of the bob is increased to 2m, then which one of the following is the new period of oscillation?
ATB2TC4TDT/2
A simple pendulum oscillating with a small amplitude has a time period of T=1.0s . A horizontal thin rod is now placed beneath the point of suspension at a distance equal to half the length of the pendulum. The string collides with the rod once in each oscillation and there is no loss of energy in such collisions. Find the new time period T of the pendulum
View SolutionThe time period of a simple pendulum is given by the formula, T=2π√l/g, where T = time period, l = length of pendulum and g = acceleration due to gravity.
If the length of the pendulum is decreased to 1/4 of its initial value, then what happens to its frequency of oscillations ?
View SolutionThe time period of a simple pendulum is given by the formula, T=2π√l/g, where T = time period, l = length of pendulum and g = acceleration due to gravity.
If the length of the pendulum is decreased to 1/4 of its initial value, then what happens to its frequency of oscillations ?
View SolutionAn amplitue of a simple pendulum of a period 'T' and length 'L' is increased by 5%. The new period of that pendulum will be
View SolutionA simple pendulum has time period T. A uniform rod, whose length is the same as that of the pendulum, undergoes small oscillations about its upper end. Its time period of oscillation will be -
View Solution