Water is falling down at the rate of 1.8 Kg per minute from a vertical tube. The radius at the bottom of the tube is 0.004 m and pressure around it is 0.76m of Hg. The diameter of the tube at a height of 0.5 m from the bottom is 0.005 m. find the pressure at that point.
m of Hg
0.824 m of Hg
0.924 m of Hg
0.624 m of Hg
The correct Answer is:A
Similar Questions
A vertical tube has diameter 0.016 m at its bottom end from which water flows out at the rate of 1.2 kg per minute. The pressure at the end is atmospheric pressure 0.7 m of mercury. If the diameter of the tube is 0.004 m at a height of 0.3 m from the bottom end, fidn the pressure there.
View SolutionA glass tube of 1.0m length is filled with water . The water can be drained out slowly at the bottom of the tube . A vibrating tuning fork of frequency 500Hz is brought at the upper end of the tube and the velocity of sound is 330m/s. Find the number of resonances that can be obtained.
View Solution
Knowledge Check
In a resonace tube, the first resonating length is 0.2 m and the second resonating length is 0.62 m from the open end. What is the inner diameter of the tube ?
A6.66 cmB4.67 cmC3.33 cmD2.33 cmA glass tube of 1.0m length is filled with water . The water can be drained out slowly at the bottom of the tube . If a vibrating tuning fork of frequency 500c/s is brought at the upper end of the tube and the velocity of sound is 330m/s, then the total number of resonances obtained will be
A4B3C2D1A glass tube of 1.0m length is filled with water . The water can be drained out slowly at the bottom of the tube . If a vibrating tuning fork of frequency 500Hz is brought at the upper end of the tube and the velocity of sound is 300m/s, then the total number of resonances obtained will be
AA. 4BB. 3CC. 2D4. 1
A glass tube of 1.0m length is filled with water . The water can be drained out slowly at the bottom of the tube . A vibrating tuning fork of frequency 500Hz is brought at the upper end of the tube and the velocity of sound is 330m/s. Find the number of resonances that can be obtained.
View SolutionA glass tube of 1.0m length is filled with water . The water can be drained out slowly at the bottom of the tube . If a vibrating tuning fork of frequency 500c/s is brought at the upper end of the tube and the velocity of sound is 330m/s, then the total number of resonances obtained will be
View SolutionAt a pressure of 3 atm air (treated as an ideal gas) is pumped into the tubes of a cycle rickshaw. The volume of each tube at given pressure is 0.004m3. One of the tubes gets punctured and the volume of the tube reduces to 0.0008m3. Find the number of moles of air that have leaked out? Assume that the temperature remains constant at 300K.(R=25/3Jmol−1K−1)
View SolutionAt a pressure of 3 atm air (treated as an ideal gas) is pumped into the tubes of a cycle rickwise. The volume of each tube at given pressure is 0.004m3. One of the tubes gets punctured and the volume of the tube reduces to 0.0008m3. Find the number of moles of air that have leaked out? Assume that the temperature remains constant at 300K.(R=25/3Jmol−1K−1)
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
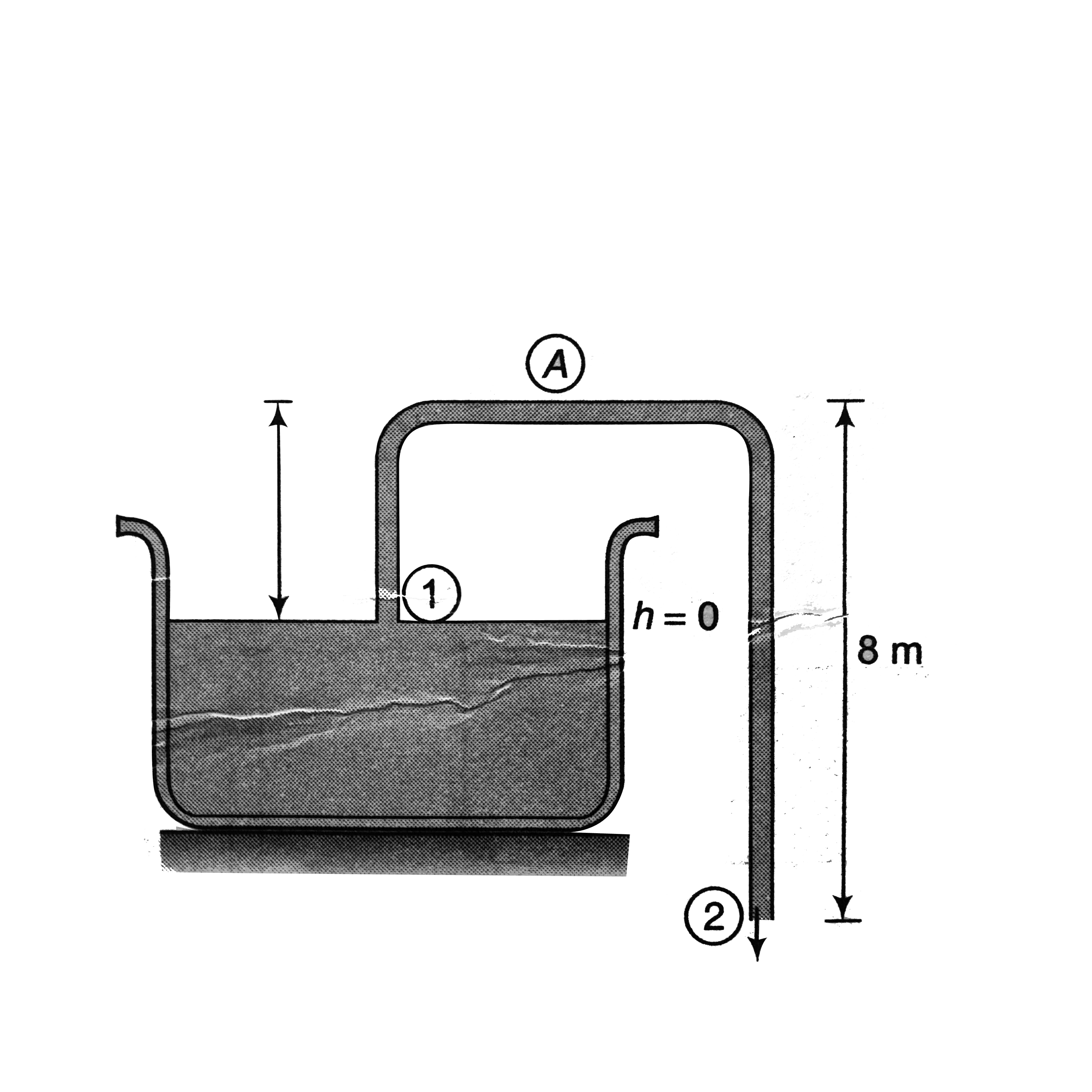 .
.
View Solution
