कथन-1 : उभयनिष्ठ अनुपात r1 के साथ a,b,c गु. श्रे. में तथा उभयनिष्ठ अनुपात r2 के साथ α,β,γ गु. श्रे. में है, यदि समीकरणों ax+αy+z=0,bx+βy+z=0,cx+γy+z=0 के केवल अशून्य हल है, तो r1≠r2,r1,r2≠1
कथन-2 : समीकरणों a1x+b1y+c1z=0,a2x+b2y+c2z=0,a3x+b3y+c3z=0 के केवल अशून्य हल है यदि ∣∣
∣∣a1b1c1a2b2c2a3b3c3∣∣
∣∣≠0
कथन-1 सही है, कथन-2 सही है, कथन-1 के लिए कथन-2 का स्पष्टीकरण सही है।
कथन-1 सही है, कथन-2 सही है, कथन-1 के लिए कथन-2 का स्पष्टीकरण नहीं सही है।
कथन-1 सही है, कथन-2 गलत है।
कथन-1 गलत है, कथन-2 सही है।
The correct Answer is:A
Step by step video, text & image solution for कथन-1 : उभयनिष्ठ अनुपात r_(1) के साथ a,b,c गु. श्रे. में तथा उभयनिष्ठ अनुपात r_(2) के साथ alpha,beta,gamma गु. श्रे. में है, यदि समीकरणों ax+alphay+z=0,bx+betay+z=0,cx+gammay+z=0 के केवल अशून्य हल है, तो r_(1)ner_(2),r_(1),r_(2)ne1 कथन-2 : समीकरणों a_(1)x+b_(1)y+c_(1)z=0,a_(2)x+b_(2)y+c_(2)z=0,a_(3)x+b_(3)y+c_(3)z=0 के केवल अशून्य हल है यदि |(a_(1),b_(1),c_(1)),(a_(2),b_(2),c_(2)),(a_(3),b_(3),c_(3))|ne0 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-I)6videos
सारणिक तथा आव्यूह
- BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - JEE ADVANCED MORE THAN ONE CORRECT ANSWERS (Comprehension type questions) (Passage-III)6videos
सारणिक तथा आव्यूह
- BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - EXERCISE262videos
सारणिक तथा आव्यूह
- BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)4videos
सरल रेखा
Similar Questions
माना कि p एक विषम अभाज्य संख्या है तथा Tp,2×2 आव्यूहों का निम्न समुच्चय है
Tp={A=[abca]:a,b,c∈{0,1,2,.............p−1}}
Tp में ऐसे A की संख्या जो सममित या विषम सममित अथवा दोनों प्रकार के हो एवं जिनके लिए det(A),p से विभाज्य है, निम्न है00:55View Solutionमाना A=⎡⎢⎣100210321⎤⎥⎦ तथा U1,U2 व U3,3×3 आव्यूह U के कॉलम है। यदि कॉलम आव्यूह U1,U2 तथा U3,AU1=⎡⎢⎣100⎤⎥⎦,AU2=⎡⎢⎣230⎤⎥⎦,AU3=⎡⎢⎣231⎤⎥⎦ को संतुष्ट करते है, तब निम्न प्रश्नो के उत्तर दीजिए
|U| का मान है00:54View Solution∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
06:14View Solution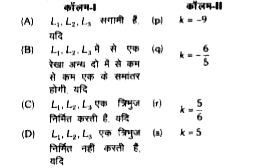
01:59View SolutionCosnsider the system of equation
a1x+b1y+c1z=0,a2x+b2y+c2z=0,
a3x+b3y+c3z=0 if ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=0, then the
system hasView SolutionConsider the system of equations
a1x+b1y+c1z=0
a2x+b2y+c2z=0
a3x+b3y+c3z=0
If ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=0, then the system hasView Solutionif x,y and z are not all zero and connected by the equations a1x+b1y+c1z=0,azx+b2y+c2z=0 and (p1+λq1)x+(p2+λq2)y+(p3+λq3)z=0 show that
λ=−∣∣ ∣ ∣∣a1b1c1a2b2c2p1p2p3∣∣ ∣ ∣∣÷∣∣ ∣ ∣∣a1b1c1a2b2c2q1q2q3∣∣ ∣ ∣∣05:05View Solutionयदि a1x+b1y+c1z+d1=0 तल और a2x+b2y+c2z+d2=0तल परस्पर लंब हैं, तो
View SolutionStatement -1 Consider the determinant
Δ=∣∣ ∣ ∣∣a1+b1x2a1x2+b1c1a2+b2x2a2x2+b2c2a3+b3x2a3x2+b3c3∣∣ ∣ ∣∣=0,
where ai,bi,ci∈ R (i=1,2,3) and x∈ R
Stement -2 If ∣∣ ∣∣a1b2c3a2b2c2a3b3c3∣∣ ∣∣ =0, then Δ =001:41View SolutionFor the system of equations :
a1x+b1y+c1z=0,a2x+b2y+c2z=0,a3x+b3y+c3z=0
if ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣=0, then this system has:06:07View SolutionIf ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣≠0∣∣ ∣∣a1+b1xa1x+b1c1a2+b2xa2x+b2c2a3+b3xa3x+b3c3∣∣ ∣∣=0 then x=
02:07View SolutionStatement 1: If the system of equations a1x+b1y+c1z=0,a2x+b2y+c2z=0 and a3x+b3y+c3z=0 have a solution (α,β,γ) such that αβγ≠0 then for any other non trivial solution (α1,β1,γ1),α1.β1.γ1≠0.
because
Statement 2 : If α,β,γ is any non trivial solution of the equations a1x+b1y+c1z=0,a2x+b2y+c2z=0 and a3x+b3y+c3z=0, then αβγ≠0.04:45View Solutionसमीकरणों a1x+b1y+c1=0 और azx+b2y+c2=0 में a1a2=b1b2≠c1c2, तो निम्नलिखित में से कौन - सा सत्य है ?
02:43View SolutionConsider the system of linear equations
a1x+b1y+c1z+d1=0,
a2x+b2y+c2z+d2=0,
a3x+b3y+c3z+d3=0, Let us denote by Δ (a,b,c) the determinant ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣ , if Δ(a,b,c)# 0, then the value of x in the unique solution of the above equations is05:24View SolutionConsider the system of linear equations
,a1x+b1y+c1z+d1=0,
a2x+b2y+c2z+d2=0
,a3x+b3y+c32+d3=0
Let us denote by Δ(a,b,c) the determinant ∣∣ ∣∣a1b1c1a2b2c2a3b2c3∣∣ ∣∣ if Δ(a,b,c)≠0, then the value of x in the unique solution of the above equations is06:05View Solutionif x,y and z are not all zero and connected by the equations a1x+b1y+c1z=0,azx+b2y+c2z=0 and (p1+λq1)x+(p2+λq2)y+(p3+λq3)z=0 show that
λ=−∣∣ ∣ ∣∣a1b1c1a2b2c2p1p2p3∣∣ ∣ ∣∣÷∣∣ ∣ ∣∣a1b1c1a2b2c2q1q2q3∣∣ ∣ ∣∣02:43View Solution