Mass of the earth has been determined through
use of Kepler's T2R3 constancy law
sampling the density of earth's crust and using earth's radius
Cavandish's determination of G and using earth's radius and g at its surface
use of periods of satellite at different heights above earth's surface.
The correct Answer is:C
To determine the mass of the Earth, we can use the following steps:
Step 1: Understanding Gravitational Force
The gravitational force between two masses is given by Newton's law of gravitation:
F=G⋅m1⋅m2r2
where:
- F is the gravitational force,
- G is the gravitational constant,
- m1 and m2 are the masses of the two objects,
- r is the distance between the centers of the two masses.
Step 2: Applying to Earth
In the case of the Earth, we can consider:
- m1 as the mass of the Earth (M),
- m2 as the mass of an object on the surface of the Earth (m),
- r as the radius of the Earth (R).
The gravitational force acting on the object can also be expressed as:
F=m⋅g
where g is the acceleration due to gravity at the Earth's surface.
Step 3: Setting the Forces Equal
Since both expressions represent the same gravitational force, we can set them equal to each other:
m⋅g=G⋅M⋅mR2
Step 4: Simplifying the Equation
We can cancel m from both sides (assuming m≠0):
g=G⋅MR2
Step 5: Solving for Mass of the Earth
Rearranging the equation to solve for M:
M=g⋅R2G
Step 6: Conclusion
Thus, the mass of the Earth can be determined using the value of g (acceleration due to gravity at the surface), the radius of the Earth R, and the gravitational constant G. This method is often referred to as the Cavendish determination of G and is a fundamental way to calculate the mass of the Earth.
Final Answer
The mass of the Earth has been determined through the Cavendish determination of G and using Earth's radius.
---
The gravitational force between two masses is given by Newton's law of gravitation:
F=G⋅m1⋅m2r2
where:
- F is the gravitational force,
- G is the gravitational constant,
- m1 and m2 are the masses of the two objects,
- r is the distance between the centers of the two masses.
In the case of the Earth, we can consider:
- m1 as the mass of the Earth (M),
- m2 as the mass of an object on the surface of the Earth (m),
- r as the radius of the Earth (R).
F=m⋅g
where g is the acceleration due to gravity at the Earth's surface.
Since both expressions represent the same gravitational force, we can set them equal to each other:
m⋅g=G⋅M⋅mR2
We can cancel m from both sides (assuming m≠0):
g=G⋅MR2
Rearranging the equation to solve for M:
M=g⋅R2G
Thus, the mass of the Earth can be determined using the value of g (acceleration due to gravity at the surface), the radius of the Earth R, and the gravitational constant G. This method is often referred to as the Cavendish determination of G and is a fundamental way to calculate the mass of the Earth.
The mass of the Earth has been determined through the Cavendish determination of G and using Earth's radius.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Acceleration due to gravity below and above the surface of earth8videos
GRAVITATION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Gravitational Potential energy7videos
GRAVITATION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Universal law of Gravitations14videos
GRAVITATION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason10videos
KINETIC THEORY
Similar Questions
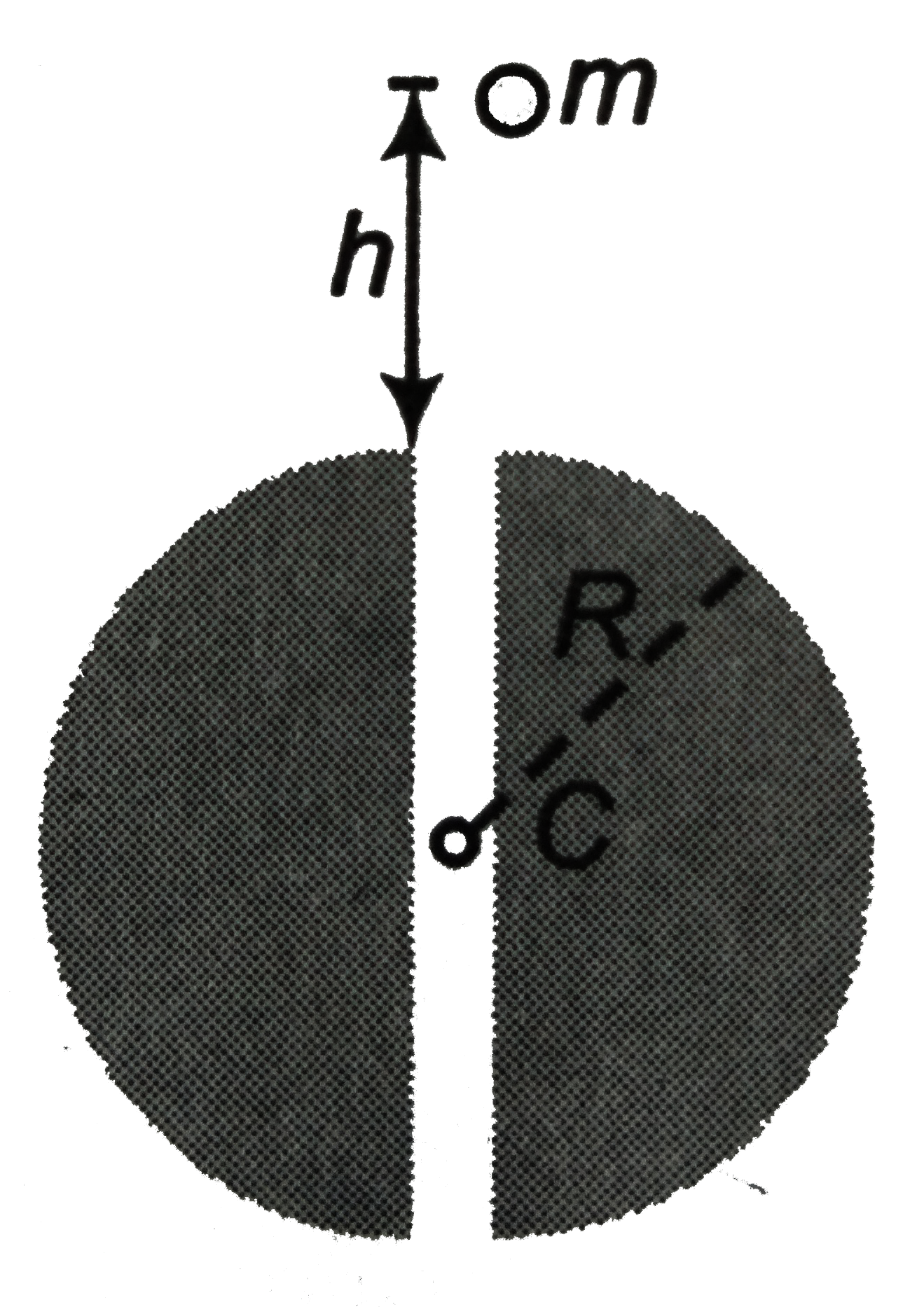
A ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
 View Solution
View SolutionLarge numbers of new varieties have been developed through mutation breeding and it has been used commonly in
View Solution
Knowledge Check
- Question 1 - Select One
Giant mouse has been produced through
ATissue cultureBGene differentiationCGene manipulationDAll the above
Zeatin has been obtained from
View SolutionThe age of the earth has been estimated by …………… dating technique.
View SolutionDistribution of clean and quality milk throughout the world has been made possible through work of
View SolutionSeedless watermelons have been obtained through
View SolutionConsider a planet in some solar system which has a mass double the mass of the earth and density equal to the average density of the earth. An object weighing W on the earth will weigh
View SolutionThe weight of an object on the surface of the Earth is 40 N. Its weight at a height equal to the radius of the Earth is
View SolutionThe distance between the centres of the Moon and the earth is D. The mass of the earth is 81 times the mass of the Moon. At what distance from the centre of the earth, the gravitational force will be zero?
View Solution

