The force on a particle of mass is N If it starts from rest what would be its position at time ?
The correct Answer is:A
To solve the problem step by step, we will follow these steps:
Step 1: Identify the given data
- Mass of the particle, m=10g=0.01kg
- Force acting on the particle, →F=10^i+5^jN
- Time, t=5s
Step 2: Calculate the acceleration
Using Newton's second law, we can find the acceleration →a using the formula:
→a=→Fm
Substituting the values:
→a=10^i+5^j0.01=1000^i+500^jm/s2
Step 3: Determine the initial velocity
Since the particle starts from rest, the initial velocity →u=0.
Step 4: Calculate the displacement
Using the equation of motion for displacement:
→s=→ut+12→at2
Substituting the known values:
→s=0+12(1000^i+500^j)(52)
Calculating 52=25:
→s=12(1000^i+500^j)×25
=12(25000^i+12500^j)
=12500^i+6250^jm
Step 5: Final result
Thus, the position of the particle at t=5s is:
→s=12500^i+6250^jm
- Mass of the particle, m=10g=0.01kg
- Force acting on the particle, →F=10^i+5^jN
- Time, t=5s
Using Newton's second law, we can find the acceleration →a using the formula:
→a=→Fm
Substituting the values:
→a=10^i+5^j0.01=1000^i+500^jm/s2
Since the particle starts from rest, the initial velocity →u=0.
Using the equation of motion for displacement:
→s=→ut+12→at2
Substituting the known values:
→s=0+12(1000^i+500^j)(52)
Calculating 52=25:
→s=12(1000^i+500^j)×25
=12(25000^i+12500^j)
=12500^i+6250^jm
Thus, the position of the particle at t=5s is:
→s=12500^i+6250^jm
Topper's Solved these Questions
Similar Questions
The force on a particle of mass 10 g is (→i10+→j5)N. If it starts from rest what would be its position at time t=5s?
View Solutionx−y is the vertical plane as shown in figure. A particle of mass 1kg is at (10m,20m) at time t=0. It is released from rest. Another particles of mass 2kg is at (20m,40m) at the same instant. It is projected with velocity (10ˆi+10ˆj)m/s. After 1s. Find (a) acceleration (b)velocity (c) position of the center of mass
View Solution
Knowledge Check
Find the torque of a force (7ˆi+3ˆj−5ˆk) about the origin. The force acts on a particle whose position vector is (ˆi−ˆj+ˆk).
A2ˆi+12ˆj+10ˆkB2ˆi+10ˆj+12ˆkC2ˆi+10ˆj+10ˆkD10ˆi+2ˆj+ˆk
A force of 10 N acts on a body of mass 0.5 kg for 0.25s starting from rest.What is its momentum now?
View SolutionA uniform force of (3ˆi+ˆj) N acts on a particle of mass 2kg. Hence, the particle is displaced from position (2ˆi+ˆk)m to position (4ˆi+3ˆj−ˆk)m. The work done by the force on the particle is
View SolutionA particle of mass 3 kg moves under a froce of [4ˆi+8ˆj+10ˆk] newton . Calcutale the acceleration (as vector) to which the particle is subjected. If the particle starts from rest and was at the origin initially. What are its new co - ordinates after 3 s?
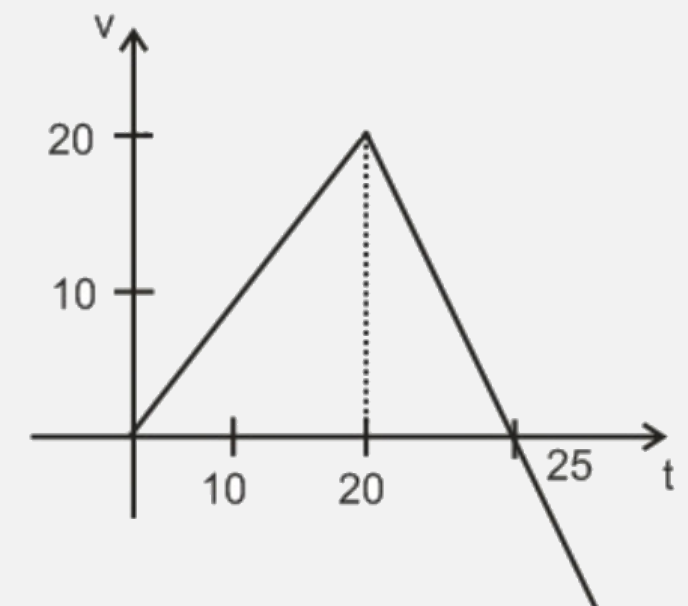
View SolutionThe figure shows the v−t graph of a particle moving in a straight line. Starting from rest, the time after which the particle returns to its starting position is
View SolutionA uniform force of (5ˆi+5ˆj)N acts on particle of mass 1 kg. The particle moves from r1=(3ˆi+4ˆj)m to r2=(5ˆi+8ˆj)m under this force. The work done by this force is
View SolutionA particle of mass 2kg is initially at rest. A force starts acting on it in one direction whose magnitude changes with time. The force time graph is shown in figure. Find the velocity of the particle at the end of 10s.

View SolutionA particle of mass m is driven by a machine that delivers a constant power k watts. If the particle starts from rest the force on the particle at time t is
View Solution
