Two absolute scales A and B have triple points of water defined to be 200A and 350B. What is the relation between TA and TB?
TA=4/7TB
TB=4/7TA
TA=2/7TB
TB=2/7TA
The correct Answer is:A
To find the relation between the temperatures TA and TB on two absolute scales A and B with their respective triple points of water defined as 200A and 350B, we can follow these steps:
1. Understand the Triple Point of Water:
The triple point of water is a specific temperature where water can exist in three phases: solid, liquid, and gas. This temperature is defined to be 273.16K.
2. Set Up the Equations:
Since the triple point of water is the same in both scales, we can equate the two definitions:
200A=273.16(1)
350B=273.16(2)
3. Find the Temperature Unit for Scale A:
From equation (1), we can express one unit of scale A in terms of Kelvin:
1A=273.16200K
4. Find the Temperature Unit for Scale B:
From equation (2), we can express one unit of scale B in terms of Kelvin:
1B=273.16350K
5. Relate the Two Scales:
To find the relationship between TA and TB, we can express TA in terms of TB:
TA=nA⋅1A=nA⋅273.16200
TB=nB⋅1B=nB⋅273.16350
6. Set the Equations Equal:
Since both temperatures correspond to the same physical condition (the triple point of water), we set the two equations equal:
273.16200⋅TA=273.16350⋅TB
7. Cancel 273.16:
We can cancel 273.16 from both sides:
TA200=TB350
8. Cross Multiply:
Cross multiplying gives:
350TA=200TB
9. Solve for TA:
Rearranging gives:
TA=200350TB=47TB
Final Relation:
Thus, the relation between TA and TB is:
TA=47TB
The triple point of water is a specific temperature where water can exist in three phases: solid, liquid, and gas. This temperature is defined to be 273.16K.
Since the triple point of water is the same in both scales, we can equate the two definitions:
200A=273.16(1)
350B=273.16(2)
From equation (1), we can express one unit of scale A in terms of Kelvin:
1A=273.16200K
From equation (2), we can express one unit of scale B in terms of Kelvin:
1B=273.16350K
To find the relationship between TA and TB, we can express TA in terms of TB:
TA=nA⋅1A=nA⋅273.16200
TB=nB⋅1B=nB⋅273.16350
Since both temperatures correspond to the same physical condition (the triple point of water), we set the two equations equal:
273.16200⋅TA=273.16350⋅TB
We can cancel 273.16 from both sides:
TA200=TB350
Cross multiplying gives:
350TA=200TB
Rearranging gives:
TA=200350TB=47TB
Thus, the relation between TA and TB is:
TA=47TB
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Heat Transper29videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Higher Order8videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Calorimetery4videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - NCERT Exemplar8videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTIONS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Assertion And Reason15videos
THERMODYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The graph between two temperature scales A and B is shown in Fig. Between upper fixed point and lower fixed point there are 150 equal divisions on scales A and 100 on scale B. The relation between the temperature in two scales is given by_
_S01_001_Q01.png) ATA−180100=TB150BTA−30150=TB100CTB−180150=TA100DTB−40100=TA180
ATA−180100=TB150BTA−30150=TB100CTB−180150=TA100DTB−40100=TA180
On the absolute scale triple point of water is one fixed temperature, what is the other?
View SolutionTwo bodies A and B having same angular momentum and IA>IB, then the relation between (K.E.)Aand(K.E.)B will be :
View SolutionThe basic difference between B and T-cells is
View SolutionIn the given figure what is the equivalent resistance between two points A and B?
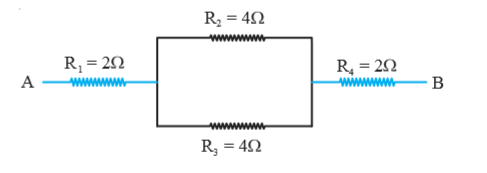 View Solution
View SolutionTwo samples of DNA, A and B have melting points 340K and 350 K respectively. This is because
View SolutionWhat is the functional difference between B cells and T cells.
View SolutionThe graph between two temperature scales A and B is shown in Fig. Between upper fixed point and lower fixed point there are 150 equal divisions on scales A and 100 on scale B. The relation between the temperature in two scales is given by_
_S01_001_Q01.png) View Solution
View Solution

