A fax message is to be sent from Delhi to washington via a geostationary satellite. Calculate the minimum time delay between
the dispatch and its getting received. Take
height of the geostationary satellite = 36000
km.

$72 \times 10^{3} k m$

$12 \times 10^{3} k m$

$27 \times 10^{3} k m$

$18 \times 10^{3} k m$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:A

A fax message is to be sent from Delhi to Washington via a geostationary satellite. The minimum distance between the dispatch and its getting received is (Take height of the geostationary satellite=36000km)
A $72 \times 10^{3} k m$
B $12 \times 10^{3} k m$
C $27 \times 10^{3} k m$
D $18 \times 10^{3} k m$
A satellite is to be placed in equatorial geostationary orbit around the earth for communication.
(a) Calculate height of such a satellite
(b) Find out the minimum number of satellites that are needed to cover entire earth, so that atleast one satellite is visible from any point on the equator.

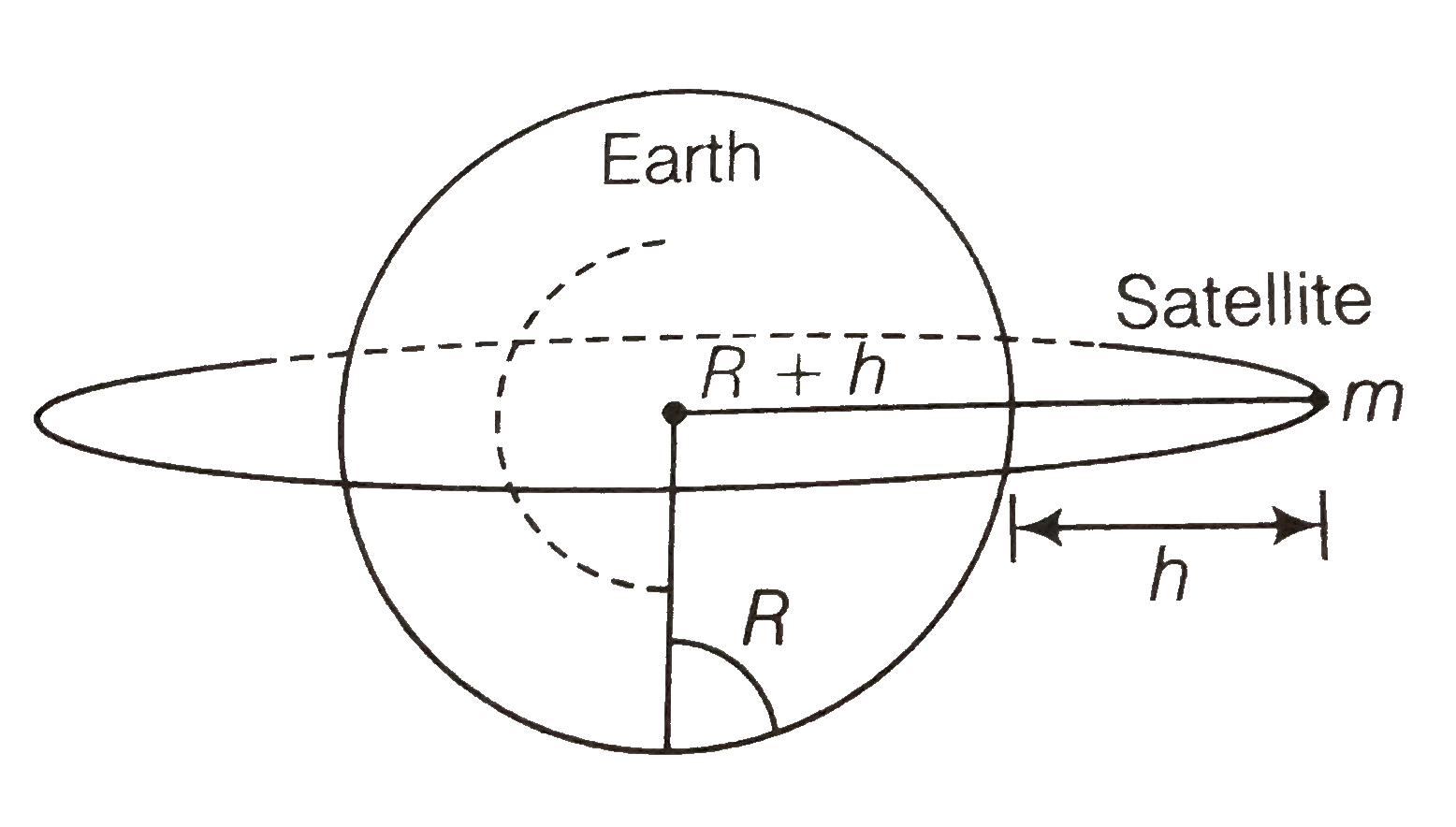
$[M = 6 \times 10^{24} k g, R = 6400 k m, T = 24 h, G = 6.67 \times 10^{- 11} SI unit]$
A $3.57 \times 10^{5} m$
B $3.57 \times 10^{6} m$
C $3.57 \times 10^{7} m$
D $3.57 \times 10^{8} m$
The orbit of geostationary satellite is circular, the time period of satellite depeds on (i) mass of the satellite, (ii) mass of earth, (iii) radius of the orbit and (iv) height of the satellite from the surface of the earth
A(i) only
B(i) and (ii)
C(i),(ii) and (iii)
D(ii),(iii) and (iv)