A plano convex lens has focal length f=20cm. If its plane surface is silvered, then new focal length will be
20cm
40 cm
30 cm
10 cm
The correct Answer is:D
To find the new focal length of a plano-convex lens when its plane surface is silvered, we can follow these steps:
1. Understand the Lens Configuration:
A plano-convex lens has one flat surface and one convex surface. The given focal length (f) of the lens is 20 cm.
2. Silvering the Plane Surface:
When the plane surface of the lens is silvered, it acts as a mirror. Therefore, we need to consider both the lens and the mirror effects together.
3. Use the Lens Maker's Formula:
The power (P) of the lens is given by:
P=1f
where f is the focal length of the lens. For our lens:
Plens=120 cm=0.05 cm−1
4. Power of the Silvered Surface:
The silvered plane surface acts like a mirror. The focal length of a plane mirror is considered to be infinite, hence its power is:
Pmirror=0 (since P=1f and f=∞)
5. Combine the Powers:
When the plane surface is silvered, the effective power of the system (lens + mirror) can be expressed as:
Ptotal=Plens+Pmirror
Since Pmirror=0:
Ptotal=Plens+0=Plens
6. Calculate the New Focal Length:
The new focal length f′ can be calculated from the total power:
Ptotal=1f′
Therefore:
1f′=2⋅Plens
Since the effective power of the lens is doubled when the plane surface is silvered:
Ptotal=2⋅0.05=0.1 cm−1
Thus:
f′=1Ptotal=10.1=10 cm
Conclusion:
The new focal length of the plano-convex lens when its plane surface is silvered is 10 cm.
A plano-convex lens has one flat surface and one convex surface. The given focal length (f) of the lens is 20 cm.
When the plane surface of the lens is silvered, it acts as a mirror. Therefore, we need to consider both the lens and the mirror effects together.
The power (P) of the lens is given by:
P=1f
where f is the focal length of the lens. For our lens:
Plens=120 cm=0.05 cm−1
The silvered plane surface acts like a mirror. The focal length of a plane mirror is considered to be infinite, hence its power is:
Pmirror=0 (since P=1f and f=∞)
When the plane surface is silvered, the effective power of the system (lens + mirror) can be expressed as:
Ptotal=Plens+Pmirror
Since Pmirror=0:
Ptotal=Plens+0=Plens
The new focal length f′ can be calculated from the total power:
Ptotal=1f′
Therefore:
1f′=2⋅Plens
Since the effective power of the lens is doubled when the plane surface is silvered:
Ptotal=2⋅0.05=0.1 cm−1
Thus:
f′=1Ptotal=10.1=10 cm
The new focal length of the plano-convex lens when its plane surface is silvered is 10 cm.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Reflection Of Light By Spherical Mirrors5videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Refraction10videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSERTION AND REASON15videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 350videos
PRACTICE PAPPER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS EXERCISE - Assertion And Reason15videos
SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS
Similar Questions
The radius of curvature of curved surface of a thin plano-convex lens is 10cm and the refractive index is 1.5. If the plano surface is silvered, then the focal length will be.
View SolutionA planocovex lens is made of a material of refractive in μ=1.5 The radius of curvature of curved surface of the lens is 20.cm. If its plane surface3 is silvered, the focal length of the silvered lens will be
View SolutionIf the plane surface of a plano-convex lens of radius of curvature R and refractive index μ is silvered, then its focal length would be
View SolutionA point object is placed at a distance of 20cm from a thin plano-concex lens of focal length 15cm. The plane surface of the lens is now silvered. The image created by the is at :
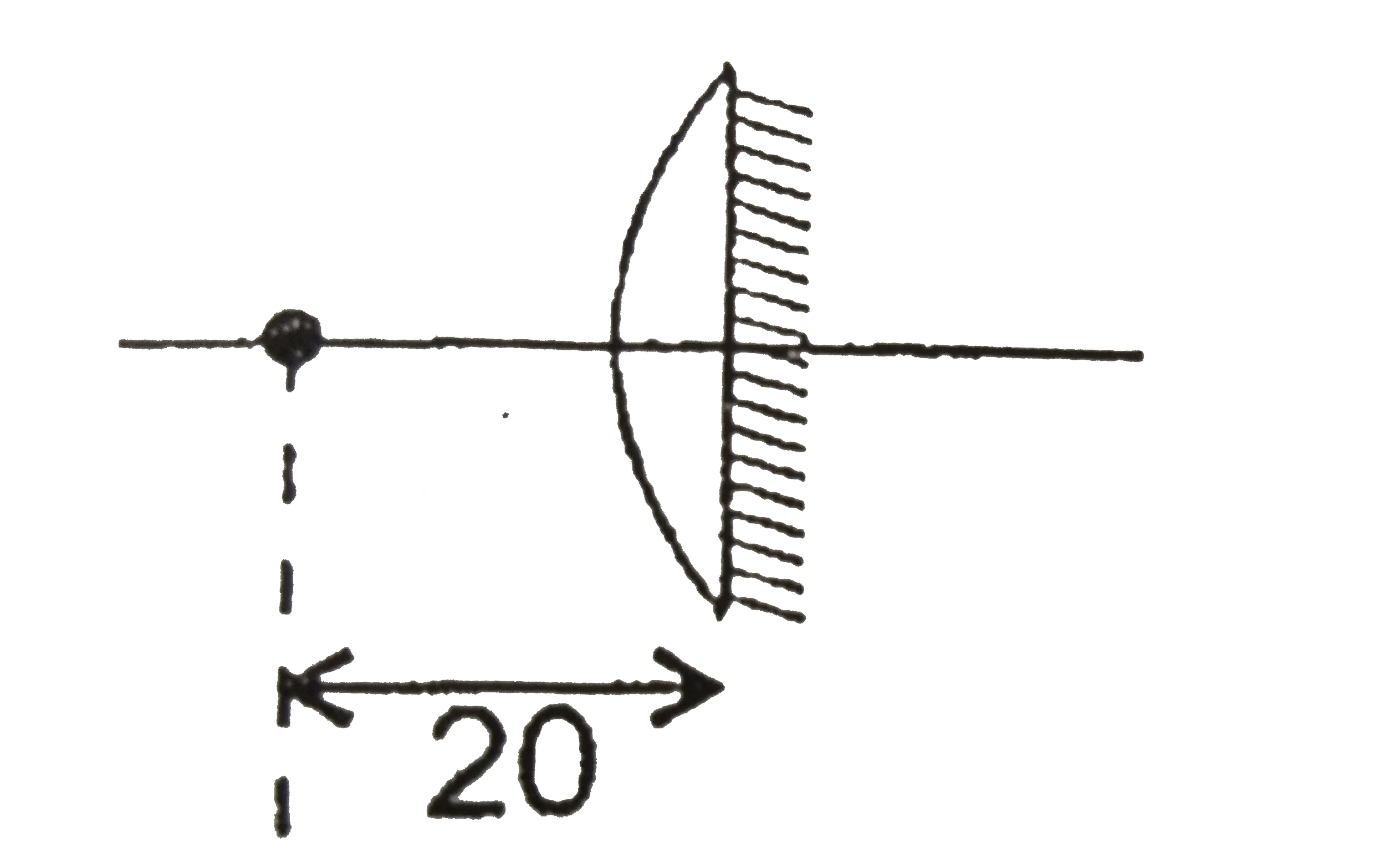 View Solution
View SolutionA point object is placed at a distance of 20cm from a thin plano-convex lens of focal length 15cm(μ=1.5). The curved surface is silvered. The image will form at
 View Solution
View SolutionA point object is placed at a distance of 20cm from a thin plano-convex lens of focal length 15cm(μ=1.5). The curved surface is silvered. The image will form at
 View Solution
View SolutionA plano-convex thin lens of focal length 10cm is silvered at its plane surface. The distance d at which an object must be kept in order to get its image on itself is
 View Solution
View SolutionA convex lens of focal length 20cm is placed 10cm away from a second convex lens of focal length 25 cm. What wil be the location of the image of an object at 30 cm in front of the first lens?
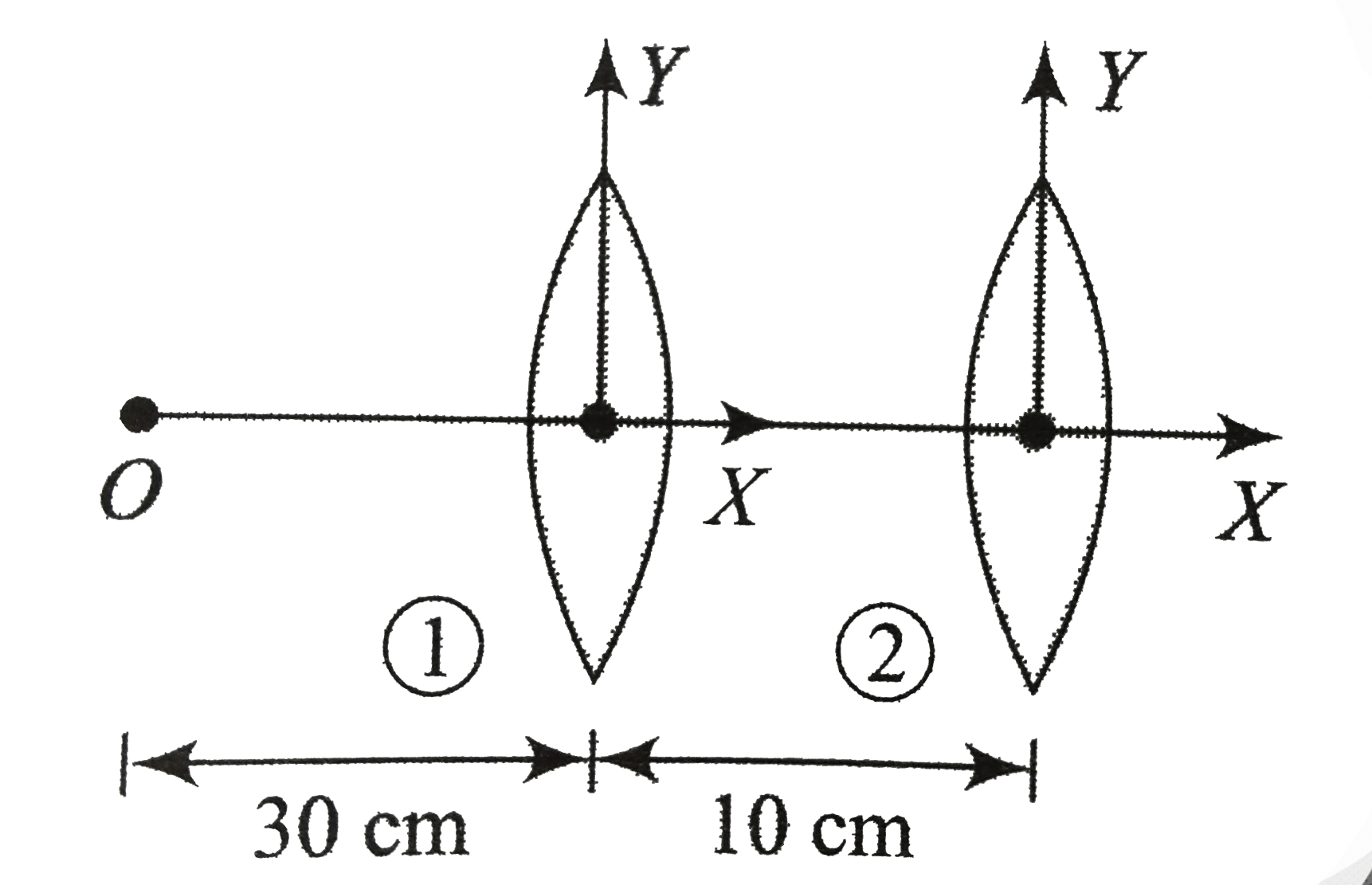 View Solution
View SolutionA thin convex lens of focal length 20 cm is kept in contact with a thin concave lens of focal length 15 cm. Find the focal length and the nature of the combination.
View SolutionA thin lens (μ=1.5) of focal length + 12 cm is immersed in water (μ=1.33). What is its new focal length ?
View Solution

