The size of the image of an object, which is at infinity, as formed by a convex lens of focal length 30 cm is 2 cm. If a concave lens of focal length 20 cm is placed between the convax lens and the image at a distance of 26 cm from the convex lens, calculate the new size of the image.
1.25cm
2.5cm
1.05cm
2cm
The correct Answer is:B
To solve the problem step by step, we will analyze the situation involving a convex lens and a concave lens.
Step 1: Understand the initial conditions
- We have a convex lens with a focal length f1=30cm.
- An object is at infinity, which means the rays coming from the object are parallel.
- The image formed by the convex lens is given as hi=2cm.
Step 2: Determine the position of the image formed by the convex lens
For an object at infinity, the image formed by a convex lens is at its focus. Therefore, the image distance v1 from the convex lens is:
v1=f1=30cm
Step 3: Identify the position of the concave lens
The concave lens has a focal length f2=−20cm (negative because it is a concave lens). The distance between the convex lens and the concave lens is 26cm. Thus, the distance from the convex lens to the image formed by it is:
d=v1−26cm=30cm−26cm=4cm
This means that the image formed by the convex lens acts as a virtual object for the concave lens, located 4cm in front of it.
Step 4: Calculate the image distance for the concave lens
Using the lens formula for the concave lens:
1f=1v−1u
Where:
- f=−20cm (focal length of the concave lens)
- u=−4cm (object distance for the concave lens, negative because the object is virtual and on the same side as the incoming light)
Substituting the values:
1−20=1v−1−4
This simplifies to:
1v=1−20+14
Finding a common denominator (20):
1v=−1+520=420=15
Thus, the image distance v is:
v=5cm
Step 5: Calculate the magnification produced by the concave lens
The magnification m produced by the concave lens is given by:
m=hiho=vu
Where ho=2cm (height of the original image) and u=−4cm:
m=5−4=−1.25
Step 6: Calculate the new size of the image
The new height of the image h′i can be calculated as:
h′i=m⋅ho=−1.25⋅2cm=−2.5cm
The negative sign indicates that the image is inverted.
Final Answer
The new size of the image is 2.5cm (inverted).
---
- We have a convex lens with a focal length f1=30cm.
- An object is at infinity, which means the rays coming from the object are parallel.
- The image formed by the convex lens is given as hi=2cm.
For an object at infinity, the image formed by a convex lens is at its focus. Therefore, the image distance v1 from the convex lens is:
v1=f1=30cm
The concave lens has a focal length f2=−20cm (negative because it is a concave lens). The distance between the convex lens and the concave lens is 26cm. Thus, the distance from the convex lens to the image formed by it is:
d=v1−26cm=30cm−26cm=4cm
This means that the image formed by the convex lens acts as a virtual object for the concave lens, located 4cm in front of it.
Using the lens formula for the concave lens:
1f=1v−1u
Where:
- f=−20cm (focal length of the concave lens)
- u=−4cm (object distance for the concave lens, negative because the object is virtual and on the same side as the incoming light)
1−20=1v−1−4
This simplifies to:
1v=1−20+14
Finding a common denominator (20):
1v=−1+520=420=15
Thus, the image distance v is:
v=5cm
The magnification m produced by the concave lens is given by:
m=hiho=vu
Where ho=2cm (height of the original image) and u=−4cm:
m=5−4=−1.25
The new height of the image h′i can be calculated as:
h′i=m⋅ho=−1.25⋅2cm=−2.5cm
The negative sign indicates that the image is inverted.
The new size of the image is 2.5cm (inverted).
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT EXAMPLAR PROBLEMS
11 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSERTION AND REASON
15 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 3
50 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
A convex lens of focal length 40 cm is in contact with a concave lens of focal length 25 cm. The power of the combination is
View SolutionA convex lens of focal length 30 cm forms an image of height 2 cm for an object situated at infinity. If a concave lens of focal length 20 cm is placed coaxially at a distance of 26 cm from convex lens then size of image would be
View Solution
Knowledge Check
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Areal, at 30 cm in front of the mirrorBreal, at 30 cm behind the mirrorCreal, at 10 cm in front of the mirrorDvirtual, at 10 cm behind the mirror
An object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the image
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
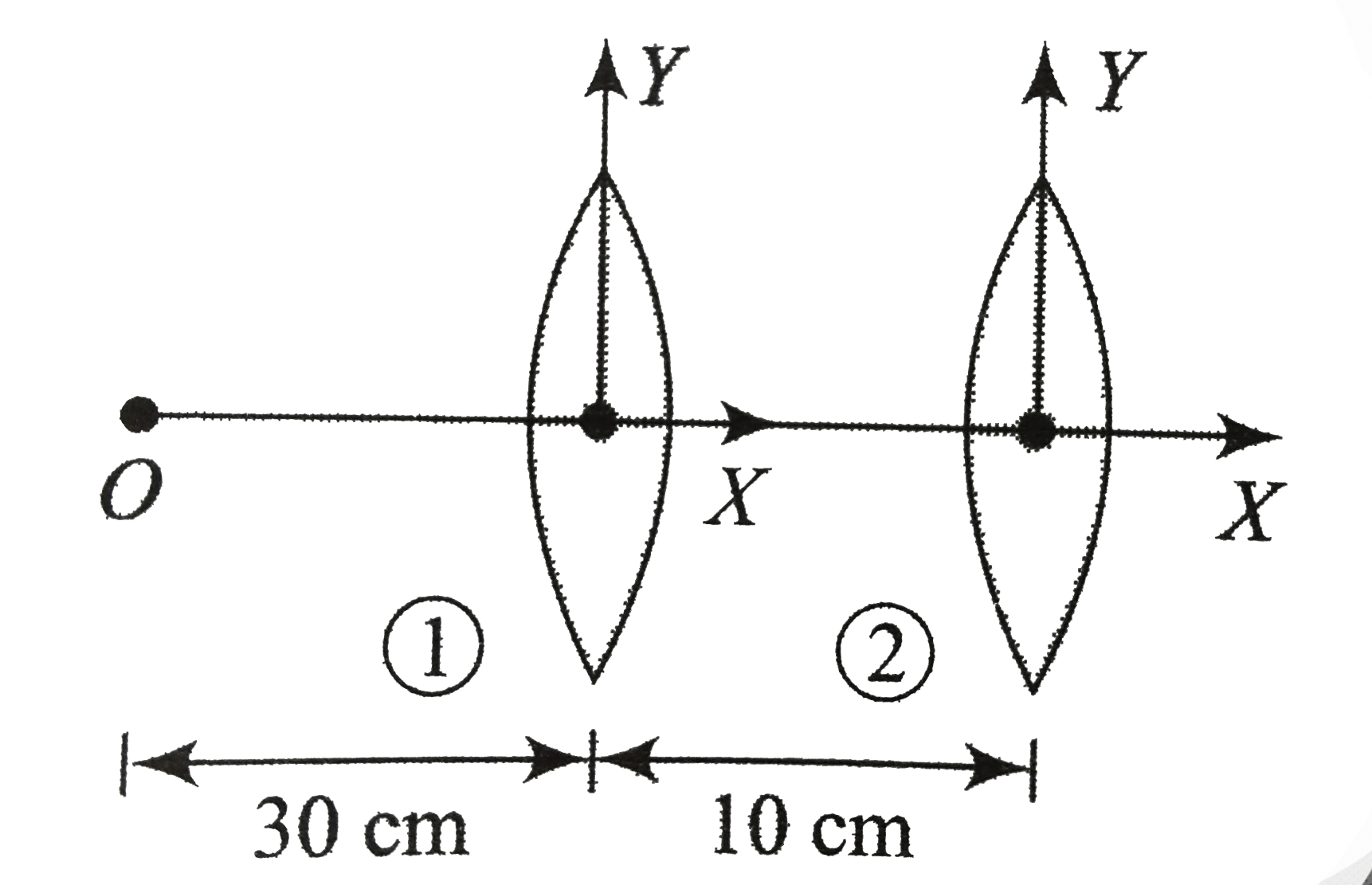
View SolutionA convex lens of focal length 20cm is placed 10cm away from a second convex lens of focal length 25 cm. What wil be the location of the image of an object at 30 cm in front of the first lens?
View SolutionAn object 25 cm high is placed in front of a convex lens of focal length 30 cm . If the height of image formed is 50 cm , find the distance between the object and the image (real and virtual)?
View SolutionIf the distances of an object and its virtual image from the focus of a convex lens of focal length f are 1 cm each, then f is
View SolutionIf the distances of an object and its virtual image from the focus of a convex lens of focal length f are 1 cm each, then f is
View SolutionWhat is the focal length of a convex lens of focal length 30cm in contact with a concave lens of focal length 20cm. Is the system a converging or a diverging lens ? Ignore thickness of the lenses.
View Solution
