if three are N atoms in a source of laser light and each atom is emitting light with intensity l, then the total intensity produced by it is
NI
N2I
N3I
N4I
The correct Answer is:B
To solve the problem of finding the total intensity produced by N atoms in a source of laser light, where each atom emits light with intensity I, we can follow these steps:
Step 1: Understand the Concept of Intensity
Intensity (I) is defined as the power per unit area carried by a wave. In the context of laser light, each atom emits light with a certain intensity.
Hint: Recall that intensity is related to the energy emitted by the source and the area over which it is distributed.
Step 2: Identify the Number of Atoms
We are given that there are N atoms in the source of laser light. Each of these atoms contributes to the total intensity.
Hint: Think about how the contributions from multiple sources combine.
Step 3: Calculate the Total Intensity
Since each atom emits light with intensity I, the total intensity produced by N atoms can be calculated by multiplying the intensity of one atom by the number of atoms.
Total Intensity=N×I
Hint: Consider how intensities from multiple sources add up in a linear manner.
Step 4: Consider the Nature of Laser Light
In laser light, the emitted light from each atom is coherent and in phase, which can lead to constructive interference. However, for the purpose of this problem, we are simply summing the contributions from each atom.
Hint: Remember that in coherent sources, the intensity can be affected by interference, but here we are focusing on the basic addition of intensities.
Step 5: Final Expression
Thus, the total intensity produced by N atoms, each emitting light with intensity I, is given by:
Total Intensity=N⋅I
Conclusion
The total intensity produced by N atoms in a source of laser light, where each atom emits light with intensity I, is N⋅I.
---
Intensity (I) is defined as the power per unit area carried by a wave. In the context of laser light, each atom emits light with a certain intensity.
We are given that there are N atoms in the source of laser light. Each of these atoms contributes to the total intensity.
Since each atom emits light with intensity I, the total intensity produced by N atoms can be calculated by multiplying the intensity of one atom by the number of atoms.
In laser light, the emitted light from each atom is coherent and in phase, which can lead to constructive interference. However, for the purpose of this problem, we are simply summing the contributions from each atom.
Thus, the total intensity produced by N atoms, each emitting light with intensity I, is given by:
The total intensity produced by N atoms in a source of laser light, where each atom emits light with intensity I, is N⋅I.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ATOMS EXERCISE - Higher order thinking skills8videos
ATOMS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ATOMS EXERCISE - NCERT Examplar problems7videos
ATOMS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assertion And Reason15videos
ALTERNATING CURRENT
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - COMMUNITCATION SYSTEMSEXERCISE - Assertion And Reason30videos
COMMUNITCATION SYSTEMS
Similar Questions
Knowledge Check
- Question 1 - Select One
During high light intensity, the chloroplasts align themselves
Ain vertical position along lateral wallsBalong tangential wallsCin centre and get scatteredDperpendicular to light. - Question 2 - Select One
Plants adapted to low light intensity have
Ahigher rate of CO2 fixation than the sun plantsBmore extended root systemCleaves modified to spinesDlarger photosynthetic unit size than the sun plants
How the light intensity affects the rate of photosynthesis ?
View SolutionPlants adapted to low light intensity have
View SolutionTwo incoherent sources of light emitting light of intensity I0 and 3I0 interfere in a medium. Calculate, the resultant intensity at any point.
View SolutionA ray of light is incident on the left vertical face of the glass slab. If the incident light has an intensity I and on each reflection the intensity decreases by 90% and on each refraction the intensity decreases by 10%, find the ratio of the intensities of maximum to minimum in reflected pattern.
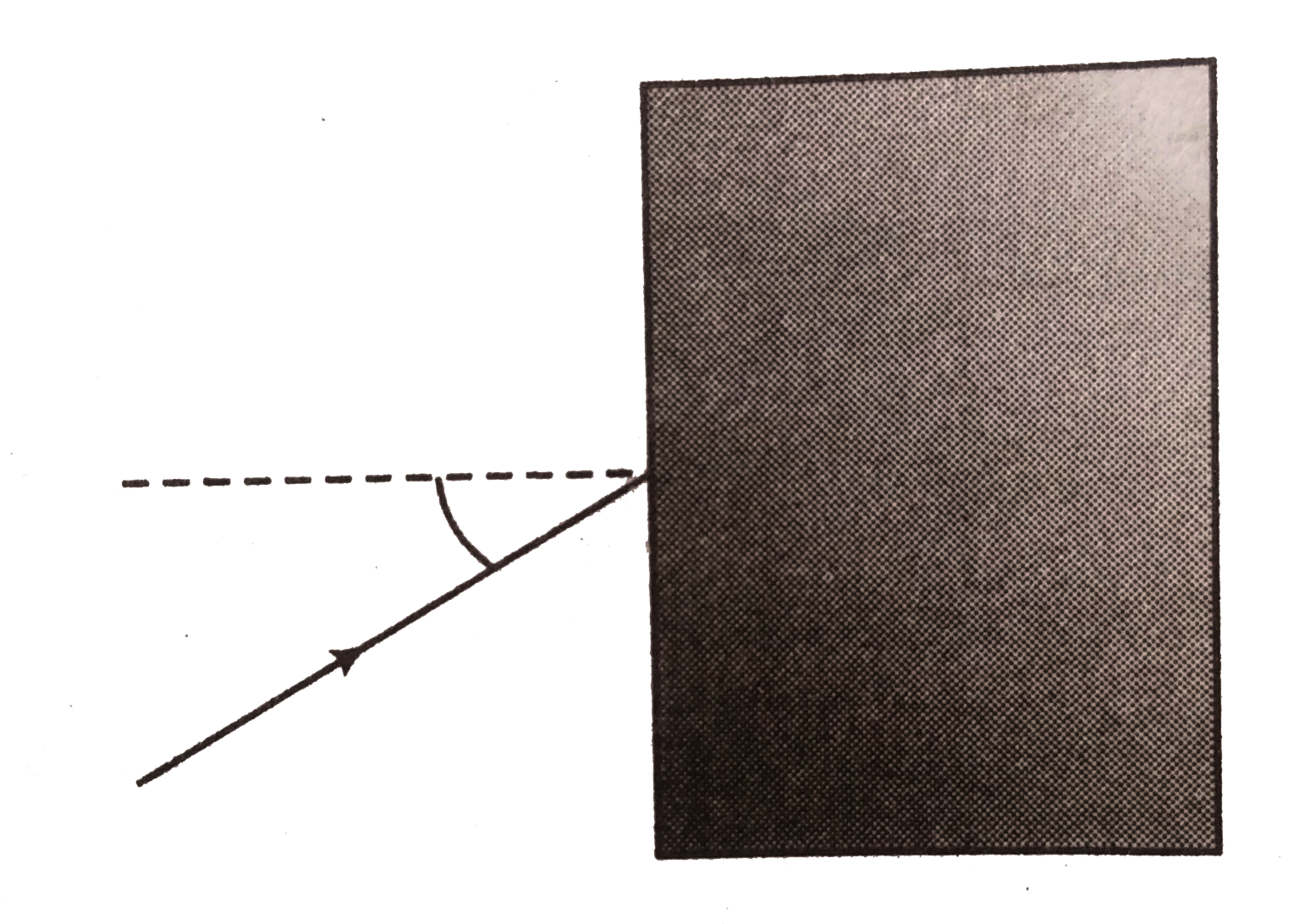 View Solution
View Solutionn identical coherent waves each with the same initial phase arrive at a point with identical path length. The intensity produced at this point is l1. If the waves are all incoherent, the intensity produced is l2. The ratio l1l2 is
View SolutionState one factor which determines the intensity of light in the photon picture of light.
View Solution

