A non conducting sphere of radius a has a net charge +q uniformly distributed throughout its volume. A spherical conducting shell having inner and outer radii b and c and net charge -q is concentric with the sphere (see the figure).
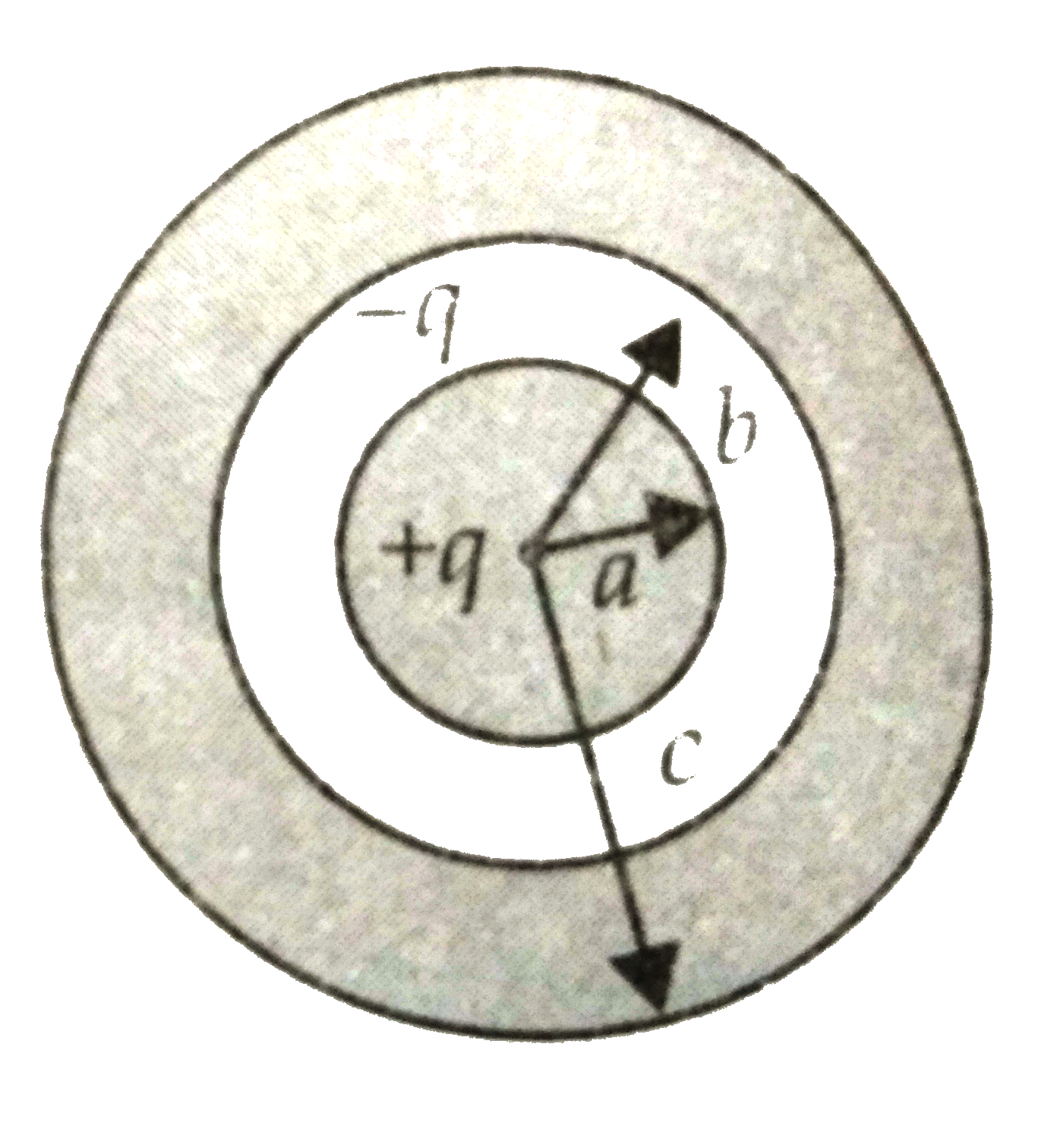
Read the following statements
(i) The electric field at a distance r from the centre of the sphere for r lt a is 14πε0qra3
(ii) The electric field at distance r for a lt r lt b is 0
(iii) The electric field at distance r for b lt r lt c is 0
(iv) The charge on the inner surface of the spherical shell is - q
(v) The charge on the outer surface of the spherical shell is + q
Which of the above statements are true?
(i), (ii) and (v)
(i), (iii) and (iv)
(ii), (iii) and (iv)
(ii), (iii) and (v)
The correct Answer is:B
Step by step video & image solution for A non conducting sphere of radius a has a net charge +q uniformly distributed throughout its volume. A spherical conducting shell having inner and outer radii b and c and net charge -q is concentric with the sphere (see the figure). Read the following statements (i) The electric field at a distance r from the centre of the sphere for r lt a is 1/(4piepsilon_(0))(qr)/a^(3) (ii) The electric field at distance r for a lt r lt b is 0 (iii) The electric field at distance r for b lt r lt c is 0 (iv) The charge on the inner surface of the spherical shell is - q (v) The charge on the outer surface of the spherical shell is + q Which of the above statements are true? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - ASSERTION & REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Electric Charges
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT Exemplar
6 Videos
Similar Questions
The electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View SolutionThe electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View SolutionA non-conducting sphere of radius R is given a charge Q. Charge is uniformly distributed in its volume. The electric potential at centre and at the surface of sphere respectively are
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View SolutionFor a uniformly charged non conducting sphere of radius R which of following shows a correct graph between the electric field intensity and the distance from the centre of sphere –
View SolutionA hollow non conducting sphere of radius R has a positive charge q uniformly distributed on its surface. The sphere starts rotating with a constant angular velocity 'ω' about an axis passing through center of sphere, as shown in the figure. Then the net magnetic field at center of the sphere is

View SolutionA hollow conducting sphere of inner radius r and outer radius 2R is given charge Q as shown in figure, then the

View SolutionThe electric field at 2R from the centre of a uniformly charged non - conducting sphere of rarius R is E. The electric field at a distance R2 from the centre will be
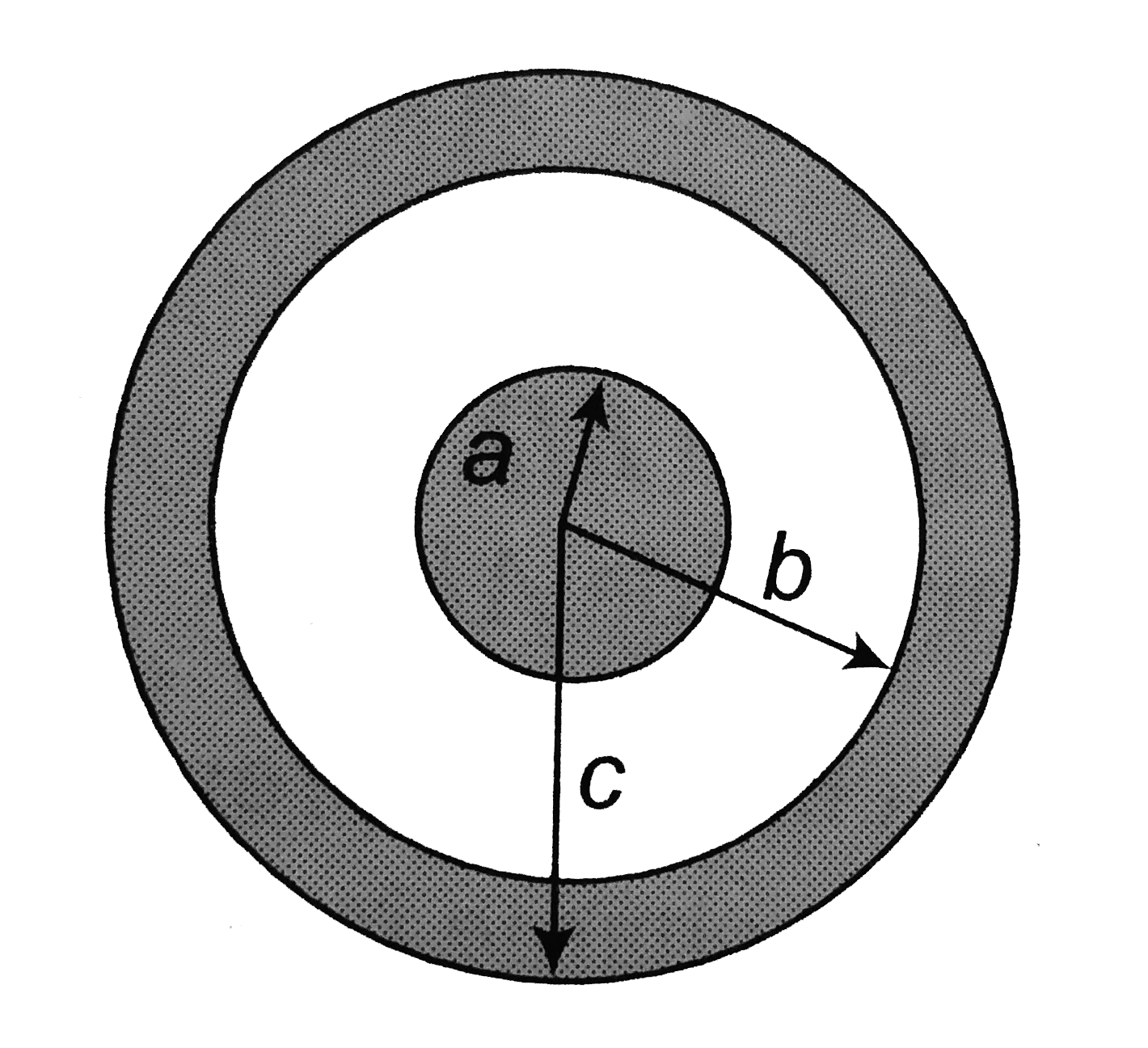
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
View Solution
