A shell is fired from a long range gun with an initial velocity u=1000ms−1 at an angle 30∘ to the horizon. How long will the shell be in the air? At what distance from the gun will it fall to the ground ? The gun and the pont where the shell lands are on the same horizontal line.
The correct Answer is:102s, 88400 m
Similar Questions
A shell is fired from a long - range gun with an intial velocity u=1000ms−1 at an angle 30∘ to the horizontal. How long with the shell be in the air ? At what distance from the gun will it fall to ground ? The gun and the point where the shell lands are on the same horizontal line .
View SolutionA shell is fired from a long range gun with an initial velocity u=1000ms−1 at an angle 30∘ to the horizon.How long will the shell be in the air? (g=10.0ms−2)
View Solution
Knowledge Check
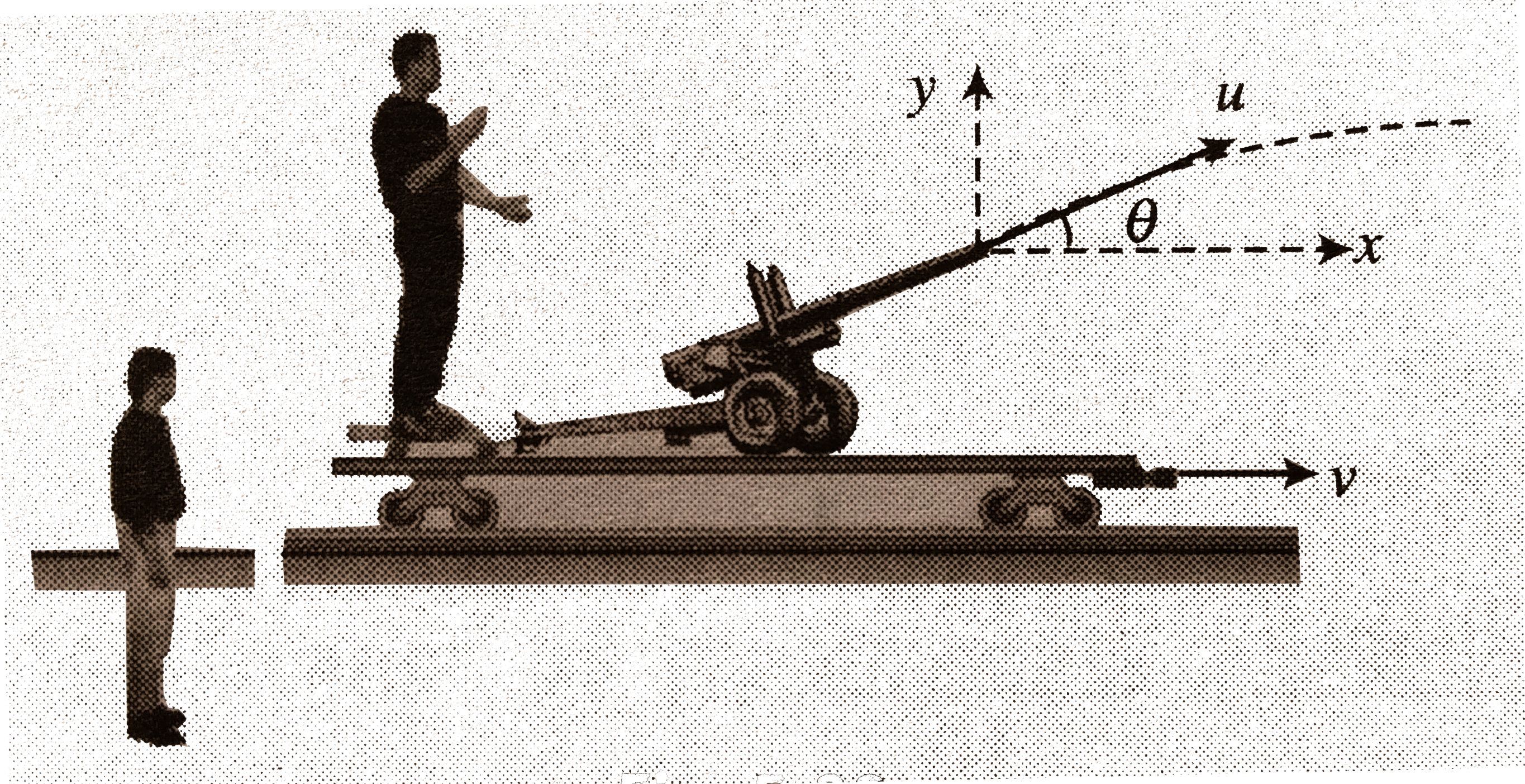
A shell is projected from a gun with a muzzle velocity v.The gun is fitted with a trolley car at an angle θ as shown in the fig. If the trolley car is made to move with constant velocity v towards right, find the horizontal range of the shell relative to ground.
 AR=2usinθ(ucosθ+v)gBR=2usinθ(ucosθ−v)gCR=usinθ(ucosθ+v)gDR=usinθ(ucosθ−v)g
AR=2usinθ(ucosθ+v)gBR=2usinθ(ucosθ−v)gCR=usinθ(ucosθ+v)gDR=usinθ(ucosθ−v)gA gun is mounted on a plateau 960 m away from its edge as shown. Height of plateau is 960 m The gun can fire shells with a velocity of 100 m/s at any angle. Of the following choices, what is the minimum distance (OP)x from the edge of plateau where the shell of gun can reach?
 A480mB720mC360mDnone
A480mB720mC360mDnoneTwo enemy guns are placed at A and B at 10√3km apart horizontally . A shell is fired from A horizontally with velocity 10m/s. At the same time a shell of double the mass of shell at A is fired from B at an angle 60∘ with horizontal towards A with the same magnitude of initial velocity as that of A. Moving in the same vertical plane, two shells collide in air while sticking to each other and falling at the depest point of the valley C (neglect air resistance).

Find the time of collision of the two shells (in sec).A200√3B20√3C2×103√3D2×104√3
A shell of mass m is fired from a gun of mass km which can reecoil freely on a horizontal plane, the elevation of the gun is 45∘. Find the ratio of te energy of the shell to that of the gun.
View SolutionA shell is fired from a gun from the bottom of a hill along its slope. The slope of the hill is α=30∘, and the angle of the barrel to the horizontal β=60∘. The initial velocity u of the shell is 21ms−1. Find the distance from the gun to the point at which the shell falls.
View SolutionA shell is projected from a gun with a muzzle velocity, u. The gun is fitted with a trolly car at an angle θ as shown in (Fig. 5.33). If the trolley car is made to move with constant velocity v towards right, find the
(a) horizontal range of the shell relative to ground.
(b) horizontal range of the shell relative to a person travelling with trolley.
 .
.
View SolutionA shell is projected from a gun with a muzzle velocity, u. The gun is fitted with a trolly car at an angle θ as shown in (Fig. 5.33). If the trolley car is made to move with constant velocity v towards right, find the
(a) horizontal range of the shell relative to ground.
(b) horizontal range of the shell relative to a person travelling with trolley.
 .
.
View SolutionTwo enemy guns are placed at A and B at 10√3km apart horizontally . A shell is fired from A horizontally with velocity 10m/s. At the same time a shell of double the mass of shell at A is fired from B at an angle 60∘ with horizontal towards A with the same magnitude of initial velocity as that of A. Moving in the same vertical plane, two shells collide in air while sticking to each other and falling at the depest point of the valley C (neglect air resistance).

Find the magnitude of horizontal component of the displacement vector (→BC) (inkm).
View Solution
