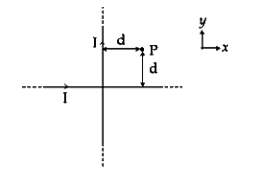
Infinite number of straight wires each carrying current I areequally placed as shown in the figure. Adjacent wires have current in opposite direction. Find net magnetic field at point P?
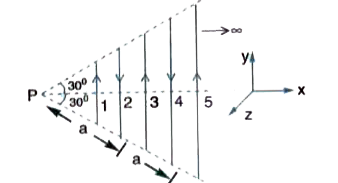
Bnet=μ0I4π(sin30∘+sin30∘)ˆk[1d−12d+13d−14d.......+∞]
(where d = a cos 30 =√3a2)
∴Bnet=μ0I2√3πaˆk[1−12+13−14+.....∞]
=μ0I√3πaln2ˆk=μ0I4π=ln4√3aˆk
Topper's Solved these Questions
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )
18 Videos
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - PROBLEMS (LEVEL -I)
30 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
20 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
Similar Questions
Infinite number of straight wires each carrying current I are equally placed as shown in the figure Adjacent wires have current in opposite direction Net magnetic field at point P is
 .
.
View SolutionFour long wires each carrying current I as shown in the figure are placed at the points A, B, C and D. Find the magnitude and direction of
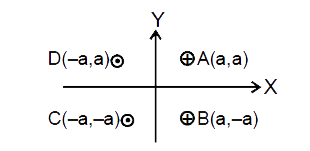
magnetic field at the centre of the square.
View Solution
Knowledge Check
Infinite number of straight wires each carrying current I are equally placed as shown in the figure Adjacent wires have current in opposite direction Net magnetic field at point P is
 .Aμ0I4πIn2√3aˆkBμ0I4πIn4√3aˆkCμ0I4πIn4√3a(−ˆk)Dzero
.Aμ0I4πIn2√3aˆkBμ0I4πIn4√3aˆkCμ0I4πIn4√3a(−ˆk)DzeroTwo perpendicular infinite wires are carrying current i as shown in the figure. The magnetic field at point P is :
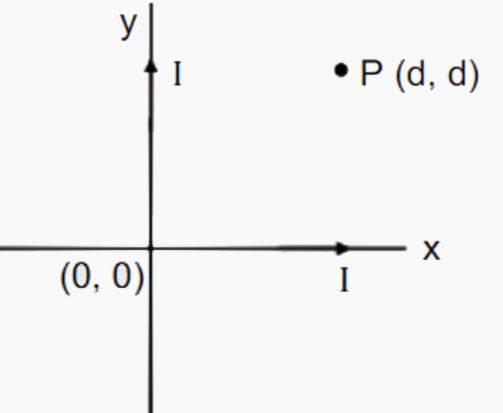 Aμ01πdBμ012πdCμ012πdDZero
Aμ01πdBμ012πdCμ012πdDZeroA non - planar loop of conducting wire carrying a current I is placed as shown in the figure . Each of the straighrt sections of the loop is of the length 2a. The magnetic field due to this loop at the point P(a,0,a) points in the direction
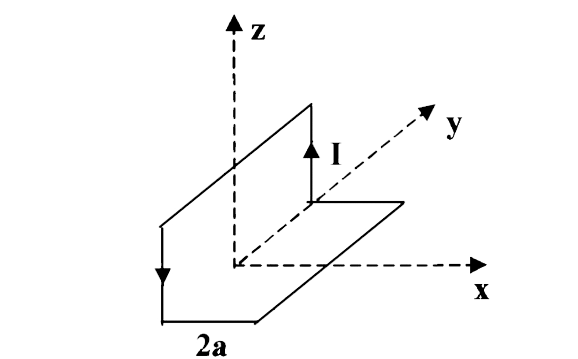 A1√2(−ˆj+ˆk)B1√3(−ˆj+ˆk+ˆi)C1√3(ˆi+ˆj+ˆk)D1√2(ˆi+ˆk)
A1√2(−ˆj+ˆk)B1√3(−ˆj+ˆk+ˆi)C1√3(ˆi+ˆj+ˆk)D1√2(ˆi+ˆk)
Two perpendicular infinite wires are carrying current i as shown in the figure. The magnetic field at point P is :
_2019_CBT2_E01_008_Q01.png)
View SolutionTwo very long straight , and insulated wires are Kept at 90∘ angle from each other in xy-plane as shown in the figure.
 These wires carry current of equal magnitude I, whose direction are shown in the figure The net magnetic field at point P will be:
These wires carry current of equal magnitude I, whose direction are shown in the figure The net magnetic field at point P will be:
View SolutionA non - popular loop of conducting wire carrying a current I is placed as shown in the figure . Each of the straighrt sections of the loop is of the length 2a. The magnetic field due to this loop at the point P(a,0,a) points in the direction

View Solution(a) Find the magnetic field at the point O if the wire carrying a current i has the shape shown. The radius of the current part of the wire is R, the linear parts of the wire are very long.
(i)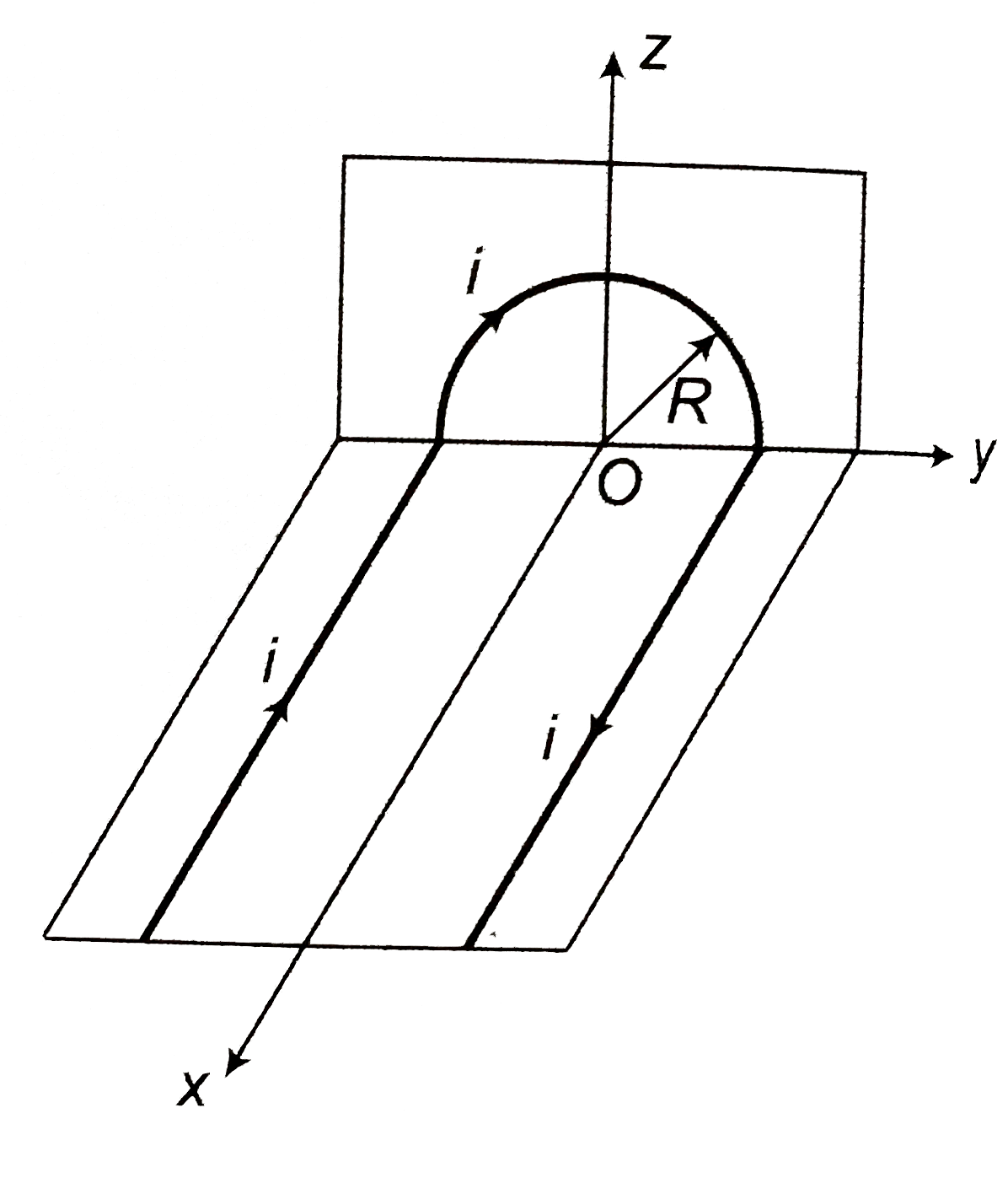
(ii)
(iii)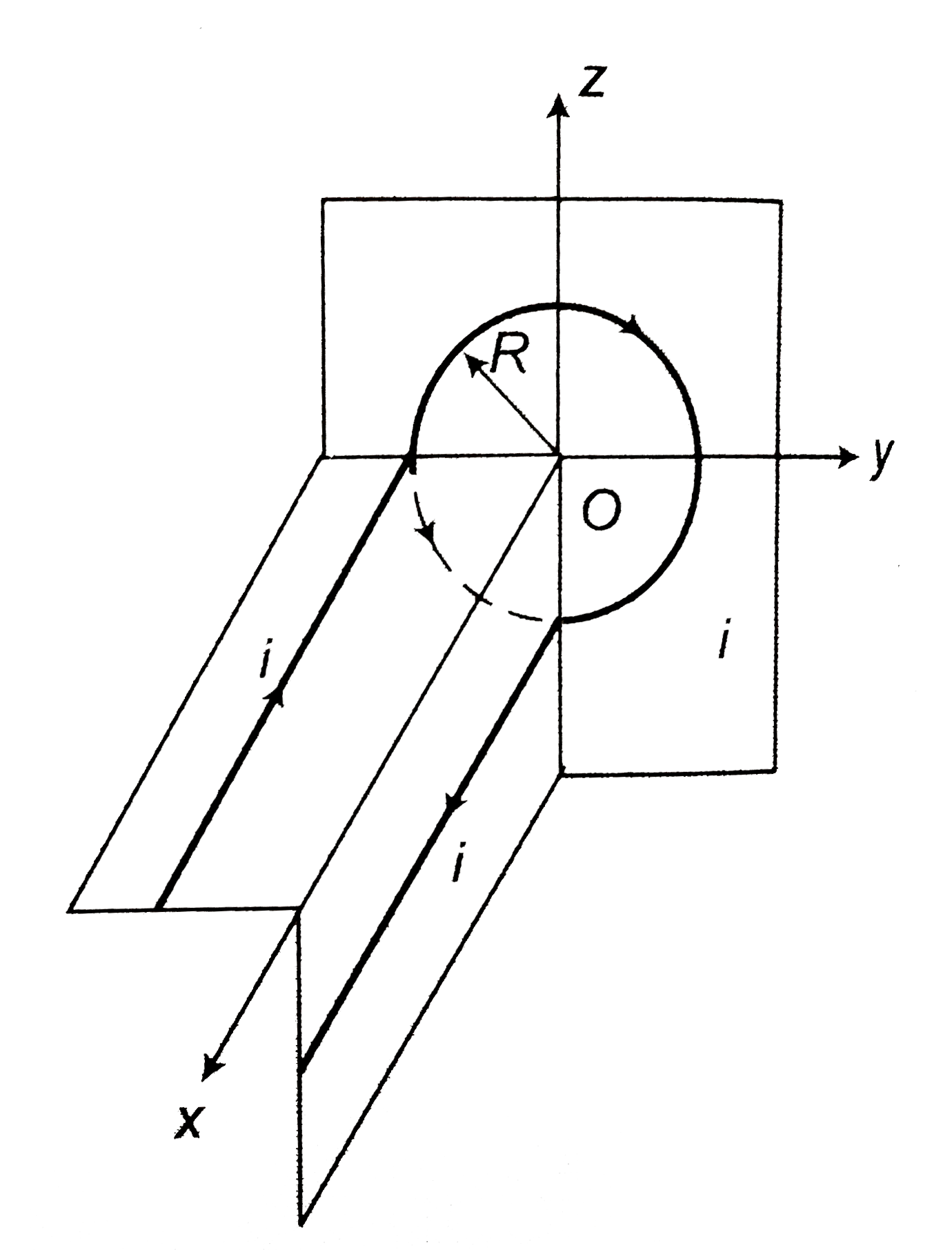
(b) A long straight wire along the z-axis carriers a current i in negative z-direction. Find magnetic field in vector from at a point having co-ordinates (x,y) on z=0 plane.
(c) A non-coplaner loop of conducting wire carrying a current I is placed as shown in the figure. Each of the straight sections of the loop of length 2a. find unit vector along magnetic field magnetic filed at the point P(a,0,a).
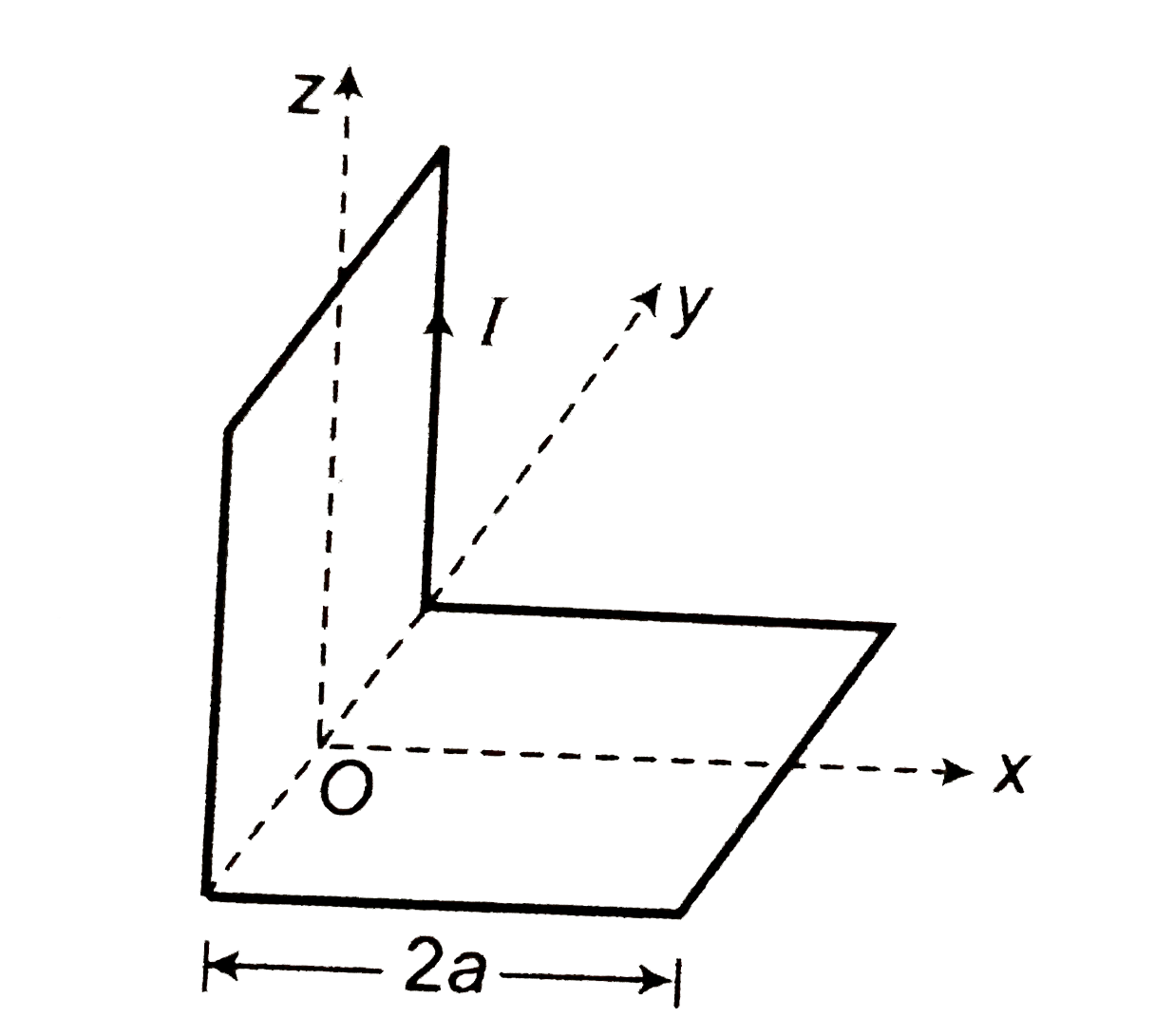
View SolutionTwo infinitely long wires each carrying current I along the same direction are made into the geometry as shown in the figure. The magnetic field at the point P is

View Solution
