A particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation of the particle as it comes out of the magnetic field if the width d of the region is slightly less than.
(a)mvqB (b)mv2qB (c)2mvqB

The radius of path r=mvqB
For d<r, we have sin θ=dr
(a)d=(mvqB)=r,∴sinθ=(mvqB)(mvqB)=1orθ=π2 rad
(b)d=(mv2qB)<r,sinθ=dr=mv2qBmvqB=12orθ=π6rad
(c)d=(2mvqB)>r, the deviation of particle is therefore θ=π rad.
Topper's Solved these Questions
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS )
18 Videos
- ELECTROMAGNETICSBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETICSEXERCISE - PROBLEMS (LEVEL -I)
30 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
20 Videos
- ELECTROSTATICSBOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
4 Videos
Similar Questions
A particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation of the particle as it comes out of the magnetic field if ihe width d of the region is
2mvqB

View SolutionA particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation fo the particle as it comes out of the magnetic field if the width d of the regions is very smaller then

View Solution
Knowledge Check
A particle having mass m, charge q enters a cylindrical region having uniform magnetic field B in the inward direction as shown. If the particle is deviated by 60∘ as it emerges out of the field then what is the time spent by it in the field?
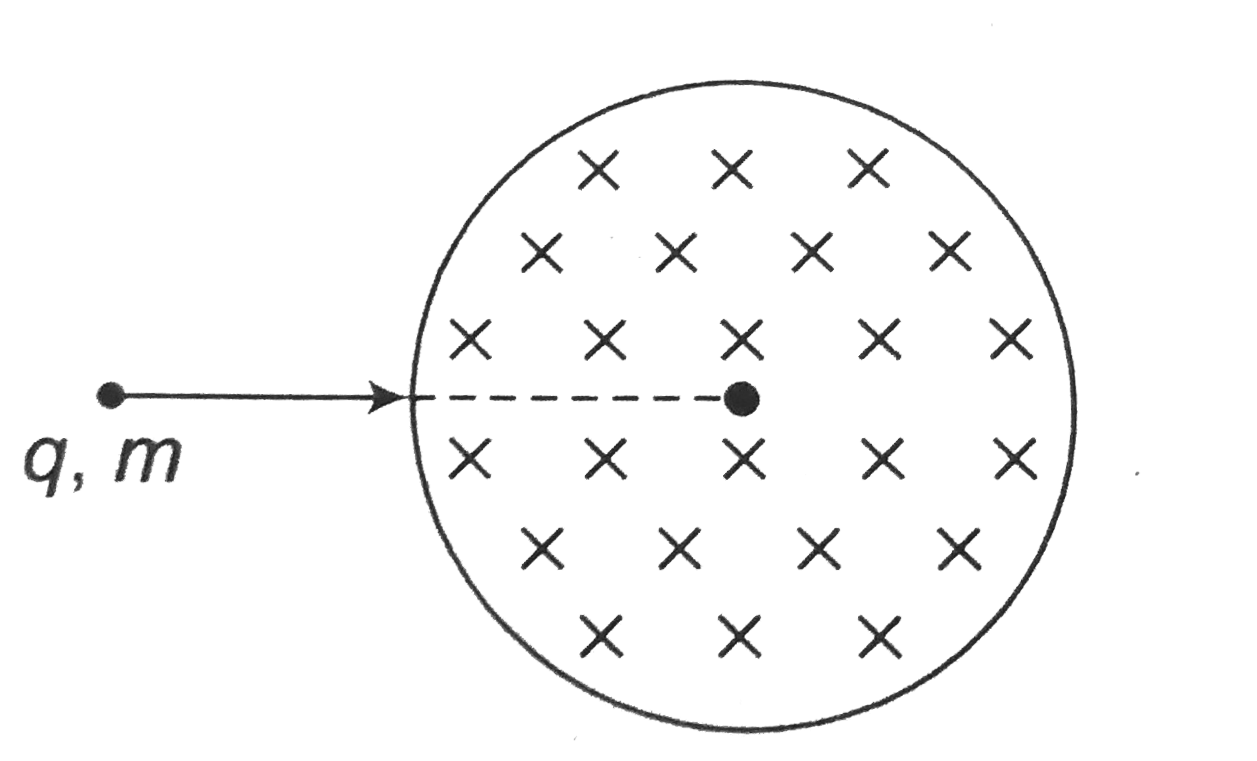 A2πmqBB2πm3qBCπm3qBDIt depends on the speed of particle.
A2πmqBB2πm3qBCπm3qBDIt depends on the speed of particle.A charged particle of mass m and charge q is projected into a uniform magnetic field of induciton →B with speed v which is perpendicular to →B. The width of the magnetic field is d. The impulse imparted to the particle by the field is (d<<mv/qB)
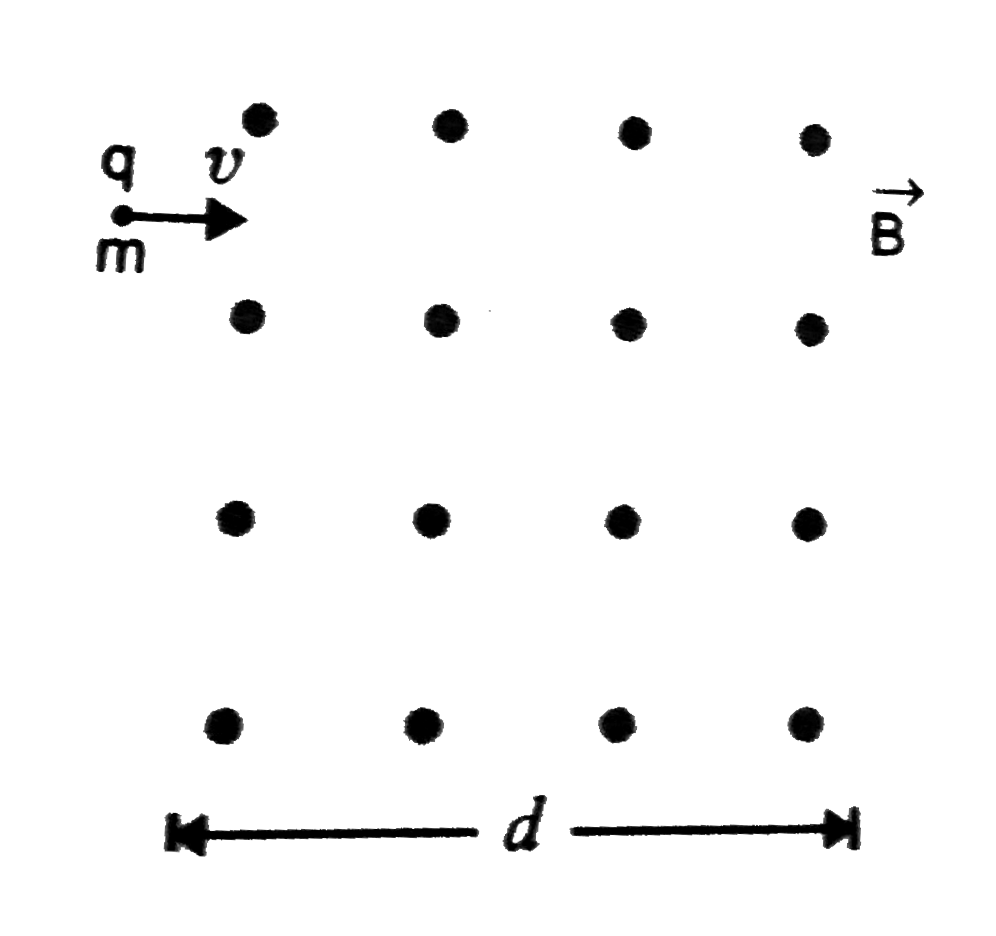 AqBdBqBv/dCmv/(qBd)Dmv2/(qBd)
AqBdBqBv/dCmv/(qBd)Dmv2/(qBd)
A particle of mass m and charge q is projected into a region having a perpendicular magnetic field B. Find the angle of deviation fo the particle as it comes out of the magnetic field if the width d of the regions is very smaller then

View SolutionA particle of mass m and charge q is projected into a region having a perpendicular magnetic, field B. Find the angle of deviation of the particle as it comes out of the magnetic field if the, width of the region is b, which is very slightly less than mv2Bq
View SolutionA particle of mass m and charge q is projected into a region having a perpendicular magnetic, field B. Find the angle of deviation of the particle as it comes out of the magnetic field if th,, width of the region is (b)
(a) 2mvBq(b)(mv)/(Bq)(c)(mv)/(2 Bq)
View SolutionA charged particle (q,m) enters perpendicular in a uniform magnetic field B and comes out field as shown. Find the angle of deviation θ for particle to cross magnetic field will be
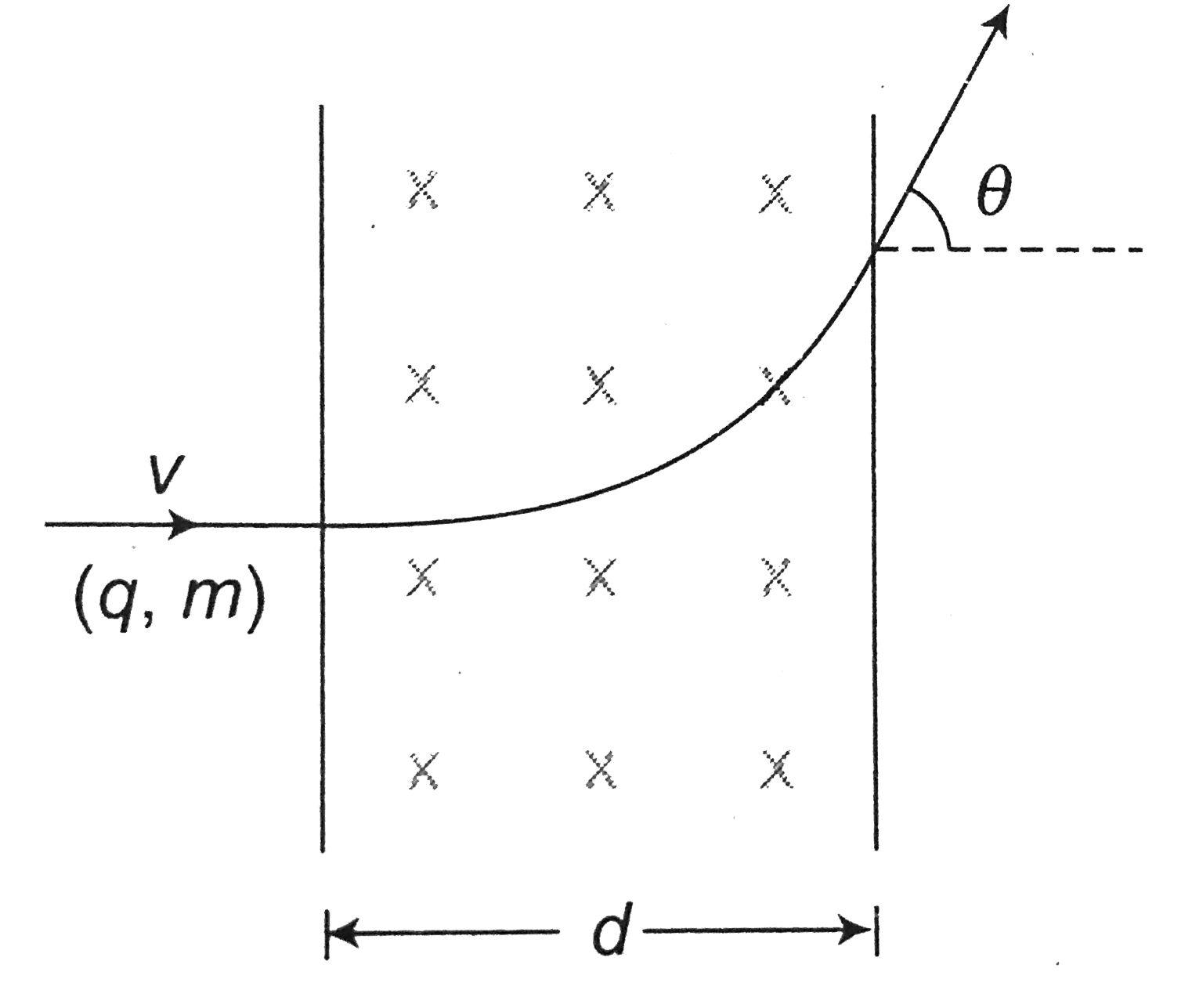
View SolutionA charged particle of mass m and charge q is projected into a uniform magnetic field of induciton →B with speed v which is perpendicular to →B. The width of the magnetic field is d. The impulse imparted to the particle by the field is (d<<mv/qB)

View SolutionA charge particle of mass m and charge q enters in a region of uniform magnetic field as shown in figure.The magnitude of change in linear momentum due to magnetic field will be
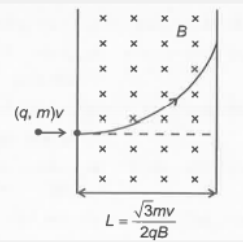
View Solution
